How Reversed-Phase Liquid Chromatography Works

The keys to understanding reversed-phase liquid chromatography (LC) are provided at the molecular mechanism level as determined by high accuracy molecular simulation. The essential features of C₁₈ stationary-phase chains in contact with methanol–water and acetonitrile–water mixtures are discussed in the context of bonded-chain geometry, spatial distribution of alkane and alcohol solutes, retention mechanism, and retention thermodynamics. This tutorial is intended to be applicable to a wide audience ranging from occasional users of liquid chromatography to separation scientists.

The technique referred to as reversed-phase liquid chromatography (LC) is the workhorse of all LC techniques. Continued improvements in columns, instrumentation, and application methodologies have led to reversed-phase LC being used in more laboratories and delivering results faster, with higher resolution. We confine the scope of our discussion of reversed-phase LC to column materials that contain a bonded-phase layer of a hydrophobic material, usually dimethyl octadecylsilane (C₁₈), bound to a porous silica support. The C₁₈ chains function as the retentive material, yet details of chain conformation, how the solutes of interest interact with the chains, where the solvent lies in proximity to the chains, and the thermodynamics of this process have been lacking for many years. It is ironic that with the high popularity of this technique, the details of the retention mechanism have been so difficult to obtain. The purpose of this tutorial is to enlighten readers about the detailed inner workings of reversed-phase LC.

For many years researchers believed they could figure out the mechanism of reversed-phase LC by examining the pattern of retention times produced by injecting different but chemically related solutes into columns with different stationary-phase chemistries using a range of mobile-phase compositions. This is a fallacy because retention time experiments cannot give a microscopic picture of the bonded-phase chains and inference of the structure of these chains is largely circumstantial. The retention time experiment is thermodynamic in nature — it is determined by the distribution of the solute between the mobile-phase solvent system and the stationary phase. This distribution is the subject of what is often referred to as "phase equilibria." No microscopic mechanism can be gleaned from data of this type (that is, thermodynamic data). In essence, thermodynamics is just a bookkeeping system, nothing more and nothing less.

Spectroscopic investigations have also been inconclusive and have not revealed details of the retention mechanism. This is because spectroscopic techniques like nuclear magnetic resonance (NMR), infrared (IR), and fluorescence spectroscopy detect bonded-phase chain conformations and solute associations for a large number of molecules simultaneously; these conformations and interactions are complex, the signals are difficult to decompose into single molecule information, and widely diverse signals lead to spectroscopic broadening and loss of resolution. Hence, reversed-phase LC retention mechanisms have not been revealed by these techniques. Some spectroscopic techniques have given outstanding structural information, for example, determining the distribution and types of silanol groups on silica and revealing the silica bonding details of derivatizing agents by solid-state NMR spectroscopy (1).

We have learned a great deal about the reversed-phase LC system through the use of high-accuracy molecular simulations (2–4). Simulation offers a unique methodology to understand disordered and microheterogeneous systems and this has been the primary technique used for many years in the study of liquids (5,6). Simulation is necessary because of the complexity from the large number of simultaneous interactions that take place (the so-called "n-body problem" of liquids). These types of problems cannot be understood or formulated mathematically unless drastic simplifications are made. These simplifications render such theories of limited use. With the advent of high-speed computers and advances in simulation methodology, this method of investigation has become more practical and has now evolved to the point where simulation can reproduce the energetics of reversed-phase LC accurately. Since simulation gives mechanical details of reversed-phase LC on an atomic scale (that is, chain conformation, solute and solvent locations) the mechanism of the reversed-phase LC process can be revealed using simulation methodology. As we will show, the comparison of simulation with experiment is impressive and provides an assurance that these computational results for model systems carry a sufficient amount of realism to learn about real chromatographic systems.

In this tutorial we will guide readers through a number of our simulation findings that give the microscopic details needed to understand reversed-phase LC. This is not only useful for chromatographers in understanding practical separations, but it may prove useful toward designing new stationary phases for liquid-based separations other than reversed-phase LC. A detailed description of the simulation methodology, the bonding procedure, and further details are given in two review articles (3,4). We refer readers to these references and others given here for the detailed workings of this methodology.

Structure of the Bonded-Phase Chains and Solvent Location

The starting point for discussing reversed-phase LC is the chain conformation. This point has been contentious because of the observation that when reversed-phase LC columns are run in 100% aqueous solvent and subsequently depressurized, retention is diminished drastically and this has been thought to be caused by chains collapsing and lying flat on the surface (7). Alternatively, this effect has been described by Walter and colleagues (8) as a dewetting process. Partial dewetting has been confirmed by molecular simulation (9), in which it was shown that the chains do not collapse on the surface when run under pure water conditions. We have found, in fact, that the probability of collapse of octadecane molecules in pure water is very small and the solvent composition does little to affect the conformation of C₁₈ chains (10).

The density profiles of C₁₈ chains bound to silica are shown graphically in Figure 1, for seven solvent systems. These are labeled as pure water (W), pure methanol (M), pure acetonitrile (A), and the systems 33% methanol in water (33M), 67% methanol in water (67M), 33% acetonitrile in water (33A), and 67% acetonitrile in water (67A). In the four cases of the mixed solvent systems, these percentages are given as the mole percent of the organic modifier, as opposed to the usual volume-to-volume designation. We prefer mole percent or the mole fraction because it is independent of temperature and pressure. For these simulations at 323 K, the 33M solvent is 53% methanol by volume. The 67M is 82% methanol by volume, the 33A solvent is 60% acetonitrile by volume, and the 67A solvent is 87% acetonitrile by volume.

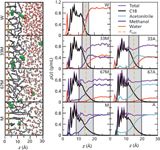
Figure 1: Left: Snapshots from simulations in different solvents, C18 chains (gray), and alkane and alcohol solutes (large spheres with green, red, and white indicating CHx, hydroxyl oxygen, and hydrogen, respectively). Middle and right: Density profiles of C18 chains, methanol, water, and acetonitrile for pure water (W), pure methanol (M), pure acetonitrile (A), and solvent mixtures given as mole percent. T = 323 K, grafting density of 2.9 µmol/m2. Adapted, in part, from references 4 and 14.

On the left in Figure 1, snapshots of C₁₈ chains are shown for various methanol concentrations. The results for acetonitrile are similar. The independent variable z shows the distance in angstroms from the site of chain attachment at the outermost silicon atom of the silica surface (at z = 0 Å) and perpendicular to the surface. We will use this distance repeatedly throughout this tutorial. In all of these cases, the chain conformation is highly disordered but with a preference to stand roughly perpendicular to the surface, as will be shown in the following figures. For the model systems shown here the silica support is assumed to be a planar substrate; simulations for a cylindrical pore yield behavior in good qualitative agreement with the planar substrate (11).

As shown on the right of Figure 1, the solvent penetration into the bonded-phase region is not extensive. Water and methanol are found hydrogen-bonded to free silanol groups at the silica surface (peaks at z = 3 and 4 Å for water and methanol, respectively). From both of the snapshots on the left and from the seven average density graphs, it is shown that very little water is found where the carbon density of the C₁₈ chains is high. For the pure water simulation (W), it appears that the water does not reach its liquid density until ≈3 Å (that is, about one molecular diameter) away from the ends of the C₁₈ chains. Looking at the red curves (the water density) and the black curves (the C₁₈ chain density) it can be seen for all of the solvent systems where water is present that there is a small distance between the chain ends and the z value where bulk water density is established. This space is caused by the lack of hydrogen bonding capability when water is near a hydrophobic interface. This space represents a low water density region characteristic of the lack of surface wetting. In all cases shown in Figure 1, the penetration of organic modifier into the chain region increases as the solvent concentration of organic modifier increases. At low organic modifier concentrations, (33M and 33A) there is a significant enrichment in the organic modifier concentration at the interface between bulk solvent and the chain region. We will address this effect in more detail below, noting this excess has been measured experimentally (12,13).

In Figure 1, the gray zone indicates the width of the chain-solvent interface that covers 80% (from 10% to 90%) of the change in solvent density. The orange dotted line is the Gibbs dividing surface (GDS), a plane that represents the location of the chain-solvent interface where the solvent density is half-way between the bulk and interior solvent density, as determined by an interpolation procedure we have used extensively (3,4). The GDS, as used here, represents a simple (but artificial) plane defining the border between mobile and stationary phases.

As can be seen from the graphs in Figure 1, the location of the GDS depends only weakly on solvent composition, although the GDS is slightly closer to the silica surface under pure methanol composition. The width of the chain-solvent interface is larger as the organic modifier concentration increases. This is slightly more pronounced for methanol than for acetonitrile. Overall, this behavior shows that the organic modifier concentration is more diffuse within the chain-solution interfacial region as the organic modifier concentration is increased.

The chain conformation, as implied from these density plots, shows rather subtle effects from changes in the solvent environment. This is shown in Figure 1 in which the density of the C₁₈ chains, as a function of distance from the silica surface, extends further from the silica surface with increasing organic modifier concentration. This suggests that the chains stretch or swell with higher modifier concentration although the effect is subtle.

Overall, it appears from Figure 1 that very little solvent is present in the interior of the chain system for highly aqueous mobile phases and there are simply no solvent molecules to displace the solute. The solute, therefore, has little to no competition with the solvent for interaction sites within the chain interior region.

Chain Coverage, Tilt, and End-to-End Distance

Many researchers have speculated that the chains are folded on the surface of the support material and others have envisioned the bonded chains to stick out from the surface. As shown previously through simulation (15), the chain geometry is dependent on surface coverage as illustrated in Figure 2. For relatively low coverage, the chains can lean over but are generally oriented away from the surface. As coverage increases, the chain extension is driven by excluded volume and the chain backbone, for parts close to the surface, orients approximately perpendicular to the surface. At all surface coverages, the chain ends show a relatively random arrangement.

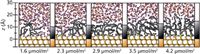
Figure 2: Snapshots illustrating the structural effects of grafting density. The stationary phase is depicted as tubes with CHx groups in gray, hydrogen in white, oxygen in orange, and silicon in yellow. Solvent molecules are shown in the ball and stick representation with oxygen in red, CH3 groups in blue, and hydrogen in white. The silicon substrate atoms define the position z = 0 Å. The average end-to-end distance of the chains, as a function of coverage, is shown in gray on the side of each molecular representation as a level plot. The methanol mole fraction is 0.5 (0.69 v:v methanol:water) and T = 323 K. Adapted from reference 15.

At medium coverage, the C₁₈ chains at carbon numbers above about half of the chain length orient rather randomly. However, at the highest coverage shown in Figure 2, the chains are more oriented toward an approximately perpendicular configuration.

The average end-to-end distances (the distance from the first carbon to the last carbon in the C₁₈ chain) for these surface coverages are 10.2 Å, 11.6 Å, 11.6 Å, 12.6 Å, and 14.9 Å, respectively. These results indicate that the chain orientation and average end-to-end distance are highly dependent on coverage. These numbers compare favorably with the value of 17 ± 3 Å obtained by neutron scattering (16) in pure methanol and with C₁₈ that was bonded to silica under maximum coverage conditions (16).

Notice in Figure 2 that at the lowest coverage the solvent has much easier access to the surface silanols than at the highest chain coverage. In all cases, a gap between the solvent and the chains exists. This is a consequence of the limited solubility of water and the organic modifier in the C₁₈ chains. This interfacial dewetting behavior is rather universal where hydrophobic chains meet hydrophilic liquids.

As mentioned previously, for smaller organic modifier concentrations, an excess of the organic modifier is found in the interfacial region between bulk solvent and the C₁₈ chains. This is illustrated in Figure 3 where the excess in the relative modifier concentration is reached progressively farther away from the silica surface for higher chain densities. For the highest chain density, almost twice the concentration of methanol is obtained in the chain region as compared to the bulk solvent composition.

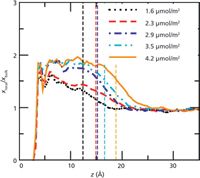
Figure 3: Local methanol mole fraction (xlocal) relative to the bulk solvent mole fraction (xbulk), as a function of the distance from the silica substrate. This graph shows the enhanced methanol concentration within the chain region for different surface coverages. Conditions are the same as for data in Figure 2. The dashed vertical lines are the GDS. The methanol mole fraction is 0.5 (69% methanol by volume) and T = 323 K. Adapted from reference 15.

Solute Molecule Spatial Location

One of the many questions that have been asked about reversed-phase LC is whether solute molecules adsorb on or partition into the chain system. In Figure 4 we show the distance-dependent distribution coefficient, K(z), for n-butane and 1-propanol solutes in seven solvent systems. K(z) is the ratio of the average number density of solute molecules in the stationary phase to the average number density of solute molecules in the mobile phase. Higher K(z)values indicate regions of higher solute retention. Averages are important here because this number varies as molecules move between the mobile and stationary phase. The simulation technique we use (3,4) to compute these results gives these numbers directly.

Figure 4: Distribution coefficient profiles, K(z), for n-butane (left) and 1-propanol (right) solutes in various mobile phase solvents. The stationary phase consists of C18 chains with surface coverage of 2.9 µmol/m2 and T = 323 K. The dashed orange lines are the GDS and the shaded gray regions give the 10–90% liquid region. Adapted from reference 14.

As can be seen from Figure 4, there are two maxima for K(z) of n-butane for all of the solvent systems investigated here. These maxima occur within the chain interior (z ≈ 7.5 Å) and below the GDS. We use n-butane as a prototypical hydrophobic alkane solute with no hydrophilic groups and 1-propanol as a model solute with a hydrophilic group (the alcohol group) and some hydrophobic character.

It appears that n-butane exhibits both a partitioning behavior (the first K[z]peak at 7.5 Å) and an adsorption behavior where the solute lies near the chain-solution interface. The orientation of the molecules can be determined using metrics such as the orientational order parameter (3,4) which shows that the n-butane solute within the chain interior region orients preferentially perpendicular to the silica surface, whereas the n-butane above the C₁₈ chains orients preferentially parallel to the surface in an adsorbed configuration near the chain-solution interface. Hence, hydrophobic solutes act with both partitioning (within the chains) and adsorption (on the chain's outer surface) retention mechanisms. As shown in Figure 4, higher organic modifier concentrations push the location of the adsorbed n-butane to higher z locations within the more diffuse interfacial region. The mean location of the partitioning n-butane peak increases only slightly with increasing organic modifier concentration.

The preferred location of the 1-propanol solute clearly indicates adsorption at the solvent–C₁₈ interface for the solvent conditions of pure water and for small concentrations of the organic modifier. As the concentration of organic modifier is increased, the location of the 1-propanol solute becomes more diffuse and part of this is because of the increased penetration of the organic modifier into the chains. For the mixed solvent cases, the preferred location of the 1-propanol solute is shifted to larger z values, that is, toward the region where enrichment of the organic component is found at the chain–solvent interface.

For the pure acetonitrile solvent, significant amounts of 1-propanol are found near the substrate. This is because of direct hydrogen bonding of 1-propanol to unreacted silanols; the split into two peaks in Figure 4 reflects different orientations of the 1-propanol solute because of the solute being either the acceptor or donor in the hydrogen bond formed with a residual silanol and due to different extents of steric crowding from the surrounding methyl side chains of the C₁₈ ligands. The hydroxyl group on 1-propanol is a much more effective hydrogen bond former than acetonitrile, which is only a weak hydrogen bond acceptor. It should be noted that for pure methanol solvent, most of the available silanols form hydrogen bonds to the solvent and, hence, there is no peak near the substrate for 1-propanol.

The K(z) plots for a homologous series can be converted to an incremental free energy diagram, as shown in Figure 5 (2–4). The incremental free energy of a methylene group and a hydroxyl group are free energy metrics that facilitate easy comparison with experiment. This is because the phase ratio, the ratio of mobile-phase volume to stationary phase volume, is most difficult to accurately measure in an experiment but is not needed for comparison of incremental free energies.

As can be seen from Figure 5, the methylene groups exhibit the most favorable interactions within the C₁₈ interior region; that is, negative free energies indicate more "favorable" interactions than with the bulk solvent (and higher probability of occurrence). Incremental free energies of zero indicate locations that do not contribute to retention and free energies greater than zero (referred to as "unfavorable") show a depletion of solute groups and are less likely to occur. At z ≈ 12 Å, where adsorption occurs, the methylene groups are slightly less energetically retained and this is likely because of the higher solvent density which permeates the chains at the interfacial region.

Figure 5: Incremental retention free energies of the methylene and hydroxyl groups as a function of location for different solvent compositions. Conditions are T = 323 K, surface coverage of 2.9 µmol/m2. Energies are given in joules (1 kcal = 4.184 kJ). Adapted from references 2 and 14.

For the hydroxyl group, the incremental free energies are negative in two regions: the first at low z values where the solute hydroxyl group forms a hydrogen-bond either directly to surface silanols or to solvent molecules that are bound to the silanols. The second region (z ≈16 Å) is found only for pure water where the solute can form a hydrogen bond to the partially dewetted solvent. Note that the magnitude of the incremental free energies is much larger for hydroxyl groups that can form hydrogen-bonds than the solute methylene group that favors lipophilic interactions with C₁₈ chains. Also note that the interactions of hydroxyl groups with chain elements is quite thermodynamically unfavorable, as denoted by the large positive free energies in Figure 5. For C₁₈ stationary phases and those formed by longer ligands, both adsorption and partition play a role for nonpolar solutes, whereas adsorption is always the major mechanism for solute molecules with polar groups.

Influence of the Mobile-Phase Composition on Retention Thermodynamics

Few topics in reversed-phase LC have caused more confusion than the retention mechanism and the subsequent thermodynamic analysis. Early attempts at a quantitative description of reversed-phase LC retention, such as the solvophobic theory of Horváth and colleagues (17), failed for the same reason that a quantitative theory of liquids failed: The complexity of the liquid state prevents a simple description of liquid chromatography.

For the thermodynamic cycle used to analyze the retention mechanism, an ideal gas reference state is included that is assigned a free energy of transfer equal to zero because there are no intermolecular interactions in this state. This cycle is given schematically in Figure 6.

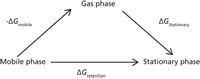
Figure 6: Schematic of the thermodynamic cycle used to decompose the contributions to retention.

The solute can be in any one of the three states: in the mobile phase, the stationary phase, or in the reference (gas phase) state. To measure the contribution of the solvent one considers moving the solute from the ideal gas reference state to the mobile phase. To measure the contribution of the C₁₈ chains, the surface silanols, and the embedded solvent, one considers moving the solute from the ideal gas reference state to the stationary phase. The retention process is then described by

This equation and the thermodynamic cycle indicate that the net free energy of solute retention, that is, moving a solute from the mobile phase to the stationary phase is the difference between the free energy of insertion from the gas phase to the mobile phase and the insertion from the gas phase into the stationary phase.

The complete thermodynamic cycle is shown in Figure 7 with free energies expressed as the incremental free energies of methylene and hydroxyl groups, as described and used above. We also include a bulk retentive phase of liquid n-hexadecane as a purely liquid–liquid partitioning process for comparison.

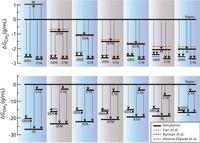
Figure 7: Computed and experimental incremental transfer free energies of a methylene group (top) and a hydroxyl group (bottom) for methanol-water and acetonitrile-water solvent mixtures. The retentive phases include dimethyl octadecyl silane (ODS or C18) bound to silica and bulk liquid n-hexadecane (C16). The C18 surface coverage is 2.9 µmol/m2, T = 323 K. The simulation data are from our laboratory and are described in a number of papers (2–4,18). The experimental data are taken from Carr and colleagues (19), Barman (20), and Alvarez-Zepeda (21).

Here is how to interpret the data illustrated in Figure 7. As discussed with Figure 5, any solute movement toward more negative free energies is referred to as a favorable transfer. However, as with any thermodynamic event, the time scale of transfer is not specified. In all of these cases, only the transfer of a methylene group from an ideal gas to pure water is unfavorable; free energy has to be expended to put a methylene group into water. In this one case the transfer is deemed solvophobic. In all other cases the methylene group prefers the mobile phase and the favorability increases from 33% methanol–water and 33% acetonitrile–water (all by mole fraction) to pure methanol and pure acetonitrile. These interactions are deemed solvophilic.

For all solvent compositions studied, lipophilic interactions of methylene groups with the C₁₈ (ODS) stationary phase drive the retention. This is indicated by the arrow going downward (showing the favorable nature of the interaction) that connects the solute group in the solvent phase to the solute group in the ODS phase.

Note that the free energy of transfer of a methylene group from the gas phase to the retentive phase does not vary much with mobile-phase composition. However, the decrease in retention as the organic modifier concentration is increased is driven by an increase in the solvophilic interactions with the solvent. Although the free energy transfer of a methylene group into ODS (that is, C₁₈) and C₁₆ are very similar in magnitude, this is somewhat coincidental because the mechanisms are different (4). Again, this highlights the danger of using thermodynamics to imply a retention mechanism.

For the transfer of a hydroxyl group into the mobile and stationary phases from the gas phase, it is found in Figure 7 that all transfers are favorable. In all cases, however, the transfer of the hydroxyl group from the mobile phase into the stationary phase is unfavorable (that is, the arrows in Figure 7 are upward facing). As shown in Figure 7, it is energetically more favorable to transfer a hydroxyl group into the mobile phase than the stationary phase. This point is echoed in Figure 5 where the incremental free energy, as a function of distance, shows that the free energy of a hydroxyl group is only favorable (that is, negative ΔG_OH) close to the substrate because of hydrogen bonding with silanol groups, whereas it is unfavorable for most of the C₁₈ chain region. The magnitudes of these free energies are such that addition of a hydroxyl group reduces retention by a larger amount than addition of a methylene group increases retention in C18.

As shown in Figure 7, simulation and experiment give very similar results. This is a tribute to the simulation methodology (2–4), which has evolved to be the leading simulation methodology for research in phase equilibria studies for some time (22). For any simulation to be believed it must agree with experiment. Simulation has enabled a profound, and in many cases surprising, picture into the inner-workings of reversed-phase LC that was not available by other methods.

We have studied other stationary phases and solutes than those discussed here, for example, embedded polar groups (23), studies with bare silica (24) used in hydrophilic interaction chromatography (HILIC), and retention of polycyclic aromatic hydrocarbons (PAH) (25) amongst others. In addition, we have studied the effects of pressure, chain length, and solute length (11) and a host of other variables pertinent to liquid chromatography. Other research groups have used molecular dynamics simulations to probe structure and dynamics of related chromatography systems including chiral stationary phases (26), adsorption of acridine orange at the reversed-phase LC chain-solvent interface (27), and solvent mobility at bare silica surfaces (28). One of the many lessons learned is that retention is highly dependent on the chemical nature of the solute and this makes reversed-phase LC both useful and fascinating at the same time.

Conclusions

The key insights for the reversed-phase LC system of C₁₈ chains bound to silica as determined by molecular simulation include:

Solvent

• Highly water-rich solvent mixtures form a nonwetting gap between the chains and the bulk fluid.

• Water, methanol, and, to a lesser extent, acetonitrile occupy the residual silanols.

• The organic component is enriched in the interfacial region between chains and the bulk fluid. This enrichment is dependent on organic modifier concentration (more enrichment at lower organic modifier) and chain density (higher enrichment at higher chain density).

Chain Conformation

• Chains are conformationally disordered with segments closer to the chain termini showing more disorder. With increasing surface coverage, the disorder is reduced and the chains align more perpendicular to the surface.

• Higher amounts of organic modifier in the solvent lead to swelling of the chains, but the effect is subtle.

Solute Position

• Alkane-like solutes can be found within the chain system (partitioning) and in the interfacial region of the chains (adsorption) for both acetonitrile–water and methanol–water mixtures. These solutes are found predominately perpendicular to the surface deep within the C₁₈ chains and lying preferentially parallel to the surface within the interfacial region.

• Solutes with hydroxyl groups (such as alcohols) are preferentially located closer to the interface of the C₁₈ chains and the solvent. This allows for enhanced hydrogen bonding to occur with solvent systems containing water.

Retention Thermodynamics

• For alkanes, the driving force for retention is lipophilic. Solvophobic interactions are only pertinent for solvent systems with very high water concentration. However, the decrease in retention upon increase in the organic modifier concentration is mostly because of an increase of the solvophilic interactions.

• For hydroxyl groups, the strongest interaction is solvophilic, that is, it is more favorable for a hydroxyl group to be in the solvent region than in the stationary phase. The difference in the favorable interactions with the solvent and the stationary phase decreases with increase of the organic modifier concentration.

• The magnitude of the hydroxyl incremental free energy is much larger than for the methylene group — it takes many methylene groups to balance the effect of one hydroxyl group.

We welcome any feedback from readers that will help us clarify, focus, and expand this work.

Acknowledgments

We are grateful to Pete Carr for many stimulating discussions and to Jack Kirkland for his helpful comments on this article. Financial support from the National Science Foundation (CHE-0718383 and CHE-1152998) is gratefully acknowledged. Part of the computer resources were provided by the Minnesota Supercomputing Institute.

References

(1) D.W. Sindorf and G.E. Maciel, J. Am. Chem. Soc. 105, 3767–3776 (1983).

(2) J.L. Rafferty, L. Zhang, J.I. Siepmann, and M.R. Schure, Anal. Chem. 79, 6551–6558 (2007).

(3) J.L. Rafferty, J.I. Siepmann, and M.R. Schure, Adv. in Chromatography, E. Grushka and N. Grinberg, Eds. (CRC Press, Boca Raton, Florida, Vol. 48, 2009), pp. 1–53.

(4) R.K. Lindsey, J.L. Rafferty, B.L. Eggimann, J.I. Siepmann, and M.R. Schure, J. Chromatogr. A 1287, 60–82 (2013).

(5) M.P. Allen and D.J. Tildesley, Molecular Simulation of Liquids (Oxford University Press, Oxford, England, 1989).

(6) D. Frenkel and B. Smit, Understanding Molecular Simulation, 2nd Ed. (Academic Press, New York, 2001).

(7) M. Przybyciel and R.E. Majors, LCGC North Am. 20(6), 516–523 (2002).

(8) T.H. Walter, P. Iraneta, and M. Capparella, J. Chromatogr. A 1075, 177–183 (2005).

(9) L. Zhang, L. Sun, J.I. Siepmann, and M.R. Schure, J. Chromatogr. A 1079, 127–135 (2005).

(10) L. Sun, J.I. Siepmann, and M.R. Schure, J. Phys. Chem. B 110, 10519–10525 (2006).

(11) J.L. Rafferty, J.I. Siepmann, and M.R. Schure, J. Chromatogr. A 1216, 2320–2331 (2009).

(12) D. Westerlund and A. Theodorsen, J. Chromatogr. 144, 27–37 (1977).

(13) F. Gritti, Y.V. Kazakevich, and G. Guiochon, J. Chromatogr. A 1169, 111–124 (2007).

(14) J.L. Rafferty, J.I. Siepmann, and M.R. Schure, J. Chromatogr. A 1218, 2203–2213 (2011).

(15) J.L. Rafferty, J.I. Siepmann, and M.R. Schure, J. Chromatogr. A 1204, 11–19 (2008).

(16) L.C. Sander, C.J. Glinka, and S.A. Wise, Anal. Chem. 62, 1099–1101 (1990).

(17) C. Horváth, W. Melander, and I. Molnár, J. Chromatogr. 125, 129–156 (1976).

(18) J.L. Rafferty, L. Sun, J.I. Siepmann, and M.R. Schure, Fluid Phase Equilib. 290, 25–35 (2010).

(19) R.P.J. Ratatunga and P.W. Carr, Anal. Chem. 72, 5679–5692 (2000).

(20) B.N. Barman, "A Thermodynamic Investigation of Retention and Selectivity in Reversed-Phase Liquid Chromatographic Systems," PhD thesis, Georgetown University, 1985.

(21) A. Alvarez-Zapeda, "A Thermodynamic Study of Acetonitrile–Water Mixtures in Reversed-Phase Liquid Chromatography," PhD thesis, Georgetown University, 1991.

(22) J.I. Siepmann, S. Karaborni, and B. Smit, Nature 365, 330–332 (1993).

(23) J.L. Rafferty, J.I. Siepmann, and M.R. Schure, Anal. Chem. 80, 6214–6221 (2008).

(24) J.L. Rafferty, J.I. Siepmann, and M.R. Schure, J. Chromatogr. A 1223, 24–34 (2012).

(25) J.L. Rafferty, J.I. Siepmann, and M.R. Schure, J. Chromatogr. A 1218, 9183–9193 (2011).

(26) R. Arjumand, I.I. Ebralidze, M. Ashtari, J. Stryuk, and N.M. Cann, J. Phys. Chem C 117, 4131–4140 (2013).

(27) A. Fouqueau, M. Meuwly, and R.J. Bemish, J. Phys. Chem. B 111, 10208–10216 (2007).

(28) S.M. Melnikov, A. Hötzel, A. Seidel-Morgenstern, and U. Tallarek, J. Phys. Chem. C 117, 6620–6631 (2013).

Mark R. Schure is with Superon and the Theoretical Separation Science Laboratory at Kroungold Analytical, Inc., in Blue Bell, Pennsylvania.

Jake L. Rafferty was a graduate student and postdoctoral researcher with the Department of Chemistry and Chemical Theory Center at the University of Minnesota in Minneapolis, Minnesota, and is with the Department of Chemistry at North Hennepin Community College in Brooklyn Park, Minnesota.

Ling Zhang was a graduate student with the Department of Chemistry and Chemical Theory Center at the University of Minnesota.

J. Ilja Siepmann is with the Department of Chemistry and Chemical Theory Center and the Department of Chemical Engineering and Materials Science at the University of Minnesota.

Direct correspondence to: mark.schure@gmail.com