Understanding Gradient HPLC

Isocratic high performance liquid chromatography (HPLC) for reversed-phase separations, while being both convenient and robust in its nature, has inherent issues, especially when dealing with compounds whose polarity (hydrophobicity) vary widely. Some of the generally accepted problems with isocratic separations include poor resolution of early eluted peaks, increased peak width for later eluted analytes because of peak dispersion, and inability to cope effectively with analytes having a broad range of hydrophobicity (LogP or LogD), which often results in unnecessarily long analyses and contamination of columns by strongly retained components.

Gradient HPLC can help to overcome some of these issues, typically providing better peak shape and the ability to elute analytes with a wide range of hydrophobicity values in a reasonable time frame. Gradients in reversed-phase HPLC usually involve the on-line (dynamic) mixing of solvents to achieve a steady increase in the organic solvent (typically methanol or acetonitrile) over the course of the analysis, which serves to increase the elution strength of the eluent over time.

Three essential parameters are required to specify a gradient in reversed-phase HPLC: initial %B, final %B, and gradient time (t_G) over which the transition in eluotropic strength will be achieved. The "big" questions in gradient HPLC are how to decide upon the optimum values for these three parameters, and it is usual to begin method development with a "scouting gradient" that increases the eluotropic strength from a low value to high value over a set period — typically 5–95% B over 10 or 20 min. The analyte elution behavior during this scan can then be used to assess the optimum operating conditions.

Before considering this in more detail, it's useful to draw a quick but effective analogy between isocratic and gradient HPLC.

In isocratic HPLC, the analytes enter the HPLC column and, depending on the partition coefficient of the analyte between the mobile- and stationary-phase material (governed by many factors including the hydrophobicity of the analyte and its shape), moves at a constant pace along the column undergoing successive partitioning events into the stationary phase, which controls analyte retention. Retention in isocratic HPLC is measured using the retention factor (k). In gradient HPLC, things are somewhat different.

At the beginning of the analysis, when the mobile-phase strength is low, the analyte will be partitioned wholly into the stationary phase ("focused") at the head of the column and will not be moving through the column at all. As the mobile-phase strength increases, the analyte will begin to partition into the mobile phase and move along the column. As the mobile-phase strength increases continuously, the rate at which the analyte moves along the column subsequently increases and the analyte "accelerates" through the column.

At some point within the column, the analyte may be wholly partitioned into the mobile phase, and will be moving with the same linear velocity as the mobile phase. The point at which this occurs depends on the nature of the analyte and its interaction with the stationary phase material. As the rate at which the analyte elution changes during gradient HPLC, k, the retention factor used above for isocratic separations is not applicable. Instead we use an "average" retention value, k*, or the retention factor of the analyte as it passes the midpoint of the column. Most analytes will be moving at the same rate as they pass the midpoint of the column and hence the gradient (average) retention factors for all analytes in gradient HPLC are very similar.

From the results of the gradient scan mentioned above, one can estimate from the retention times of the initial and final peak within the chromatogram the initial and final percentage of organic modifier (%B) required. It should be noted that when calculating these values the system dwell volume (or dwell time) needs to be considered. This is outside the scope of this short installment; for full details see the link to the CHROM-academy tutorial at the end of the text.

The remaining critical parameter is the gradient time. This is typically estimated using equation 1:

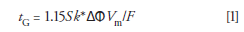
where gradient time (t_G) is in minutes, S is a shape factor and a value of 4 is typically used where the accurate value is not known, k* is the average retention factor for which a value of 5 is typically optimal, ΔΦ is the change in organic composition from the scouting gradient, V_m is the column volume (see equation 2) in milliliters, and F is the mobile-phase flow rate in milliliters per minute. So, for a scouting gradient of 5–95% B using a 150 mm × 4.6 mm column at a flow rate of 1 mL/min, the gradient time would be t_G = 1.15 × 4 × 5 × 0.9 × 1.5/1 = 31 min

All gradient analyses require a reequilibration time to reset the column conditions to the initial %B value ready for the sample injection; this is typically calculated as a multiple of the internal volume of the column, with a multiple of 10× commonly recommended by column manufacturers. A reasonable estimate of column internal volume can be achieved using equation 2:

The column volume (V_m) is in microliters and column length (L) and diameter (d_c) are both in millimeters. You should get a value of around 1.5 mL for a 150 mm × 4.6 mm column, which would mean a reequilibration time of 15 min with an eluent flow rate of 1 mL/min.