A Question of Balance? Part 2: Putting Principles into Practice

In the first part of this article on analytical balances we spent some time looking at laboratory weighing from the perspective of the basic principles and common practices.¹ Weighing is such a critical operation in all chromatography laboratories that we are amazed that there are so few references available outside analytical text books. For example, the European Pharmacopoeia (EP)² doesn't have a monograph on weighing and it is also not mentioned in ISO 17025 for testing and calibration laboratories.³ In contrast, the United States Pharmacopeia (USP) does.⁴ In fact, it has two: General Chapters <1251> and <41>. Chapter <41> will be discussed later in this article but remember that compliance with this is a legal US requirement, whereas <1251> is only guidance, albeit very strong guidance! Ignore it at your peril.

The most surprising fact to us about USP <1251>, which involves weighing on an analytical balance, is that it needed to be written at all! However, anyone who graduated in analytical chemistry in the last 20 years or so probably wasn't trained in the same classical gravimetric and volumetric analysis disciplines as the present authors were (Well, at least Chris was! Bob spent most of his time dissecting rotting livers as far as we can tell). Chapter <1251> covers some of the aspects we discussed in Part 1 and also good weighing practices, which will be discussed later. This chapter omits to mention buoyancy and its impact on the accuracy of weighing. However, before we get on to that topic we need to look at selecting the correct analytical balance to meet our basic weighing accuracy needs.

The Right Balance for The Right Job

You would never weigh an analytical reference standard on an ordinary top pan balance that you use for weighing substances for preparing buffers and mobile phases, would you? Of course not, an analytical balance would always be used but what type? Take, for example, a common 4-place modern analytical balance that has a capacity of 160–200 g and a displayed resolution of 0.1 mg. OK, so what is the smallest sample weight that can be weighed on such a balance: 100 mg, 10 mg, 1 mg?

Like all good philosophical questions, it depends... The explanation comes from the USP General Chapter <41>, which specifies that the minimum weight (the minimum permissible load) for a balance is that which will have an error less than 0.10% of that weight. [Pedantically, this error is more correctly defined as the expanded relative uncertainty with a coverage factor of 3 — but like politicians we won't let the facts get in the way].

Those of you chromatographers who do not work in laboratories that use the USP may wonder why you should bother reading this section. However, the principles outlined in this general chapter of the USP are applicable in any laboratory that needs to weigh analytical reference material with any degree of accuracy. Read, absorb and apply as appropriate — it makes good scientific sense.

OK, back to the analytical science, the minimum weight, m_min', that can be weighed on an analytical balance, is simply found from Equation 1.

The value for S_RP', which is the standard deviation for n replicate weighings (n ≥ 10), X_i' under actual conditions of use, and is easily calculated from Equation 2.

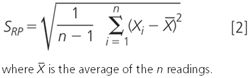
Note that to determine the value of S_RP accurately, you'll need a minimum of 10 and preferably more individual weighings. The importance of this is at the heart of the work we do in chromatography — get this wrong and the rest of our work doesn't make sense. So we don't work out standard deviations on 2, 3 or 4 weighings that are statistically poor practice or in the case of 2, nonsense. If you don't have a sufficiently large number of individual values for n then you don't get a good estimate of the uncertainties of the balance. A small amount of additional work here will repay itself many times over and underpin all of our critical work.

So, how many times are you going to determine S_RP? A baseline should be established when the balance is first installed perhaps? However, as the balance ages the SRP can change so you will need to decide how frequently you want to check it. For a balance that shows no problems perhaps once a year after the annual service would be sufficient. However, if the operational range of the balance changes, then this is a good time to recalculate S_RP. Good practice suggests that accuracy and precision be measured at the operating extremes of the balance (e.g., top and bottom rather than at the middle and then extrapolated to the extremes).

The problem with Equation 1 comes when the repeatability of the balance is so good that S_RP becomes zero. To get round this problem S_RP is set to 0.4 d where d is the readability of the balance. Hence we can set minimum weight limits for our balances based on the following two criteria.

You may wonder why the USP has felt it necessary to go into such detail for this legally binding requirement. Well, the sad fact is that some laboratories don't use common sense or sound science when weighing.

The police force for the USP is the Food and Drug Administration (FDA) who, when they find a laboratory deserving of note, write up their observations of non-compliance on FDA Form 483 (Investigational Observations). Here's an example from an FDA 483 showing how to get it wrong with an analytical balance:

"Reference standards are weighed on an analytical balance and weights are recorded to 4 decimal places (e.g., 0.0100 g). Typically 10 mg quantities are weighed out, however, in some instances smaller quantities are weighed."

Using the USP approach described above, let us suppose, perhaps optimistically, that this 4-place balance was so good that SRP was zero. The minimum weight that should have been weighed on the balance would be 0.12 g or 120 mg! No wonder the FDA got upset that the laboratory is weighing out typically less than 10% of what is required for reasonable weighing accuracy of their reference standards!

Not only is the very best performing 4-place balance not good enough to weigh 10 mg but neither is a best performing 5-place balance where m_min is 12 mg! This might come as a bit of a shock to some laboratories who want to use low standard weights to conserve expensive reference materials. If you want to weigh 2–10 mg quantities of reference substances you must use a 6-place microbalance. However...weighing on a microbalance is an art, not a science that needs stringent environmental controls and specialist operators who are regular users. This is for specialist laboratories only in our experience or put another way — don't try this at home.

By the way, the 483 comments above did include one instance where the weight of standard weighed out on the 4-place balance was 1.1 mg! Imagine the errors generated when this occurred.

So the bottom line is to ensure that you use an appropriate analytical balance and that you weigh sufficient material to reduce errors, especially of analytical reference materials that you will use in quantitative chromatographic analyses. The greater the number of decimal points could reduce the amount you weigh but if it is not checked then you won't know if it's fit for its purpose. If you have a large number of analytical results that are out of the expected range, one of the factors may be the weighings.

Buoyancy Correction

Imagine, that we have had our balance calibrated by a certified ISO Guide 17025 engineer with traceable E1 masses. Then the reading on the balance display will be correct then, won't it?

Do you want the good news or the bad news?

The good news is that the reading on the balance is correct (at least within the measurement uncertainty) but only if we weigh other masses of the same density. After all this is what we do to perform our periodic checks isn't it? The bad news is that we actually want to weigh samples in weighing containers (bottles, beakers or, heaven forbid, directly into volumetric flasks) whose density is typically less than that of the masses used to calibrate the balance. The use of volumetric flasks is not particularly a good idea for solid materials but more of that later.

What is the problem then? Well, the problem is buoyancy. Some readers who bath regularly (or even occasionally) may recall Archimedes and his famous overflowing principle about the loss in weight because of the up thrust (buoyancy) being equal to the mass of fluid (in our situation air) displaced.

In order to get rid of this small annoyance, we can perform all our weighings in a vacuum! However, this isn't terribly practical. Strictly speaking you can only have buoyancy when the fluid is subject to a gravitational field. An even more impractical solution would be to put our balance in an orbiting space craft but alas our sample would have no weight only mass! But, as they say, that is another story.⁶

The effect of the buoyancy is to make the measured sample mass — if it is less dense than the calibration masses — lighter than the actual mass so that the balance reading observed by you is lower than the actual mass of the object. In most instances this difference is small and we can ignore it, but not always. We can calculate the difference from Equation 4.

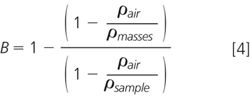
where B, buoyancy, is in g per g of sample weighed. From this equation (trust us — you know we are right), the buoyancy effect at a given temperature is dependent on three items:

• Density of the air, ρ_air

• Density of the calibration masses, ρ_masses

• Density of the sample being weighed, ρ_sample.

The relationships between these items are more easily explained using a graph where the density of the material being weighed is plotted against the buoyancy effect for masses with a density of 8.00 gcm^–3 — this is the density of the majority of reference masses we use. If you really want to be anally retentive, it is the density of austenitic stainless steel used to make the masses we use in a chromatography laboratory (this means that the metal used to manufacture the masses contains high levels of chromium and nickel and low carbon to ensure that they do not corrode easily otherwise they become useless pretty quickly). The lighter the density of material being weighed the greater the impact of buoyancy and the greater the difference between the balance reading and the actual mass of the object being weighed. However, when materials above the density of the weights are used, then the buoyancy effect is reversed and the balance reading is higher than the actual mass of the object.

In Figure 1, we did the calculation for sample densities between 0.4–10 gcm¯³ and expressed B as a percentage to make it more usable. Alternatively, we can calculate the buoyancy corrected true mass, M_t' from the observed mass, M_obs' from Equation 5.

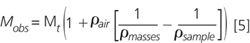
For any reader who has survived this far and is feeling an urge to learn more about air buoyancy correction in high accuracy weighing applications, we recommend that you read the Schoonover and Jones 1981 paper in Analytical Chemistry⁷ SOP 2 from the NIST website.⁸ As we said before, the effects are small but if you are making accurate standards with low-density materials you might need to do the correction. ''Ah!'' we hear you cry, but as we usually do all our weighings by difference doesn't this buoyancy effect get cancelled out. Well, it does for the tared container as they do cancel out but not the sample because of the density difference between it and the calibration masses.

The other assumption that we are making in Equation 4 is that the density of the air is constant, which it isn't! Temperature, water content (humidity), carbon dioxide level and pressure all affect the value. There is a wonderful equation for allowing for these effects but it is best left to the national laboratory metrologists.⁹ Just in case you didn't believe us, here it is!

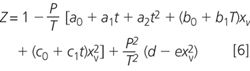
For the meaning of the symbols, additional constants and equations in 21 pages of mind numbing erudition see the NIST SOP 2.⁸

For those that can't be bothered, for practical intents and purposes an air density value of 0.0012 gcm¯³ is sufficient for the majority of chromatography laboratory purposes.

Figure 1

So what the sceptics amongst you may say is what does this mean in practice for us in the laboratory? Let's look at a simple example. Suppose for the sake of argument that we want to make a 5.0000% m/v standard solution of acetonitrile in water using a volumetric flask. You may remember we warned you not to weigh materials using this approach earlier but this can be done using weighing pipettes. Consider the following sequence of events:

• We weigh accurately about 5 g of acetonitrile carefully dispensed into a tared 100.0 mL Grade A volumetric flask on our qualified and calibrated 4-place analytical balance. Say we get a value of 4.9988 g. Well, we would, wouldn't we!

• If we then could make the solution up exactly to volume with water, making sure that any errors from degassing and temperature effects were eliminated, is the concentration value 4.9988% m/v? No it isn't, because we have failed to correct for the buoyancy of the sample and the density of the acetonitrile is 0.786 gcm^–3 not the 8.00 gcm¯³ of our calibration masses.

• Using our formula in Equation 4 we calculate that the buoyancy is about 20.14% (i.e., the true mass is approximately 5.0057 g, which is a difference of 6.9 mg). This isn't a lot and is insignificant for most laboratory applications but it a source of constant bias, which for high accuracy work should be eliminated if at all possible.

Gravimetric Preparation of Mobile Phases

How do you prepare mobile phase in your laboratory? Just take two measuring cylinders and measure the volumes of the aqueous and organic components and mix them in the bottle on the HPLC system? Well, another area of interest for accurate weighing, for practising chromatographers, is the control and preparation of mobile phase using gravimetric dilution. This is much more precise and more accurate than using the measuring cylinders above or other volumetric dispensing methods that are normally used in our laboratories.

Gravimetric dilution is particularly helpful where small changes in the mobile phase composition could lead to large changes in retention for example. If we wanted to dispense 500 mL of acetonitrile we would need to weigh 500 times the density of acetonitrile 0.786 gcm^–3 (i.e., 393 g). Using a standard two decimal place top pan balance we could reasonably expect to dispense this quantity to ± 10 g or better which is accurate to 0.03% which is very much better than we can do volumetrically (0.1% with Grade A glassware). However, the problem is that we need to know the acetonitrile density at control laboratory temperature say 20 ± 2 °C. This is easy for pure substances as we can find the values in standard reference works but what about buffer or other non-standard mixtures?

Suppose we make 250.0 mL of an aqueous buffer solution in a Grade A volumetric flask of which we know the dry weight and compensate for it by taring the balance. We then reweigh the flask on our top pan balance to two decimal places. We then calculate the density by dividing the solution weight by 250. This is illustrated in Figure 2.

Figure 2

Suppose our empty dry flask weighed 98.46 g and we tare it. The observed mass of the solution is 251.42 g. This gives an apparent density of 1.0057 gcm^–3. (The purists among you will probably prefer to determine density properly using density bottles or other standard techniques but this "quick and dirty" method is good enough for mobile phase preparation densities). Assuming that for buoyancy correction purposes the solution density is almost the same as water, 1.00, the buoyancy correction is 20.26 g, which is quite large. The true mass is then 251.68 g, which gives a density of 1.0067 gcm^–3. If you are going to do gravimetric dilution properly within the controlled chromatography laboratory you really should be buoyancy correcting your density measurements. (By the way, if you are ever tempted to calibrate your volumetric flasks then this is essential as the correction for a litre of water is about 1 g!)

The bottom line is that for the best work you need to consider buoyancy correction especially if you are weighing low density materials.

Good Weighing Practice

So what can really ruin a weighing operation? In this section we provide a checklist of issues that need to be considered when placing and setting up a balance as well as when operating one to weigh samples. More advice is given in USP <1251>.

Draughts: Many analytical balances are designed with doors to protect the weighing compartment from draughts. This is important as these can impact the accuracy and stability of a balance, especially when weighing small samples. For open pan balances, you can assess if draughts may adversely impact the operation by putting a box or other container around the instrument to assess if it will stabilize the weighing operation.

Temperature effects: If you want to really compromise your weighing operations, place any balance next to a window with direct sunlight or adjacent to a radiator or other heat source. The balance temperature will vary throughout the day making consistent operation impossible, especially when the instrument is designed to operate at a consistent temperature. In addition, heat sources will also induce air flow that will mean draughts that will also contribute to the inconsistency of operation.

Electrical and electromagnetic interference: Ideally analytical balances should be located in a separate location away from sources of electrical or electromagnetic influences. However, in many laboratories balances are located together with the rest of the analytical equipment, which may generate either electrical or electromagnetic interference. Furthermore, plant within a building may also generate this type of interference. Equipment that is known to produce electrical fields should either be turned off if practicable when the balance is used or the balance sited away from the equipment.

To minimize the impact of electromagnetic fields ensure that the balance is marked CE for compliance with the European Union Electromagnetic Compatibility (EMC) Directive.

Magnetic effects: You'll remember from Part 1 that many analytical balances use magnets in their operation. Therefore, if there is a magnetic field close to the balance there is a possibility of interaction and the balance operating incorrectly. Weighing in the NMR room next to the superconducting magnet is probably not the best weighting practice.

Flat surface: Accurate weighing requires that the balance is situated correctly. The surface where the balance is placed should be flat. For this reason all balance manufacturers incorporate a spirit level in the instrument so that the legs can be adjusted and the system operates in a level plane (what do you mean — you always wondered what the little bubble at the front was for?).

Vibration: This factor is also an important factor that must be eliminated or at least minimized. The influence of vibration will be seen with instability of a balance especially when measuring low masses. Traditionally, analytical balances were used on slate tables with brick pillars. The slate surface can be made very smooth and the overall design is excellent for eliminating vibration.

However, in many laboratories balances are placed on normal benches, vibration may need to be evaluated and perhaps an anti-vibration mounting rubber mat used to minimize the impact. Many modern balances have a variety of time constant adjustment settings to assist with less than ideal conditions.

Spilling liquids into the electronics is not a good idea as it tends to make the balance malfunction or at best lead to corrosion of delicate parts. Always clean up any spillages immediately and that applies to solids as well.

Static electricity: Glassware has a tendency to build up a static charge and hence can affect the balances accuracy and stability. Antistatic precautions are essential especially as the mass of sample decreases. Static discharges can cause the balance reading to drift and induce systematic errors. The more well known effect of static is the ability of finely powdered materials to spread themselves liberally around the balance case and onto the balance pan and not into the container you want it to be in.

Direct weighing of solids into volumetric flasks: We said earlier don't do this but if you need to then do it properly by difference using a weighing boat or weighing funnel. In this method you weigh the flask before and after dispensing as well as the weighing funnel. This method allows you a sanity check between the amount dispensed from the weighing funnel and the actual amount inside the flask. If there is a difference then you will know that there has been a spillage.

Good Calibration and Maintenance Practice

Your balance needs to be calibrated regularly throughout the operating range of the balance used by the chromatography laboratory. Typically, the supplier or an accredited agent will perform this on a regular basis but in between supplier calibration the balance must be checked regularly. Although there is the option of an internal check built in by the supplier or an external check using a mass of known value — checking the accuracy against external calibrated mass(es) is good practice.

However, the definition can regularly be open to interpretation:

• Once per use: The balance is calibrated before each sample and every weighing or weighing session. From this you know that the balance is within calibration and you can proceed without any apparent problems.

• Once per day: The first person to use the balance per day calibrated the balance and then all other users. Again, provided the idiot before you did not pour sulphuric acid into the balance you have a reasonable degree of confidence that the balance works on the day you used it.

• Once per week: At the beginning or the end of a week the balance is calibrated. Hmmm, well its only five days ago but the figures show that the balance worked. If the worst comes to the worst, we only have to repeat a maximum of five days work as the balance was out.

• Once per month: Close to the calibration there is less of a problem. The longer you go the greater the problem. Still, look on the bright side, it's only a maximum of 30 days of work in a worst case scenario that needs to be repeated if there is a problem.

It is better practice to do this on a daily or once per use basis rather than a weekly one as the influence of an out-of-calibration balance will be dramatic.

Use the internal calibration mechanism from the supplier to adjust the zero reading of the balance. Then a single calibration mass within the operating range should be used to check the correct functioning of the balance. If working within a regulated or ISO 17025 laboratory you'll have a procedure for this and keep records of the calibration checks over time. However, the process is applicable to all chromatography laboratories regardless of the quality system they use. In some laboratories, local laws may mandate that balances must be calibrated by an approved independent organization with certification.

Your procedure will also need to have a section on what to do if the balance falls outside of pre-defined acceptance limits. If this happens you'll not use the balance and call in the vendor or service agent to perform maintenance on the balance.

The biggest issue if your analytical balance is not maintained is can you really trust the work your laboratory produces? So in addition to the calibration, there should be a regular balance maintenance programme — typically this is combined with a calibration — that is performed by the vendor or an approved service agent.

Summary

An analytical balance is a critical element in the chromatography laboratory and needs to be controlled and subjected to TLC (tender loving care in this instance!). How much control is required is determined by you and your laboratory. How much is enough? This can be determined by a quotation from the world famous chromatographer, Clint Eastwood, "Do you feel lucky?" Or do you want to feel reasonably certain?

Chris Burgess is principal of Burgess Consultancy, specializing in the quantification and validation of analytical instrumentation and systems.

Bob McDowall is principal of McDowall Consulting, Bromley, Kent, UK. He is also a member of the Editorial Advisory Board of LCGC Europe.

References

1. C Burgess and R. D. McDowall, LCGC Eur., 17(7), 390–395(2004).

2. European Pharmacopoeia, 5th, EDQM, Strasbourg 2005.

3. ISO/IEC 17025:2005, General Requirements for the competence of testing and calibration laboratories, Geneva, Switzerland.

4. United States Pharmacopeia, 28th Edition, Rockville, Maryland, USA, 2005.

5. Forest/Inwood Laboratories Inc., 483 Observations, 23rd October 1998, Observation 11.

6. http://www.npl.co.uk/mass/faqs/buoyancy.html and http://www.npl.co.uk/mass/guidance/buoycornote.pdf

7. R M Schoonover and FE Jones, Analytical Chemistry, 53, 900–902 (1981).

8. http://ts.nist.gov/ts/htdocs/230/235/ir%206969/PDF%20Sections/Sop_2_Mar_2003.pdf

9. RSP Davis, Metrologia, 29, 67–70 (1992).