Method Adjustment for Gradient Elution

You have to be careful to avoid unwanted results when adjusting gradient conditions.

In a recent “LC Troubleshooting” installment (1) we looked at how to adjust liquid chromatography (LC) methods according to the guidelines (2) of the United States Pharmacopeia (USP). In general, the allowable adjustments were restricted to isocratic methods-those with constant mobile-phase composition. In this month’s discussion, I would like to concentrate on how to adjust gradient methods when the flow rate, column dimensions, and particle size are changed. These adjustments are not part of the USP guidelines, but I’m hopeful that someday the USP will include similar guidelines for the adjustment of gradient LC methods.

Retention Factor Is the Key

An earlier series of “LC Troubleshooting” discussions (3–5) covered the influence of different variables on resolution for isocratic methods. Central to the discussion was the fundamental resolution equation:

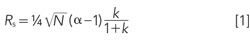
where resolution, R_s, is a function of the column plate number, N, the selectivity, α, and the retention factor, k. The selectivity factor is critical to peak separation:

where k₁ and k₂ are the retention factors of two adjacent peaks 1 and 2. The retention factor is calculated as follows:

where t_R is the retention time and t₀ is the column dead time, often noted as the retention time of the “solvent front.”

Let’s consider next what happens when we change the flow rate, column length, column diameter, or particle size. A change in flow rate will change retention time and the column dead time inversely in proportion to the change. For example, if we change the flow rate from 1 mL/min to 2 mL/min, the retention time will drop to half of the previous value, as will the column dead time. This means that the retention factor (equation 3) will stay constant. If k₁ and k₂ are constant, α will also be constant (equation 2). With particles ≤5 µm in diameter, a flow rate change by a factor of two will have little practical influence on the column plate number, so, as a first approximation, flow rate will not change resolution in isocratic methods.

A change in the column length, L, or internal diameter, d_c, will change the volume of the column according to simple geometry. If the column volume is decreased by a factor of two and the flow rate is constant, the retention time and dead time will drop by the same factor of two; k and α will stay constant, so the separation of the peak centers will remain unchanged. However, any change in column length will affect the plate number, so resolution is expected to change when the column dimensions are changed unless some other adjustment to keep N constant is made, such as changing the particle size.

A change in particle size, d_p, alone will not change k or α, but will change N, so resolution will change (equation 1) inversely with the particle size change. Thus, a change from 5-µm d_p particles to 3-µm ones will change N by 5/3 ≈ 1.7-fold. This will, of course, increase resolution. As we saw previously (1), the USP technique of coordinating the change in L and d_p to keep L/d_p constant results in the same value of N so resolution should not change if k is not changed.

What About Gradients?

The adjustments allowed by the USP guidelines depend on the immunity of k and α to changes in flow rate and column volume in isocratic separations. However, the retention factor and selectivity in gradient elution are not affected in the same way. For gradients, we can restate equation 1 as

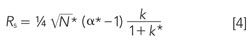
where the asterisk superscripts differentiate gradient from isocratic variables. Selectivity in gradient elution, α*, can be expressed as

where the subscripted k*-values correspond to two adjacent gradient peaks. The gradient retention factor, k*, can be expressed as

where t_G is the gradient time, F is the flow rate, ∆%B is the gradient range, V_m is the column volume, and S is a constant for a given solute. Unlike isocratic separations, where k is different for each peak in a run, k* is approximately the same for every peak. This means that when we make changes in the column volume (by changing length or diameter) or flow rate, k* will change also. If k* changes for one peak, it is likely to change for others as well, just like in isocratic separation (4), so α* is also likely to change.

The USP adjustments for isocratic methods depended on not changing k in the process, so we must take precautions to keep k* constant for the gradient case. In the present discussion, we’re only considering changes in column length, diameter, flow rate, and particle size, so we can simplify equation 6 to

We can also restate the numerator of equation 7 as

where V_G is the gradient volume. This equation tells us that if we keep the ratio of the gradient volume to the column volume constant, we will keep k* constant. This is the key concept to follow for successful gradient method adjustment.

Let’s consider two examples to illustrate the adjustment process according to equation 7. First, what do we do if we want to increase the flow rate from 1 mL/min to 2 mL/min? To keep the gradient volume constant, the easiest adjustment is to reduce the gradient time by half-for example, from 20 min to 10 min. What about a change in column length from 250 to 150 mm? This change is a reduction of 150/250 = 0.6, so we need to adjust the numerator by the same amount. We could either lower the flow rate from 1 mL/min to 1 x 0.6 = 0.6 mL/min or more likely change the gradient time from 20 min to 20 x 0.6 = 12 min. In each of these cases, k* is the same before and after the change, so selectivity should not change.

When we considered only isocratic separations (1), a change in the column diameter required a change in the flow rate to keep the linear velocity constant, and this is a good idea for gradients, too. The flow rate should be adjusted in proportion to the square of the column diameter reduction. For example, moving from a 4.6-mm i.d. column run at 1 mL/min to a 2.1-mm i.d. column would require a flow rate reduction of (2.1/4.6)² ≈ 0.2, to 1 x 0.2 = 0.2 mL/min. Notice that the combination of the diameter and the flow rate satisfies the requirements of equation 7 so that k* remains constant. So the process of adjusting flow rate with column diameter to keep constant linear velocity applies just as well for gradient methods as it does for isocratic ones.

We can summarize the required changes of gradient time, flow rate, column length, and column diameter as

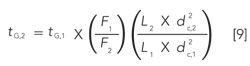
where the subscripts refer to the initial (1) and adjusted (2) conditions. For purposes of the adjustments discussed in conjunction with Table I, we can simplify equation 9 to:

Adjustments for L/d_p

In the discussion of the USP guidelines for isocratic methods (1), we saw that one of the important changes introduced recently was the ability to change column length and particle size as long as the L/d_p ratio was constant within a range of -25% and +50%. This keeps the column plate number approximately constant, and with no changes in the retention factor, the resolution will stay approximately constant. We should be able to do the same kind of changes with gradients, provided that we make any compensating adjustments unique to gradient requirements discussed above. For example, a change in column length from 150 mm to 100 mm with a corresponding change in particle diameter from 5 µm to 3 µm will change L₁/d_p,1 = 150/5 = 30 to L₂/d_p,2 = 100/3 = 33. This means that the L/d_p ratio changes by 33/30 = +10%, well within the -25% to +50% guidelines. However, we saw earlier that the change in the column length will reduce the column volume by 100/150 = 0.67, so we will need to reduce the gradient time by 0.67-fold to keep k* constant so that the selectivity does not change. You can see that there is no reason the isocratic L/d_p adjustment cannot be used with gradients as long as the appropriate adjustments are made for the change in column length to ensure that the gradient retention factor is not changed.

Putting It All Together

At this point, we have all the information we need to enable us to extend the USP’s guidelines for adjusting a gradient method for changes in flow rate, column dimensions, and particle size. For best results, we need to do this in a three-step process:

1.    Use the L/d_p guidelines for the choice of a new column length and particle size.

2.    Adjust the flow rate for any change in column diameter. If you have made additional adjustments to flow rate to compensate for ideal behavior when particle size is changed or for other reasons (see reference 1 for examples), include them here.

3.    Adjust the gradient time for each segment of the gradient to account for changes in flow rate and column dimensions.

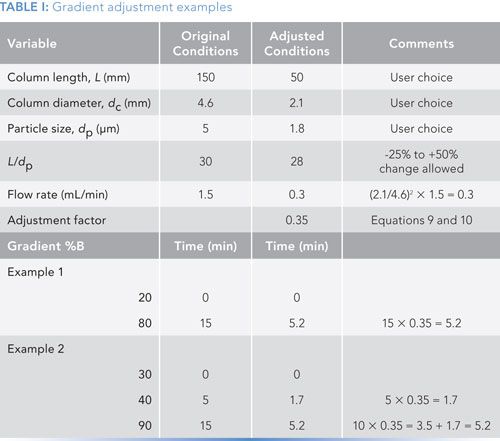
Table I contains two examples summarizing the application of these three steps to two different gradient methods. The methods differ only in the gradient program and illustrate the transfer of a method from traditional LC conditions to ultrahigh-pressure liquid chromatography (UHPLC) conditions. (Note that if you try to repeat my calculations, your numbers may differ slightly-I’ve rounded or truncated numbers for ease of presentation.)

The original methods both use a 150 mm x 4.6 mm column packed with 5-µm d_p particles and operated at 1.5 mL/min. It is desired to use a 50 mm x 2.1 mm column packed with 1.8-µm particles. The first step is to determine if the new column is within the limits for L/d_p. In the original column, L/d_p = 150/5 = 30; for the proposed column, L/d_p = 50/1.8 = 28. This change of 28/30 = -7% is within the -25% to +50% guideline limits, so the proposed column satisfies the L/d_p requirements.

Second, we need to adjust the flow rate to keep the linear velocity constant. As we saw above, the change from 4.6 mm to 2.1 mm i.d. required a reduction in flow rate by a factor of ~0.2, so a flow rate of 1.5 mL/min × 0.2 = 0.3 mL/min is required with the narrower column.

The third step is to adjust the gradient time to compensate for the other changes such that k* stays constant. This adjustment is easiest to do by calculating the gradient adjustment factor defined in equations 9 and 10. For this I get (1.5/0.3) x (50 x 2.1²)/(150 x 4.6²) = 0.35. This adjustment factor will be used to adjust the gradient conditions.

With example 1 of Table I, we have a simple gradient of 20–80% B in 15 min. Applying the adjustment factor, we get a new gradient time of 15 min x 0.35 = 5.2 min. So the new gradient will be 20–80% in 5.2 min. This will keep k* constant to keep the peak spacing the same; the L/d_p adjustment ensured that the column plate number would be constant, so resolution, according to equation 4 will be unchanged.

Example 2 is a little more complicated because it includes two gradient segments, 30–40% B in 5 min followed by 40–90% B in an additional 10 min for a total time of 15 min. The adjustment process is the same here as for example 1, except that we have to treat each gradient segment as a separate gradient. Therefore, we adjust the first segment as 5 min x 0.35 = 1.7 min and the second segment as 10 x 0.35 = 3.5 min, which is added to the 1.7 min segment for a final time of 5.2 min. 

In both cases, if you had a reverse step at the end of the gradient to return to the initial conditions, you would adjust that segment in the same way. For the first example, you might include returning from 80% to 20% in 0.1 min with a 5-min equilibration hold before the next injection. Keep the 0.1-min step and adjust the equilibration to 5 min x 0.35 = 1.7 min; I’d round this to 2 min for convenience.

Don’t Forget the Dwell Volume

One more factor to take into account with gradient adjustments for different sized columns, is the dwell volume. The dwell volume is the system volume between the point the solvents are first mixed and the head of the column. Depending on the design of the system, this can range from 0.2 mL for a well-plumbed UHPLC system using high-pressure mixing to 4 mL or more for a traditional LC system with low-pressure mixing. A difference in dwell volume is one of the major contributing factors in the difficulty of transferring gradients from one LC system to another. I don’t have space to go into this in detail, but you can read more about dwell volume problems in an earlier column (6). 

As a short summary, technically the dwell volume, V_D, should be adjusted in proportion to the change in column volume:

This change is not convenient to make, because dwell volume is not an easily adjustable parameter. When switching from LC to UHPLC, as in the examples discussed here, the smaller dwell volume typical of UHPLC systems may be such that the ratio is close enough to avoid problems. The easiest thing to do is to try the adjusted method and see if it is satisfactory. Dwell volume problems will affect the early peaks in the chromatogram the most, so if your method has well-retained peaks, you are likely to see little, if any, change in separation. If you are developing a new method on a fairly modern LC or UHPLC system, most of these systems have a feature that allows you to generate “zero-dwell-volume” gradients. This is done by starting the gradient and delaying the injection time until the mixed solvent has passed through the dwell volume (the delay equivalent to the dwell time). Thus, the sample and the gradient arrive at the column at the same time. The zero-dwell-volume approach makes gradients easy to transfer if all the instruments to be used have this feature.

Conclusions

We’ve covered a lot of territory to show that it is possible to adjust gradient LC methods for changes in column dimensions, flow rate, and particle size without compromising the method. The key to making these adjustments is to consider the gradient volume as the critical parameter that you need to control. That is, the gradient volume, measured in column volumes (equations 7 and 8), needs to be kept constant. If you follow the three-step process described above (L/d_p, adjust flow rate, adjust gradient time) and keep potential dwell volume problems in mind, you should have success adjusting gradients. The information presented here will fill in the gaps of Table I of reference 1, where changes in column dimensions, flow rate, and particle size were not allowed.

References

1. J.W. Dolan, LCGC North Am. 35(6), 368–373 (2017). 

1. General Chapter <621> “Chromatography” in United States Pharmacopeia 40 National Formulary 35 (USP 40-NF 35, United States Pharmacopeial Convention, Rockville, Maryland, 2017), pp. 508–520.

1. J.W. Dolan, LCGC North Am. 35(3), 170–173 (2017).

1. J.W. Dolan, LCGC North Am. 35(4), 240–245 (2017). 

1. J.W. Dolan, LCGC North Am. 35(5), 306–311 (2017). 

1. J.W. Dolan, LCGC North Am. 31(6), 456–463 (2013).

John W. Dolan

“LC Troubleshooting” Editor John Dolan has been writing “LC Troubleshooting” for LCGC for more than 30 years. One of the industry’s most respected professionals, John is currently a principal instructor for LC Resources in McMinnville, Oregon. He is also a member of LCGC’s editorial advisory board. Direct correspondence about this column via e-mail to LCGCedit@ubm.com

Dwight Stoll is the incoming editor for “LC Troubleshooting,” effective November 2017. Stoll is an associate professor and co-chair of chemistry at Gustavus Adolphus College in St. Peter, Minnesota. His primary research focus is on the development of 2D-LC for both targeted and untargeted analyses. He has authored or coauthored 48 peer-reviewed publications and three book chapters in separation science and more than 95 conference presentations. He is also a member of LCGC’s editorial advisory board.