Practical Gas Chromatography

Questions about how practical proposed gas chromatography (GC) method changes are often come up during optimization for speed and resolution, or while converting to a different carrier gas. Related objective measurements such as the optimum practical carrier gas velocity were defined more than 40 years ago. This instalment reviews such metrics in the light of their relevance to today's GC challenges.

One of the classical trade-offs in gas chromatography (GC) separations lies between speed of analysis and peak resolution. Chromatographers can increase the speed of analysis in a number of ways, including the use of shorter and narrower columns, higher temperatures and temperature programme ramp rates, and faster flow rates, but higher speeds do not guarantee equal or better peak resolution. The relationships between flow or velocity and resolution have recently received attention in the context of a drive towards faster separations, and the on-going substitution of hydrogen carrier gas for helium in many laboratories also fuels the discussion. This instalment of "GC Connections" discusses the effects of increased carrier-gas flow or velocity in an example separation that includes two pairs of solutes.

Optimum Practical Velocity

One of the more neglected separation metrics is the optimum practical carrier-gas velocity (OPGV). This idea is not new: The pioneers of gas chromatography formulated the OPGV as one way to measure the trade-offs between speed of analysis and resolution. As the carrier-gas flow increases above an optimum value, peaks become broader and their resolution starts to decline but they are eluted sooner in proportion to the higher flow. Scott and Hazeldean (1) proposed that an optimum compromise between the two could be found by increasing the flow until the corresponding increase in a plot of plate height versus average carrier-gas velocity becomes essentially linear. An optimum velocity would be reached at the point where additional losses of resolution because of further increases in velocity could not be compensated for by a corresponding increase in column length.

Without experimental measurements from multiple columns, the OPGV has been considered as the velocity at which a tangent line from the origin meets a plot of measured values of the plate height, H_meas, versus the average linear carrier-gas velocity, u withbar. This is the velocity at which the quantity H/u withbar hits a minimum (2). A plot of experimental H_meas versus u withbar departs from the linear at higher velocities because of secondary gas-compression effects at higher pressures and extracolumn broadening from the detector if the peaks become narrow enough. The basic Golay equation, however, neglects such effects. A plot of the theoretical plate height, H_theor, versus u withbar will never become completely linear:

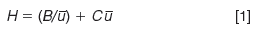
where H is the height of one theoretical plate, u withbar is the average carrier-gas linear velocity, B describes the broadening of a peak because of gas diffusion along the direction of carrier-gas flow, and C describes broadening because of the effects of solute molecules entering and leaving the stationary phase. As the linear velocity (flow) increases, a decreasingly small fraction of the total theoretical plate height is a result of the B term, and the C term dominates.

These effects are shown in Figure 1 for the basic Golay equation using a 25 m × 0.53 mm column. In this, and the subsequent column treatments, the influence of the stationary phase on solute broadening is minimal; the stationary-phase film thickness used was 0.4 µm, which influenced this plot by less than 2%. Plot (a) is the total theoretical plate height; plot (b) is the B term contribution; and plot (c) is the C term contribution. Plot (c) also represents a tangent that meets the total plate height (a). That this junction occurs at infinite linear velocity shows the fundamental difficulty with using the basic Golay equation this way for OPGV calculations.

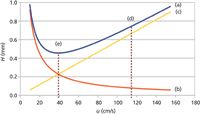
Figure 1: Plot of the basic Golay equation for n-hexane: (a) total plate height, (b) B term contribution to the plate height, (c) C term contribution to the plate height, (d) OPGV where the B term contribution accounts for 10% of the total plate height, and (e) optimum carrier-gas velocity. Theoretical column parameters: 25 m × 0.53 mm, 0.4-µm nonpolar stationary-phase film thickness, 130 °C, helium carrier gas.

The basic Golay equation yields an infinite linear velocity if a tangent-line construction is used to find the OPGV because the theoretical relationship neglects the effects on plate height of operating at higher inlet pressures and of producing potentially very narrow peaks. The theoretical plot does not curve away from a linear relationship at elevated velocities, but experimental data do. Although it is a convenient way to explain idealized column band-broadening behaviour without making arduous measurements of H_meas versus u withbar data for multiple columns, widespread use of the basic Golay equation has resulted in the neglect of OPGV as a means of expressing a practical upper limit for average linear velocity in specific separations.

A simple approach to determining a finite value for OPGV from the basic Golay equation is to choose an arbitrary point that sets the OPGV at the velocity where gas–gas diffusion contributes a fixed percentage of the overall band broadening. Figure 1 illustrates an example at the point labelled (d), where gas–gas diffusion contributes 10% of the total band-broadening and u withbar = 117 cm/s. The optimum velocity, u withbar _opt— the point at which H is at a minimum — is shown as well at point (e), where u withbar = 39.2 cm/s. But this idealized and arbitrary OPGV point is not connected to physical properties that accommodate the effects of an increasing inlet pressure gradient on peak broadening; the velocity of 117 cm/s seems too high for a reasonable upper limit. Extended band-broadening theories that do include such effects can produce a better theoretical picture of the effects of increasing the velocity.

Practical Theory

A number of authors have proposed more-complete theories, including Golay himself, although his extended equations address porous-layer open-tubular (PLOT) and support-coated open-tubular (SCOT) columns and do not consider how gas–gas diffusion is affected by carrier-gas compression inside the column. A relationship proposed by Giddings (3) works well as a theoretical model for determining OPGV values that are closer to experimental data. Such calculations are only as good as the model and the accuracy of the applied physical parameters, of course, but they can provide useful insight for the selection of practical operating gas velocities or flows.

The B and C terms of the Golay equation (equation 1) are proportional to the rate at which solutes diffuse through the carrier gas. Thus, band-broadening increases as the gas–gas diffusion rates increase. The Golay equation considers these diffusion rates at the column outlet pressure (atmospheric pressure) alone and does not consider the effect of the higher pressures and gradient inside the column itself. Physical measurements as well as theoretical treatments of gas diffusion show that diffusion is inversely proportional to pressure: Diffusion slows down as pressure increases. The effect of intracolumn carrier-gas pressure on diffusion rates is not very large at low inlet pressures, such as in a 0.53-mm i.d. column. As inlet pressures rise the effect becomes more significant — for example, with a 0.25-mm i.d. column — especially at higher linear velocities.

Giddings' equation applies a pressure correction factor that accommodates the net effect of increasing diffusion rates on band-broadening as solutes progress along the column. The correction is larger with increasing inlet pressure and, thus, follows the influence of higher carrier-gas velocities. (The details of this correction factor and the Giddings equation are available for interested readers as supplementary material on-line at http://wiki.hrgc.com.)

In addition to the influence of the carrier-gas pressure, diffusion rates are different for different solutes. They grow smaller with increasing molecular weight. The diffusion rate of n-hexane, for example, in helium (or in any other carrier gas) is about 40% faster than that of n-dodecane. Temperature plays a role too; gases diffuse more rapidly at higher temperatures. The current discussion is limited to isothermal conditions so variable temperature isn't a concern, but its influence is significant when temperatures or programming rates are changed as part of optimization.

Figure 2 shows a series of Giddings theoretical plate height versus u withbar curves for n-C₆, n-C₈, n-C₁₀, and n-C₁₂ on a 25 m × 0.25 mm column at 130 °C. Theoretical diffusion coefficients for each solute were used as listed by Ettre (4). The column pressure drops were calculated from theory as well, using the same relationships found in instrumental electronic pneumatics.

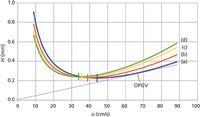
Figure 2: Plots of the Giddings equation for a series of hydrocarbons: (a) n-hexane, (b) n-octane, (c) n-decane, and (d) n-dodecane. Dashed line: tangent that intersects plot (a) at its OPGV. Theoretical column parameters, same as Figure 1 except for column internal diameter of 0.25 mm.

The influence of the individual solute diffusion rates on plate height is quite clear. Each solute takes on its own optimum average linear velocity, as marked on each plot in Figure 2. That different solutes have different u withbar_opt values is not new information, although the span of the optima in this case — from 34 cm/s for n-C₁₂ to 44 cm/s for n-C₆ — appears wider than might be expected. Without considering the OPGV for the moment, the optima plainly show that biasing the carrier-gas velocity on the high side appears to be a good idea: Small losses in efficiency are taken while achieving a faster separation. But what about the high end of linear velocity for speed optimization purposes?

With the Giddings equation a tangent line from the origin intersects each curve at a well-defined point, and these points correspond to the original definition of the OPGV. The tangent line for n-C₆ is drawn in Figure 2; it intersects the plot at u withbar = 68 cm/s. Figure 3 illustrates another way of expressing the OPGV by plotting the number of plates generated per second as a function of the average carrier-gas velocity. The maxima of these plots correspond to each solute's OPGV. Similar to the span of u withbar_opt, the OPGV values range from 54 cm/s for n-C₁₂ up to 68 cm/s for n-C₆.

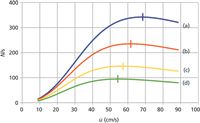
Figure 3: Plots of theoretical plates per second from the Giddings equation: (a) n-hexane, (b) n-octane, (c) n-decane, and (d) n-dodecane. Vertical tick marks on each plot show the maximum, at the OPGV. Theoretical column parameters, same as in Figure 2.

Taken together, Figures 2 and 3 would define a range for minimum and maximum optimized average velocities across the scope of the idealized normal hydrocarbons that were employed, from the highest optimum velocity of 44 cm/s to the lowest OPGV of 54 cm/s. Compared to operating at the lowest optimum of 34 cm/s, a velocity of 54 cm/s would decrease the analysis time by roughly 38% while sacrificing about 20% of the theoretical plates generated for the later-eluted n-C₁₂. Running the separation at 44 cm/s would restore much of the plate number while sacrificing some of the gain in analysis time.

These trade-offs in column efficiency for speed are also not new information, but here the OPGV values provide a more meaningful upper end for a range of velocities than do arbitrary velocities based on simple percentages or multipliers, while preserving most of the column's separating power. And as always, the best procedure is to determine actual experimental performance for the analytes of interest. Theoretical predilections provide useful guidelines and place boundaries on the range of practical conditions, but they are no substitute for real data.

Practical Resolution

Chromatography is all about peak resolution, not just the efficiency of individual solutes. The discussion so far has considered solutes standing alone, ones that are well separated under almost any conditions at that. Applying the Giddings equation to pairs of closely eluted solutes provides some more information and guidance for selecting optimized velocities by putting the modelled separation into a context of resolution. Two pairs of peaks were chosen so that the resolution, R_s, of each pair will range around 2.0. This exceeds the minimum "baseline" resolution of 1.5 that is often considered good enough, but a resolution of 2.0 does provide some working room for eventual performance losses because of column degradation and also makes for a more robust method. Many separations do exhibit more than one critical pair of peaks, those that are resolved at close to the minimum, so it is useful to consider what happens to solute pairs at the beginning and end of a separation as the velocity is optimized.

Figure 4 shows the resolution that would be obtained in theory between two pairs of solutes, n-hexane with a closely following hypothetical analogue, and n-dodecane with another closely eluted analogue. Each solute pair is separated from its neighbour with a separation factor, α, of 1.03. Each pair of solutes shows an optimum resolution at close to the optimum linear velocity, which is expected because they both share nearly identical properties. The later-eluted pair (Figure 4[b]) does gain some resolution at optimum over the earlier pair (Figure 4[a]), which is also expected because the later pair simply has more time in the column for resolution to develop. A bias towards higher velocities up to the OPGV is again clear, even more so than is apparent for the individual solutes' efficiencies as shown in Figure 2. It is also interesting to see that both pairs' resolution declines linearly with nearly the same slope when the carrier-gas velocities are pushed higher above their OPGV levels. If a minimum resolution of 1.5 were the goal for these peak pairs, then a velocity of around 82 cm/s would be acceptable and would realize a gain in speed of analysis of approximately 2.5-fold.

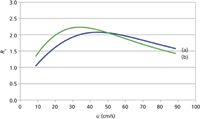
Figure 4: Plots of resolution for two pairs of solutes, using the Giddings equation: (a) n-hexane and a closely eluted analogue and (b) n-dodecane and a closely eluted analogue. Separation factor for both pairs α = 1.03. Theoretical column parameters, same as in Figure 2.

Conclusion

Both efficiency and resolution are critical for obtaining acceptable separations. With extra resolution available, a column can be pushed to higher linear velocities or flows while still obtaining a minimum performance level. Short of experimental data, theoretical treatment of a separation can yield useful information about how performance would be affected by increasing the speed of analysis, but only to the degree that the theory reflects the chromatographic process. Application of an extended theory such as the Giddings equation provides additional insight beyond the basic Golay equation, and this helps to frame practical limits on optimization for speed of analysis. But in cases where peak resolution is minimal, there is no substitute for careful experimental evaluation of a faster separation scheme.

John V. Hinshaw is a senior scientist at BPL Global Ltd., Oregon, USA, and is a member of the LC•GC Asia Pacific editorial advisory board. Direct correspondence about this column should be addressed to "GC Connections", LC•GC Asia Pacific, 4A Bridgegate Pavillion, Chester Business Park, Chester, CH4 9QH, UK, or email the editor-in-chief, Alasdair Matheson, at amatheson@advanstar.com

References

(1) R.P.W. Scott and G.S.F. Hazeldean, in Gas Chromatography 1960, R.P.W. Scott, Ed. (Butterworths, London, UK, 1960), pp. 144–161.

(2) W. Jennings, Analytical Gas Chromatography (Academic Press, Orlando, Florida, USA, 1987), pp. 77–79.

(3) J.C. Giddings, S.L. Seager, L.R. Stucki, and G.H. Stewart, Anal. Chem. 32, 867–870 (1960).

(4) L.S. Ettre and J.V. Hinshaw, Basic Relationships of Gas Chromatography (Advanstar, Cleveland, Ohio, USA, 1993), p. 47.