Tools for Simulation and Optimization of Separations in Ion Chromatography

Users of ion chromatography (IC) are faced with lengthy method development times because of the relatively slow equilibration of IC columns to each new eluent. Method development becomes even more demanding when multistep elution profiles (containing sequential isocratic and gradient steps) are used. Given that modern IC instruments are designed to generate these multistep elution profiles, computer-based methods to simulate separations for a wide range of analytes, columns, and eluents are highly desirable. The tools available for computer-assisted method development are discussed here.

Ion chromatography (IC) is used widely for the detection and determination of ionic species in various samples. With the advent of eluent generators and electrolytic suppressors, IC is now operated routinely using both isocratic elution and multistep eluent profiles comprising sequential isocratic and gradient steps. Frequently, an eluent profile consisting of multiple steps (commonly up to five) is applied, typically when the separation is difficult or the number of analytes to be separated is large. The practical implementation of these multistep eluent profiles is simplified greatly by the use of an eluent generator in which the eluent is generated electrolytically from an input stream of water. The desired eluent concentration profile can be created simply by applying a suitable profile of electrical current to the eluent generator.

The development of a new IC separation method involves many decisions. Which column should be used? Which eluent type should be used and what is the optimal eluent composition? As with most chromatographic techniques, the development of a new IC method can be a time-consuming process. Trial-and-error optimization of the separation is therefore both tedious and challenging. For these reasons, there has been a strong interest in the use of computational tools to facilitate the method development process.

Considerable work has been undertaken to understand the retention processes that apply in IC. Over the years, there have been numerous attempts to produce mathematical retention models for isocratic elution (1–5), gradient elution (6), and multistep elution profiles (comprising sequential isocratic and gradient steps) (7–10) in IC. These models aim to provide a mathematical relationship between the analyte retention factor and measurable properties of the analyte (such as its charge), the eluent driving ion (such as its concentration and charge), and the stationary phase (such as its ion-exchange capacity and phase ratio). Many of the published models are remarkably accurate in their prediction and in theory, they could be used for the a priori calculation of retention factor for any analyte without the need for experimentation, provided that values for all of the above properties are known. This situation rarely occurs in practice because the process of determining values for all the necessary analyte, eluent, and stationary-phase properties would normally take longer than a straightforward trial-and-error manual optimization. For this reason, computer-assisted method development in IC almost invariably involves a combination of experimentation and computer prediction. The experimentation step is done to acquire some limited retention data using, for example, three eluent conditions, and then applying the chosen mathematical retention model to calculate analyte retention for all possible eluent conditions. This process is represented schematically in Figure 1. This process shows that, provided the retention model is accurate and sufficient experimental data are available to permit reliable implementation of the model, it should be possible to quickly produce a retention map for all analytes over all eluent combinations (the "search area"), leading to straightforward simulation and optimization of separations.

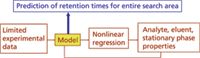
Figure 1: Schematic overview of computer-assisted optimization in IC.

Retention Models in IC

It is not the intention of this article to discuss in any detail the theoretical basis underlying IC retention models. However, it is pertinent to show which models are most useful for the process outlined in Figure 1. It is also often the case that the most useful models are surprisingly simple.

Isocratic elution in IC is described accurately by the following relationship:

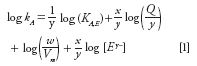
where k_A is the retention factor, k_A,E is ion-exchange selectivity coefficient between the analyte and the eluent competing ion, x is the charge of the analyte, y is the charge on the eluent, Q is the effective ion-exchange capacity of the stationary phase, w is the mass of the stationary phase, V_m is the volume of the eluent species, and [E^y- ] is the concentration of the eluent.

If this model is employed for isocratic separations consisting of a single competing ion, k_A,E, Q, w, and V_m can be treated as constants and thus the model can be simplified to

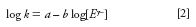
where a and b are both constants.

A plot of log k versus log[E^y- ] will give rise to a linear relationship with the effective charge of the analyte relative to the competing ion as the slope, b, and the intercept, a, indicating the degree of interaction between analyte and stationary phase. This linear relationship is illustrated in Figure 2 for a univalent eluent ion (OH^- ) and a series of inorganic and organic univalent analyte ions.

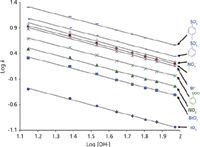
Figure 2: Plot of log k versus log [OH-] for inorganic and organic anions.

Retention modeling for gradient elution IC or for separations involving multistep elution profiles is understandably more difficult than is the case for isocratic separations. Generally, two approaches can be used. In the first, a mathematical gradient retention model is derived, but this generally involves quite complex derivations, requires accurate gradient retention data to solve the model, and can also be challenging computationally when calculations of retention factors under numerous eluent conditions are undertaken. The second, and preferred, approach is to break up the gradient or multistep elution profile into a series of small, successive isocratic segments, to then apply an isocratic retention model to each segment, and finally to integrate the individual segments into the overall elution profile. This process is illustrated schematically in Figure 3. The chief advantages of this approach are that only isocratic retention data are required (because an isocratic retention model is used), the same model can be used for both isocratic and gradient steps of an elution profile, and computational demands are less for isocratic models.

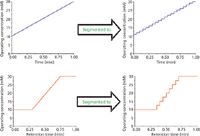
Figure 3: Schematic overview of segmenting gradients and multistep profiles to isocratic segments.

Simulation and Optimization of Isocratic Separations in IC

It is clear from Figure 2 that the isocratic retention model shown in equation 2 gives a very accurate description of retention behavior. It is also evident from Figure 2 that the retention data for any of the analytes shown could be predicted if a minimum of two experimental data points were used to calculate the intercept (a in equation 2) and slope (b in equation 2) of the plots shown in Figure 2. Thus, when applied to the simulation of isocratic IC separations, the process depicted in Figure 1 would have the following steps:

• Retention factors for all analytes in the test mixture would be determined using at least two eluent concentrations.

• These retention data would be used to solve equation 2 for each analyte to obtain a and b values for the retention plot for each analyte.

• The a and b values can then be used to calculate the retention factors for each analyte at any isocratic eluent concentration. In this way, the chromatograms obtainable for any isocratic elution composition can be simulated.

After the ability to predict retention has been achieved, it is then a simple further step to include optimization. This involves using a mathematical algorithm that can assign a numerical value to an entire chromatogram, according to its alignment with a desired goal. For example, this goal might be to maximize the separation of all peaks, to maximize the separation of a problematic pair of peaks, or to achieve the separation in the fastest possible time. Such an algorithm is referred to as an optimization criterion, and many such algorithms have been proposed.

It is clear from the above comments that reliable isocratic retention data are essential for the successful implementation of simulation and optimization of isocratic separations in IC. Of course, these retention data can be acquired as needed for particular combinations of analytes, eluents, and stationary phases. An alternative approach is to compile an extensive set of reliable and accurate isocratic retention data for a wide range of analytes, eluents, and columns and to store these data in a suitably accessible database. In this way, users could simulate and optimize separations by accessing the database, without the need to undertake any experimentation. The authors have worked with Thermo Scientific Dionex (hereinafter referred to as Dionex) over the past 10 years to compile such a database and this has formed the basis of the Virtual Column software for IC simulation and optimization, marketed by Dionex. The database underlying this software contains retention data for more than 75 analytes (anions and cations) on more than 20 Dionex IC columns using a range of eluents, column diameters, and temperatures. The user simply needs to select the analytes that are to be separated and the software can then be used to simulate all possible chromatograms on all columns and to identify the optimal combination of eluent and column for the desired separation.

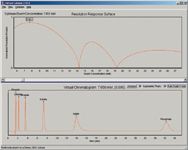
Figure 4: Screen display from Virtual Column software for isocratic separations.

One example of the output (in its simplest form) generated by the Virtual Column software is shown in Figure 4. In this case, fluoride, chloride, bromide, sulfate, iodide, and phosphate are being separated on a Dionex AS11 column using hydroxide eluents in the range 4.5–28 mM. The top panel shows a plot of the optimization criterion (in this case, the normalized resolution product, which reaches a maximum value of 1.0 when all peaks are spaced evenly over the chromatogram, and has a value of zero when any two peaks are coeluted). It can be seen that the maximum value of this criterion is reached when the eluent concentration is 7.65 mM. The bottom panel displays the chromatogram obtained with this eluent concentration. There is a slider bar at the bottom of the top panel and the user can slide this to any desired eluent concentration and the chromatogram obtainable at this concentration will appear in the bottom panel. The software also allows other columns, diameters, and temperatures to be investigated, as well as the use of other optimization criteria. In addition, two-component eluents (such as mixtures of carbonate and bicarbonate) can be simulated, in which case the top panel reverts to a response surface plotted against the concentrations of the two eluent components on the horizontal and vertical axes.

Simulation and Optimization of Multistep Elution Profiles in IC

Because of the ease with which multistep eluent profiles containing sequential isocratic and gradient steps can be generated, and the versatility that they offer in developing new separations, such profiles are now the preferred mode of operation in modern IC. However, the selection of an optimal eluent profile is a very challenging and time-consuming task if trial-and-error procedures are used. For this reason, there has been very strong interest in extending the isocratic approaches described above for use with multistep elution profiles.

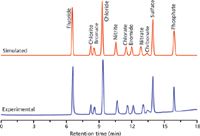
Figure 5: Simulated and experimental separations on a Dionex AS19 column under a three-step gradient profile consisting of: 3.75 mM KOH for 0.8 min, 3.75 to 33.75 mM KOH for 10 min, followed by 33.75 to 99.75 mM KOH for 4 min at 1 mL/min at 30 °C.

The earlier discussion of retention models highlighted the potential use of isocratic retention data for gradient elution by dividing the gradient into successive small isocratic segments. If this approach is taken, only isocratic retention data are needed for calculation of retention factors, so the same isocratic database used for the Virtual Column isocratic software can be applied. Simulation performed in this way is highly successful, as evidenced by Figure 5, which shows the simulated and experimental chromatograms for the separation of 11 anions on a Dionex AS19 column using a three-step elution profile. Figure 6 shows the overall correlation between experimental and simulated retention times for 37 analytes (anions and cations) on several columns using four different five-step elution profiles.

Figure 6: Correlation of experimental and simulated retention times for 24 anions and 13 cations on Dionex AS11 HC, AS16, AS19, CS12A, and CS16 columns for four 5-step complex eluent profiles.

Figures 5 and 6 show that accurate simulation of retention under multistep elution profiles is possible. However, optimizing the separation by finding the optimal elution profile is an exceedingly difficult task because of the large number of parameters that need to be optimized. For example, in a simple three-step elution profile comprising an isocratic step, a gradient step, and then a final isocratic step, it is necessary to optimize the initial eluent concentration, the duration of the first step, the slope and duration of the gradient step, and the duration of the final isocratic step. The complexity increases when more steps are included in the eluent profile. Currently, there are no published methods for this type of optimization. However, it is possible to simulate the separation using the approach depicted in Figure 7. Here, a four-step elution profile is depicted, with an initial isocratic step, a shallow gradient, a steeper gradient, and a final isocratic step. The square boxes represent points where the shape of the profile can be adjusted on a computer screen. For example, the length of the first isocratic step can be adjusted by moving the first box left or right. Any change made to the profile will lead to the instant simulation of a new chromatogram by the software. Users can thereby simulate any desired eluent profile and can optimize the separation manually without experimentation.

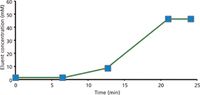
Figure 7: Screen display for manipulation of a multistep elution profile.

Updating the Retention Database

An obvious potential limitation of the above approaches to simulation and optimization is that they rely on retention data stored in the Virtual Column database, which may become dated. For example, a new column might not behave in the same manner as the column used to acquire the data some years previously. We have addressed this issue by devising a system where the database can be updated (or "ported") to a new column by making adjustments to the stored data, based on some actual measurements obtained on the new column on which the IC method is to be used (11). In this way, the database can be continually adapted for use with newer versions of the columns on which the original data were obtained.

Conclusions

The ability of modern IC instruments to apply multistep elution profiles has placed severe demands on method development procedures designed to exploit the full advantages of these elution profiles. Retention models derived for simple isocratic IC separations can be applied to multistep eluent profiles by segmenting these profiles into small isocratic steps. This approach simplifies the retention modeling process and enables rapid calculation of retention factors based only on isocratic data. The computational simplicity of these calculations means that real-time simulations can be performed. Historical isocratic retention databases can be easily updated with a small amount of experimentation. These new tools are not yet available in the commercial Virtual Column software, but work is currently under way to update this software.

References

(1) J. Havel, J.E. Madden, and P.R. Haddad, Chromatographia 49, 481–488 (1999).

(2) P.R. Haddad and R.C. Foley, J. Chromatogr. 500, 301–312 (1990).

(3) J.E. Madden and P.R. Haddad, J. Chromatogr. A 829, 65–80 (1998).

(4) J.E. Madden and P.R. Haddad, J. Chromatogr. A 850, 29–41 (1999).

(5) P. Hajós, O. Horváth, and V. Denke, Anal. Chem. 67, 434–441 (1995).

(6) J.E. Madden, N. Avdalovic, P.R. Haddad, and J. Havel, J. Chromatogr. A 910, 173–179 (2001).

(7) R.A. Shellie, B.K. Ng, G.W. Dicinoski, S.D.H. Poynter, J.W. O'Reilly, C.A. Pohl, and P.R. Haddad, Anal. Chem.80, 2474–2482 (2008).

(8) T. Bolanča, Š. Cerjan-Stefanović, M. Luša, M. Rogošić, and Š. Ukić, J. Chromatogr. A 1121, 228–235 (2006).

(9) P.J. Zakaria, G.W. Dicinoski, M. Hanna-Brown, and P.R. Haddad, J. Chromatogr. A 1217, 6069–6076 (2010).

(10) P.J. Zakaria, G.W. Dicinoski, B.K. Ng, R.A. Shellie, and M. Hanna-Brown, P.R. Haddad, J. Chromatogr. A 1216, 6600–6610 (2009).

(11) B.K. Ng, R.A. Shellie, G.W. Dicinoski, C. Bloomfield, Y. Liu, C.A. Pohl, and P.R. Haddad, J. Chromatogr. A 1218, 5512–5519 (2011).

Robert A. Shellie is an associate professor at the University of Tasmania, where he is a member of the Australian Research Centre on Separation Science (ACROSS). Having worked in the area of hyphenated techniques in chromatography for more than a decade, his research has largely focused on the exploration of approaches for separation and characterization of complex multicomponent samples. He has been actively engaged in the development and application of retention models for rapid method development since 2006.

Robert A. Shellie

Boon K. Ng is a Science and Endowment Industry Funded Postdoctoral Fellow at the University of New South Wales, Australia. His PhD focused on the development of computer-assisted optimization tools for method development in ion chromatography. His current research interests are in ion chromatography and coordination chemistry.

Boon K. Ng

Greg W. Dicinoski is an associate professor and the Head of the School of Chemistry and the Deputy Director of the Australian Centre for Research on Separation Science. He leads projects relating to counter-terrorism and pharmaceutical analysis. Specific focus is given to theoretical aspects, including the modelling and optimization of retention in chromatographic systems and mobility simulation in electrophoresis, and specialist applications for the separation of target chemical species using reversed-phase and ion chromatography, and capillary electrophoresis.

Greg W. Dicinoski

Paul R. Haddad is a Distinguished Professor at the University of Tasmania, Australia, and he is the Director of the Australian Centre for Research on Separation Science (ACROSS). He has very diverse interests spanning separation science and is the author of over 500 publications in this field. Method development in ion chromatography has been one of his strongest research interests. Please direct correspondence to: paul.haddad@utas.edu.au

Paul R. Haddad