A Practical Understanding of Partition Coefficients

Partition coefficients, log P or K_ow, are often used in introducing and describing new extraction techniques. They can also be used in developing new separation methods. An understanding of partition coefficients, along with diffusion, is necessary to more completely guide separation processes. As with the application of any theoretical concepts, several assumptions may be applied. This month, we take a dive into the practical applications of partition coefficients as they are commonly applied to chemical extractions.

With apologies to Mark Twain and other famous orators, one of my favourite quotes of all-time (excluding song lyrics and quotes from Yogi Berra) comes from the 50-year-old textbook used in my sophomore quantitative analysis class:

“If two compounds are to be separated, we must, somewhere along the line, get them into two different and separable phases. At the heart of any chemical separation are the processes of 1) phase contact and equilibrium; and 2) phase separation. These steps occur in all separation techniques, and a key to understanding a given method is the identification and classification of the steps according to the nature of the phases involved and the mechanism of phase contact and separation. Similarly, if a particular method of separation is to be improved, these are the only processes worth adjusting (1).”

This quote so eloquently places the fundamentals of chemical separations squarely in the understanding of equilibria. Thus, when developing new techniques or describing the methodology of chromatography and extraction, one frequently encounters the terms partitioning, distribution ratio, octanol-water partition coefficient, Log P, K_ow, and D. What do these terms mean, or are they synonymous? When do we use these concepts? Why are they important?

Let’s start by defining these terms, at least for the most important versions of partition coefficients:

The partition coefficient, designated P (or, more commonly, log P), is a ratio of the concentration of a compound at equilibrium when disbursed between two immiscible phases. As an equilibrium constant, it is dependent on temperature. Partition coefficients may be estimated by the ratio of the solubilities of the solute in each solvent and the term generally, but not always, refers to liquid mixtures (such as two-phase solutions). The two immiscible phases are undefined by this definition, so log P is generally used for more descriptive purposes, and more specific constants are used for individual situations. Some of these more important coefficients are described.

The distribution ratio, typically abbreviated as D, is similar to the partition coefficient, with the important exception that all forms of the solute are taken into account; that is, if the compound is known to ionize, associate or dissociate, volatilize, or decompose, one may replace log P with log D. If the species of interest remains in a single chemical form, partition coefficients may be used. This is especially important, for example, when the pH of a system, relative to the compound’s pK_a , may lead to protonation or deprotonation. Note, however, that the distribution ratio does not discriminate between the individual forms of a compound, but rather adds the concentrations of each version in a given phase. Distribution ratios are increasingly important when performing mass balance calculations.

Octanol-water partition coefficients, or K_ow, are commonly used versions of partition coefficients used to describe the lipophilicity (fat solubility) or hydrophilicity (water solubility) of a compound. In this case, the immiscible phases are n-octanol and water. K_ow values are often used by environmental chemists, biochemists, or toxicologists to estimate environmental fate (or distribution), cellular uptake, or bioaccumulation. For example, in the fields of pharmacokinetics and toxicokinetics (a compound’s adsorption, distribution, metabolism [biotransformation], and excretion properties), K_ow values are used in predicting a compound’s bioavailability according to the Lipinski Rule of 5. Since n-octanol is a good representation of nonpolar solvents in general, K_ow values are good predictors of suitable solvents for extraction from aqueous samples. This value is so important and so commonly used that K_ow tabulations are commonplace in electronic or physical databases.

Henry’s law constants, K_H, or air-water partition coefficients express the ratio of a compound’s partial pressure in air to the concentration of the compound in water, again dependent on temperature. If expressed in terms of atmospheres of pressure and mole per cubic metre of water, and if the compound is less than 200 daltons, the compound is generally considered volatile. K_H may, for example, be applied to headspace extractions. Extraction sensitivity, or analyte concentration in the gas phase, is related to K_H and the phase ratio (β); that is, the ratio of water to headspace. Since temperature has minimal effect on phase ratio, sensitivity will be directly related to K_H.

Adsorption-desorption distribution coefficients, K_d, are used in studies to model or predict environmental fate because of partitioning of a compound between water, soil, sediment, or other compartments. More so than other partition coefficients, K_d is dependent on a variety of matrix and environmental factors. K_d values and their measurement often do not account for the shape of the adsorption isotherm—that is, the nature of the sorptive interaction between the solute and the matrix. Consequently, laboratory measurements of K_d can be difficult to extrapolate to the field, though general trends can be observed. To overcome this issue, K_d is often normalized to the organic carbon content of the matrix to determine the organic carbon-water partition coefficient, K_oc.

Uses of Partition Coefficients in Extraction

We’ve previously suggested that K_ow values can be used in solvent selection during analytical extractions. One would generally prefer a solvent with a log P value equal to or greater than the log K_ow. Of course, extractions are dependent on more than solute solubility. Diffusivity, compatibility with the analytical method, and green considerations are also important. We’ve also looked at the relationship between Henry’s law constants and headspace concentrations, including the role of temperature.

Partition coefficients play an important role in understanding the quantitative nature of analytical extractions. Consider, for example, the situation with batch extractions. If we can define a partition coefficient as K = [A]_org/ [A]_aq where [A] is the analyte concentration in the organic (org) or aqueous (aq) phases, we can estimate the extraction efficiency following a certain number of batch extractions:

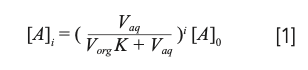
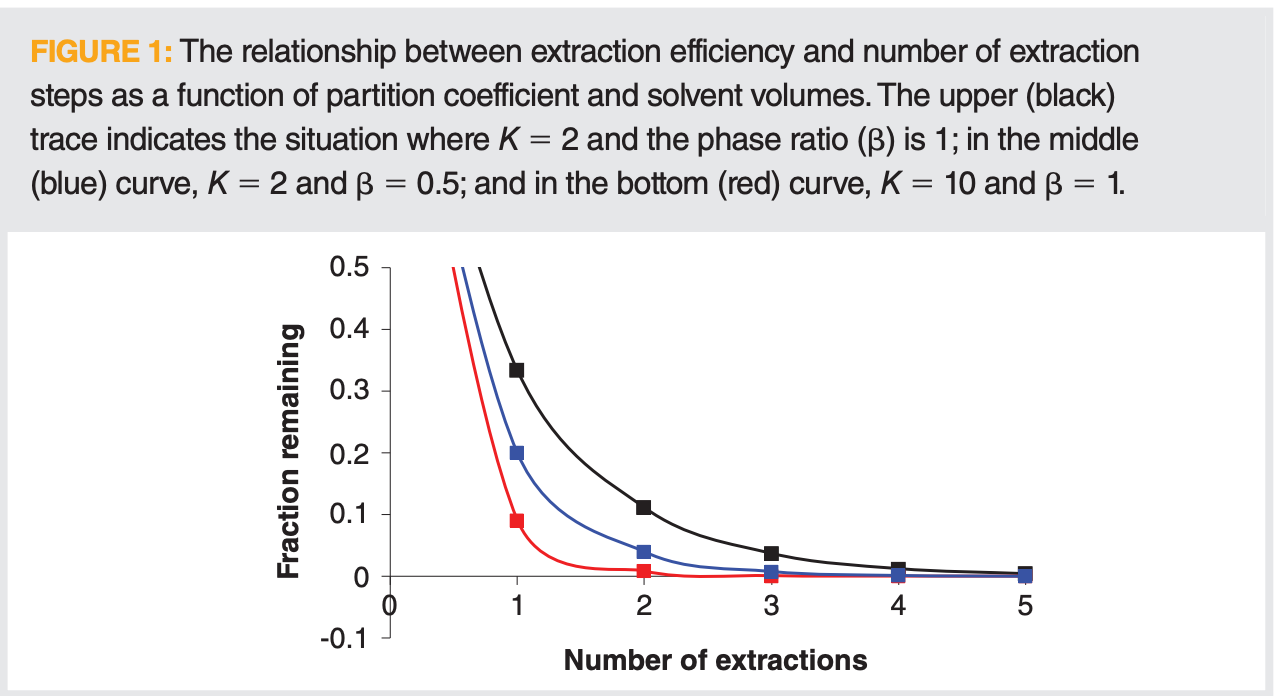
where [A]_i is the concentration of analyte in the aqueous sample phase after i number of batch extractions, [A]₀ is the analyte concentration in the original aqueous sample, and V_aq and V_org are the volumes of aqueous and organic solvent, respectively, in each batch. For example, in Figure 1, we can see an application of this concept. In Figure 1, we can use the green (upper) plot as our baseline case, where equal amounts of aqueous and organic solvents (say, 100 mL of each, for example) at a partition coefficient of 2 are used. This means that two units of analyte would move into the organic phase with one unit (or 33% of the original amount) remaining in the aqueous sample. Upon a second extraction, one third of the 33% of aqueous analyte remaining in the sample, or 11%, remains in the sample. If we define quantitative extraction as greater than 95% recovery, then a third extraction to yield just over 96% extracted, with 300 mL of solvent required. If we aim to improve the extraction method by doubling the amount of organic extracting solvent to 200 mL, then the first extraction would isolate 80% of the analyte into the organic phase. A second extraction would remove 80% of the remaining 20% in the aqueous sample, or a cumulative 96% of the analyte is extracted. However, in this case, 400 mL of solvent is required. However, if a different extracting solvent with a larger partition coefficient (K = 10) is used with the same phase ratio as our baseline situation, diagrammed in red, then in the first extraction, ten units of analyte extract into the organic phase and one unit, or 9%, of analyte remains in the aqueous sample. With a second extraction, just over 99% extraction yield is achieved with the use of only 200 mL of solvent. This example shows the importance of favourable partition coefficients during solvent selection.

The trend towards microscale extractions relies on the knowledge that these separations are equilibrium based, and are thus dependent on understanding partition coefficients. For example, in solid-phase microextraction (SPME), stir-bar sorptive extraction (SBSE), and related sorptive extractions, one can use K_ow to approximate the stationary phase/water partition coefficient. Then K_ow ≈ [A]_stat/[A]_aq where [A]_stat is the analyte concentration in the stationary (extracting) phase. Breaking down the concentrations to their mass (m) and volume components, K_ow ≈ (m_stat/m_aq) (V_aq)(V_stat) or β(m_stat/m_aq). Thus, as long as the phase ratio is constant (that is, a constant volume is used for all samples and standards), the amount of analyte adsorbed directly correlates to the amount of analyte in the sample. Of course, one must be sure to extract for a long enough time to achieve equilibrium. For solvent-based microextractions, such as single-drop extraction, absolute phase immiscibility is required, since the extracting phase is not immobilized on a fibre or other support.

The above treatment of partition coefficients applied to extraction assumes a neutral solute, or one which does not hydrolyze, ionize, or otherwise degrade. For these more complicated situations, one must use distribution coefficients.For example, in the case of a weak acid, HA, which may dissociate in water, the acid dissociation constant, K_a, results from the aqueous reaction HA + H₂O ↔ H₃O⁺ + A^-. Note that di- or tri-protic acids will have multiple, stepwise dissociations, each with its own K_a. We must also be concerned with the partitioning of the neutral species, HA, into the nonpolar organic phase. Thus, the distribution coefficient is:

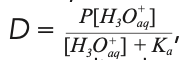
keeping in mind that P is the generalized partition coefficient for HA. The extent of protonation or deprotonation, and consequently D, is related to the pH of the aqueous sample relative to the pK_a or pK_b of HA. Thus, pH may be manipulated to “push” the acid into the neutral form (HA) to extract the acid into the organic extracting solvent.

The more complicated example is the case of ionic salts, which must account for the aqueous-organic partition of both the nondissociated salt and the chelated ions, as well as the dissociation of the salt to its component ions and the equilibrium for the chelation of those ions. Although this situation may be somewhat more complex, its straightforward treatment is common in most analytical chemistry textbooks. Of course, the most complex situation is the real world, as these are mixtures containing more than one analyte type. Hence, assumptions and simplifications are often made in the use of partition coefficients.

Measurement of Octanol‑Water Partition Coefficients

For this section, we will focus on the laboratory measurement of K_ow, perhaps the most widely used and applicable partition coefficient in analytical chemistry. The definition of octanol-water partition coefficients indicates that these values can be determined by calculating a simple ratio of the analyte solubility in n-octanol to its solubility in water. However, we must keep in mind that this value is based on an equilibrium, so we must keep in mind the mutual solubility of the two solvents in each other. For example, water is quite soluble in n-octanol, up to around 20%.

Table 1 summarizes the laboratory methods for the determination of K_ow endorsed by the Organization of Economic Cooperation and Development (OECD) (1,2). Of these, the shake-flask methods are the simplest and most reliable, though time-consuming.

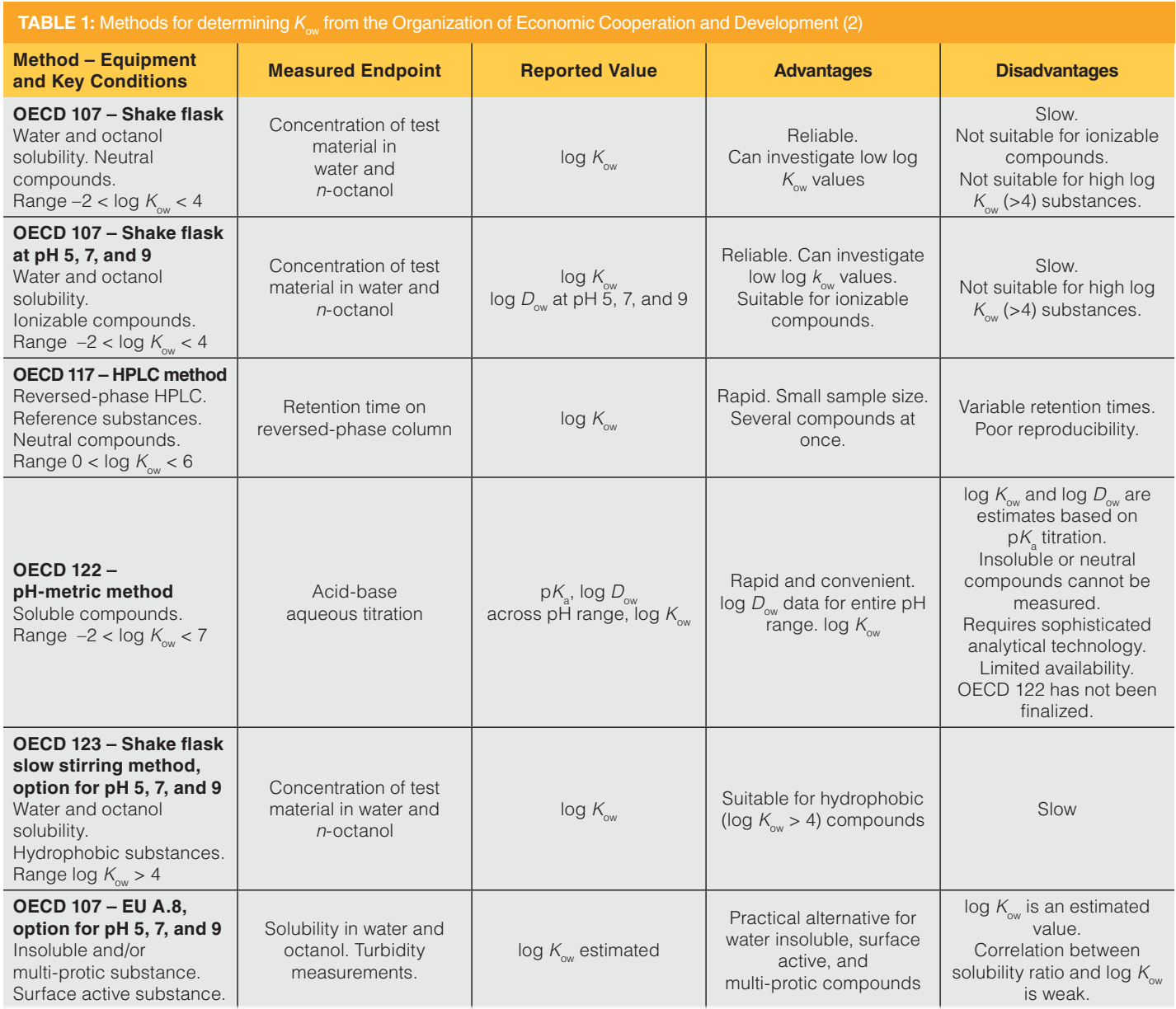
Related to the shake-flask method is a derivation called the slow-stir method, with the major difference being the glassware used and mode of agitation (phase contact and equilibrium). Following the shake-flask method, solute concentrations may be measured by any applicable analytical technique, commonly chromatography, optical spectroscopy, or proton-nuclear magnetic resonance spectroscopy. Reversed-phase liquid chromatography (RPLC) is used to determine K_ow values with the key assumption being that the stationary phase, generally octyl- or octadecyl-, mimics n-octanol. RPLC is mostly applicable to ionic samples.

Conclusions

At its most fundamental level, chemical separations, both analytical and industrial scales, are based on the concepts of equilibrium and diffusion. Regarding equilibria, the equilibrium constants describing partitioning and other phase distribution processes are vital, including consideration of solute dissociation. To simply this confusing multitude of terms, simple definitions, applications of partition coefficients to chromatographic sample preparation (such as extractions), and a summary of how to measure partition coefficients are given in this article.

References

(1) Peters, D. G.; Hayes, J. M.; Hieftje, G. M. Chemical Separations and Measurements: Theory and Practice of Analytical Chemistry; W. B. Saunders Co., 1974; pp. 468–469.

(2) Ecetoc, Technical Report 123. https://www.ecetoc.org/technical-report-123/appendix-b-measurement-of-partitioning-kow/ (accessed 2023-05-09).

About the Column Editor