Chromatography Fundamentals, Part VII: Influence of Peak Broadening on Detection Sensitivity, Solute Dilution, and Efficiency of Coupled Columns

In this contribution, we examine the degree to which injected samples become diluted during chromatographic elution, reducing detection sensitivity. Equations are also introduced for estimating the plate count of column sets that comprise different column lengths and efficiencies.

The purpose of this tutorial is to present equations for estimating the extent of broadening that a solute undergoes as it migrates through a chromatographic column in high performance liquid chromatography (HPLC). These relationships can then be used for predicting the degree of solute dilution that in turn will influence detection sensitivity, as well as the effectiveness of semipreparative HPLC. Furthermore, equations are derived for estimating the number of theoretical plates generated when joining columns of different efficiencies and lengths.

Background

Peak broadening is a complex process involving the resistance of mass transfer and molecular diffusion of solutes, coupled with hydrodynamic-flow properties of the mobile phase as it permeates through the packed bed. As a result of these mechanisms, solutes emerge from the column as broadened peaks that take the form of a Gaussian distribution (1,2).

Chromatographic peaks are characterized by a mean and standard deviation, σ, better known as the peak-maximum retention time, t_r, and peak width, w, such that w = 4σ. Although 4σ includes only 95.6% of the peak area, it is, nevertheless, a good approximation, considering that chromatographic peaks are usually slightly tailed or asymmetric.

The number of theoretical plates, N, is the figure of merit that defines column efficiency as

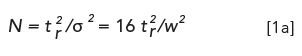
where t_r is the retention time of a test solute; typically, but not necessarily, an unretained solute. Since retained components include additional peak broadening caused by the stationary phase, plate count measured in this manner is significantly lower than with an unrestrained solute. Because the units of retention time and peak width are the same, N is a dimensionless property of a column measured at specific conditions. Please note that the accepted definition of theoretical plates is now equation 1b, where w_0.5 is peak width at half height.

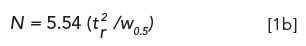
To a first approximation, the number of theoretical plates generated by a column is defined by the column packing, most notably particle size, shape, and particle-size distribution. This value, however, can be increased to some extent by decreasing the flow rate, reducing injection volume, or increasing column temperature.

The impact that peak broadening has on separations cannot be overstated. To fully appreciate its importance, it is instructive to compare hypothetical chromatograms with and without broadening. In the absence of peak dispersion, components would be eluted as razor-sharp, rectangular-shaped peaks, each with a peak volume corresponding to the injection volume. Thus, a 5-µL injection at a flow rate of 0.5 mL/min would produce geometrically shaped peaks with 0.15-s peak widths. Under these conditions, a peak that is retained for 10 min, for example, would generate roughly 1.6 × 10⁷ plates, as compared to about 10⁴ plates with normal broadening with a high-efficiency column. With these extraordinarily high numbers of theoretical plates, it would be feasible to separate compounds based on subtle chemical or structural differences.

Influence of Peak Broadening on Solute Concentration

The Gaussian distribution function,

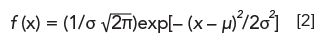
forms the basis of chromatographic theory (1,2), where the pre-exponential term, (1/(σ√2π), is a normalization factor that ensures that all peaks have an area of unity. The first moment of the distribution, µ, is the peak-maximum retention time of a Gaussian-shaped peak, and x is the retention time with a corresponding peak height, f(x) = y. As peaks broaden, f(x) or peak height decreases at each elution-volume increment along the peak.

With the use of equation 2, we can derive the following relationship:

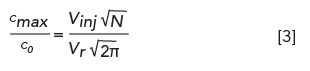
Here, c_max/c₀ is the ratio of the solute concentration at peak maximum, c_max, relative to the initial injected concentration, c₀, V_inj is the injection volume, and V_r is the retention volume. The ratio, c_max/c₀, is also equal to the dilution factor of an eluting solute.

Equation 3 is useful for monitoring the extent of solute dilution, especially for semipreparative fractionation; if collected fractions are too dilute, a concentration step may be required before proceeding to the next step. This equation tells us that solute dilution is proportional to retention. In other words, the greater the peak broadening, the more dilute the solute becomes, as would be expected.

Example calculations are given in Table I, which shows the effects of column efficiency and injection volume on sample dilution. Plates per column range from 1000 to 30,000, with injection volume ranging from 5 to 40 µL; these data do not take into account efficiency loss with increasing injection volume, which would further increase sample dilution.

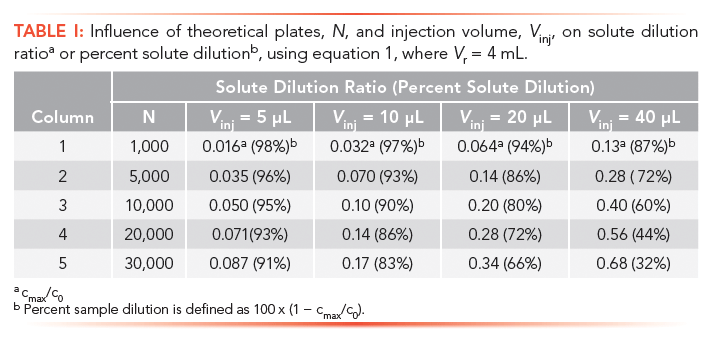
It is interesting to note the high degree of solute dilution that occurs even when injecting a small volume with respect to the elution volume of the solute. With a low-efficiency of 1000 plates, an injection of 5 µL would result in 98% dilution; at these same experimental conditions, a high-efficiency 30,000-plate column would dilute the solute by 91%. However, with increased injection volume, and by keeping elution volume constant, solute dilution decreases. For example, a 40-µL injection using a 1000-plate column, would result in 87% dilution, as compared to 32% for the 30,000-plate column. (For semipreparative separations, the injection volume should be as large as possible. )

Equation 3 is applicable only for isocratic elution; with gradients, peaks can be compressed, depending upon the gradient program, and analytes are eluted in a more concentrated form.

Effect of Peak Broadening on Detection Sensitivity

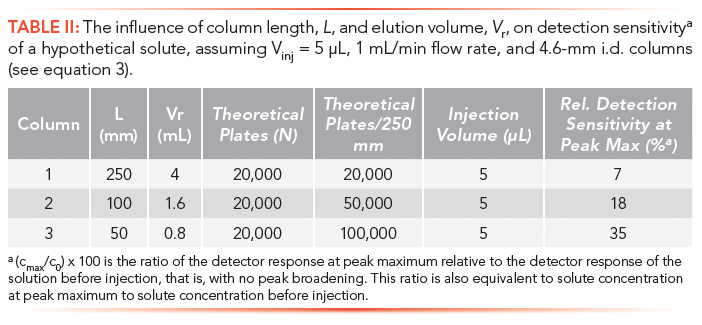
Equation 3 can also be used for predicting relative detection sensitivity, an important HPLC parameter, which is defined as the slope of detector response of a solute versus injection concentration, a value used for calculating detection limits. As discussed in the previous section, peak broadening has a pronounced effect on detection limits, influencing both accuracy and reproducibility. Thus, another consequence of peak broadening is decreased peak height at peak maximum, characterized by the ratio c_max/c₀.

In Table II, we define detection sensitivity as the relative percentage of detector response: (c_max/c₀) x 100. This value is calculated for three hypothetical columns that have the same plate number (20,000), but different lengths: 250, 100, and 50-mm, packed with decreasing particle size. The injection volume is kept constant at 5 µL. As shown in Table II and in equation 3, the elution volume of a given solute decreases with decreasing column length, and the relative detection sensitivity increases with decreasing column length. For example, the detection sensitivity of a 250-mm column is only 7%, but increases to 35% for a 50-mm column of corresponding efficiency. The reason for this trend is that c_max/c₀ scales with V_inj/V_r at constant plate count. From a practical consideration, a relative detection sensitivity of about 10% should be adequate for most applications, except for trace analysis, where a higher ratio would be required to overcome baseline noise.

Keeping the same LC conditions and column dimensions, we can also estimate the required number of theoretical plates, N₂, to obtain a given, relative detector response (c_max)₂, using the following ratio:

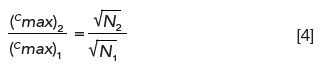
For example, increasing plates from 2000 to 10,000 would more than double detector response for a given method.

Equation 3 can be rearranged to estimate the injection volume V_inj needed to obtain a given c_max/c₀ ratio, for a solute with retention volume V_r and number of plates,

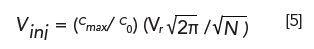
For example, an injection volume of 50 µL would be needed to obtain a relative detection sensitivity of 0.5 using a 10,000-plate column for a solute with a 4-mL retention volume. For a 12-mL retention-volume peak, a 150 µL injection would be required to obtain a comparable detector response.

An interesting relationship is obtained by expressing equation 3 in terms of the peak standard deviation, σ_v, by substituting

into equation 3, and canceling out retention volume to give

Although equations 3 and 7 are equivalent, we can obtain insight into the separation process by examining results from both. Such an application is given in Table III, which shows the influence of theoretical plates on peak width (w = 4σ_v ), and relative detector response of a 10-µL injection with a 4-mL peak retention volume. With a conventional, low-efficiency column of 400 plates (column 1), there would be considerable peak broadening: The initial 10-µL injected volume would spread by a factor of 80x to about 800 µL, with a relative detection sensitivity of only 2%. Detection limit can be improved by a factor of fivefold with a 10,000-plate column (column 4). To achieve a peak width of only 16 µL, we would have to generate close to one million plates (column 7). With modern HPLC columns, we typically can keep the relative detection sensitivity to about 8–20%.

Coupling Columns

A practical approach for increasing plate counts is to simply couple together columns of the same packing that have equivalent lengths and theoretical plates. The total plate count of a multiple-column set consisting of columns that have the same length is simply the sum of the theoretical plates of the individual columns,

The number of theoretical plates can correspond to either an unretained or retained compound, depending on the situation, but it must be either one or the other. The reason why we add theoretical plates, rather than variances (2), is that variance and plate counts are interrelated through σ² = t_r² /N.

Difficulty arises when coupling columns of different lengths or plate counts, in which case equation 8 is no longer valid. When a solute leaves a highly efficient column (column 1) and enters a second column (column 2) of lower efficiency, the peak will broaden to a greater extent. Conversely, if column 2 has higher efficiency than column 1 (such as when adding a precolumn to a column), the peak cannot reverse course, but would continue to broaden. In other words, once a peak broadens, it cannot be reversed or remedied by entering a column of higher efficiency. (We can, however, concentrate the solute onto the head of a second column by decreasing eluent strength, but this involves employing a different strategy not considered in this paper.)

For a single column, the number of theoretical plates, using either an unretained or retained component with units of time, is calculated with

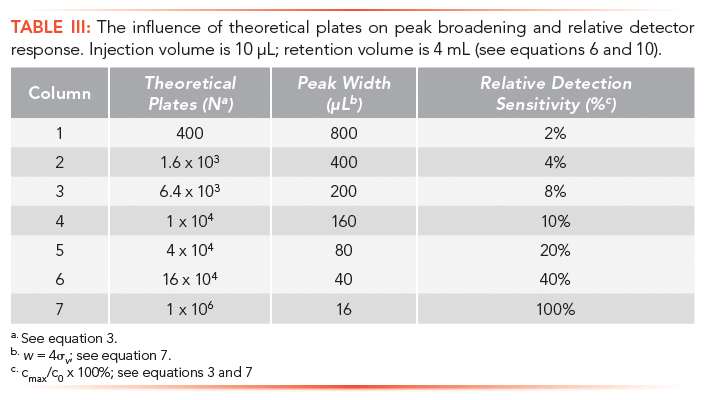
where t is elution time, and σ_t² is the peak variance of the corresponding peak in units of time. For column sets comprising columns with different lengths or efficiencies, the total plate count of either an unretained or retained peak is

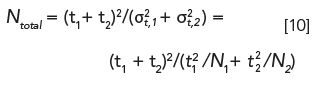
where subscripts 1 and 2 refer to the two columns. The general equation for calculating the total plate count of an unretained or retained peak of a bank of n columns is therefore

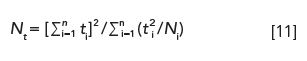
Likewise, for a single column, the number of theoretical plates of an unretained component using column length as the unit of measure is

where L is column length and σ_L² is the peak variance of an unretained peak in units of length. For a coupled column comprising two columns, the total plate count of an unretained peak is

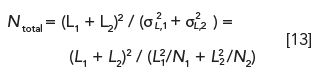
where subscripts 1 and 2 refer to the two separate columns. The general equation for calculating the total plate count of an unretained peak of a bank of n columns is

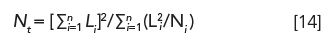
The total number of theoretical plates of column sets, like those seen in equations 11 and 14, is a weighted average, not an arithmetic sum. Note that the efficiency of a bank of columns is independent of column sequence; similar results would be obtained if the column order were to be reversed. Furthermore, consider the mathematical order of the numerators in equations 11 and 14; column lengths or retention times are first added together, and then squared, while in the denominators, time or lengths are first squared, then divided by their corresponding plates, and finally added together.

A practical application of these concepts would be attaching a well-used 50-mm precolumn with 500 plates to a new 250-mm analytical column with 30,000 plates. According to equation 11, the total estimated plate count of the 300-mm column set would be about 13,000, representing a 60% efficiency loss.

Conclusions

When an injected sample undergoes peak broadening, the concentrations of eluted components are greatly reduced. Based on a Gaussian distribution model, relationships are presented that show that the degree of solute dilution is proportional to retention volume, and inversely proportional to injection volume and the square-root of column plate count. Solute dilution not only reduces the efficacy of analytical and semipreparative HPLC fractionation, but also lowers detection sensitivity.

A convenient approach for increasing column efficiency is simply to couple columns together. As a result of peak broadening, however, a weighted-average plate count, rather than an arithmetic mean, must be used to calculate the total plate count of column sets that consist of different length or efficiency columns. Calculations show that connecting a short, but low-plate, count column in series with a high-plate analytical column has a deleterious effect on the total efficiency of the column bank.

Our next contribution will discuss the impact that peak broadening has on chromatographic resolution.

Dedication

This paper is dedicated to Prof. Barry L. Karger, Director Emeritus of the Barnett Institute, and the James L. Waters Chair and Distinguished Professor Emeritus at Northeastern University, in honor of his 80th birthday.

References

(1) H.G. Barth, LCGC North Am. 36(11), 830–835 (2018).

(2) H.G. Barth, LCGC North Am. 37(4), 269–273 (2019).

Howard G. Barth is with Analytical Chemistry Consultants, Ltd., in Wilmington, Delaware. Direct correspondence to: howardbarth@gmail.com