Measurement of Interstitial Space Dispersion in Packed Bed Columns: Comparison of Superficially Porous and Fully Porous Particles

The difference in packing quality of superficially porous and fully porous columns has been measured using the total pore blocking technique. This technique physically eliminates all mass transfer band broadening contributions, and hence provides the purest possible measure of the packing quality of a column. The measurements confirm earlier assertions made in the literature about the generally better packing quality of superficially porous columns over fully porous particle based columns.  

Producing reduced plate height curve minima that are typically on the order of 0.5 units lower than possible with fully porous particles (FPPs), the (re-)introduction of superficially porous particles (SPPs) around the year 2007 has absolutely revolutionized the efficiency of HPLC columns (1–6). Part of this higher efficiency (roughly some 40 to 50%) can be attributed to the fact that SPPs exhibit a lower longitudinal diffusion band broadening (presence of the core partially blocks the longitudinal diffusion, and most SPPs have a lower internal porosity, that also tends to slow down diffusion) as well as a lower stationary phase mass transfer resistance (presence of the core minimizes internal diffusion distances). The latter, however, typically accounts for some 5 to 10% of the observed gain, which is considerably smaller than claimed in the advertisements of some SPP manufacturers.

The remaining 40 to 55% of the gain, therefore, necessarily needs to be attributed to the fact that SPP columns display a significantly lower eddy-dispersion (so-called A-term band broadening, describing the packing heterogeneity). The reason underlying this prior, unexpected effect is a problem that has not been fully resolved up to now. There have been speculations about differences in surface roughness, leading to differences in packing quality (3). Another proposed explanation was that SPPs have a markedly narrower particle size distribution than FP particles, which can, in turn, be expected to lead to more uniform packings. This explanation has also been the subject of considerable controversy (3,7,8). Nevertheless, the fact that the introduction of FPPs with a narrower PSD also lead to a marked decrease in h-values has now recently brought new evidence to support this hypothesis (9).

Traditionally, the eddy-dispersion is determined by subtracting known (or estimated) values of the longitudinal diffusion and the mobile and stationary phase mass transfer resistances (so-called C_m- and C_s- term; see equations 2 and 3) from the measured plate height. However, the model for the mobile phase mass transfer resistance is still under debate, and the observed eddy-dispersion plate heights tend to depend on the retention factor and the intraparticle dispersion of the analytes, complicating the direct comparison of SPP and FPP columns.

In the present contribution, the mass transfer resistance contributions are physically switched off using the so-called total pore blocking (TPB) technique to render the mesoporous space of the particles completely inaccessible for the injected analytes (10,11). As a consequence, the mass transfer processes are blocked, and the only remaining source of band broadening (apart from the longitudinal diffusion) is the heterogeneity of the interstitial space.

In brief, the TPB method works as follows: First, the column is flushed with a solvent, such as isopropanol, that is fully miscible with both hydrophobic and hydrophilic liquids. Subsequently, the column is filled with a strong hydrophobic solvent, such as decane, which can fully replace the isopropanol in both the interstitial space as well as in the particle mesopores (Figure 1a). Next, the decane is pushed out of the interstitial space again, using a hydrophilic buffer that is immiscible with the decane. Due to the high affinity of the decane for the C18 layer inside the mesopores, the decane cannot be removed from the particle's interior (Figure 1b). Injecting now a strongly hydrophilic marker (such as potassium iodide), this marker will not be able to penetrate the particles, mimicking (at least for the marker) a completely nonporous column.

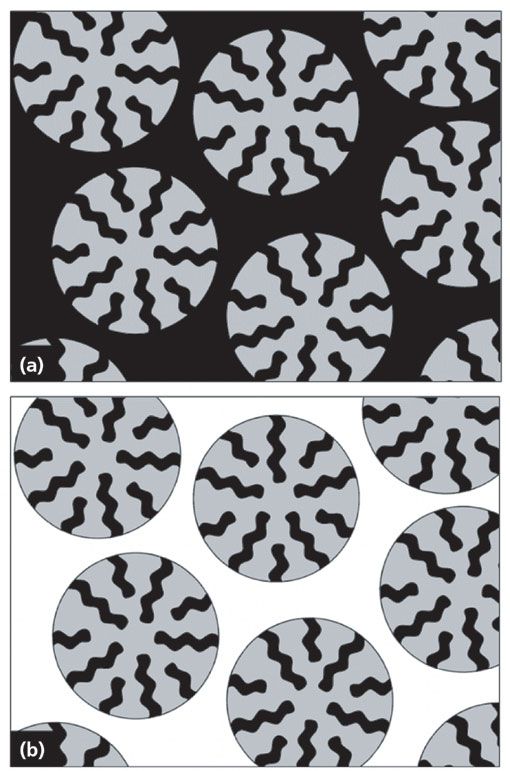
Figure 1: Schematic representation of the total pore blocking principle showing the column composition (a) after the column is completely filled with decane, and (b) after all the decane is chased out of the interstitial space by flushing the column with an immiscible hydrophilic buffer.

The TPB technique was originally introduced to make accurate measurements of the external porosity (ε) of packed columns (10,11). Conventionally, this is measured using so-called inverse size exclusion chromatography (ISEC). Whereas the polystyrene standards used in ISEC have difficulties accessing the smallest spaces of the interstitial volume, and thus require an extrapolation to assess the full extent of the interstitial space, the TPB method works with small molecule tracers that can explore the entire space.

Since its introduction, TPB has been used frequently by others (9,12–16). Here, we report on the TPB measurement to investigate differences in interstitial space dispersion between FPPs and SPPs. This was done on a set of 4.6 mm × 150 mm columns packed with 5 µm particles. These dimensions were purposely selected to minimize contributions from extracolumn sources, because the peaks under TPB conditions are eluted at zero retention (as a matter of fact, they are even eluted before the t₀-marker of the unblocked column), and therefore are very narrow.

Experimental

All experimental work was performed on an Agilent 1290 Infinity UHPLC system with a 1260 DAD, equipped with an 80 or 500 nL detector cell (Agilent Technologies). All columns used in this study were 4.6 mm × 150 mm columns, packed with either FPPs or SPPs with a diameter of 5 µm and C18-derivatized. Excel (FP_A) and Ultracore (SP_A) columns (ACE), as well as Luna (FP_B) and Kinetex (SP_B) columns (Phenomenex) were compared. Van Deemter measurements on the unblocked columns were performed at flowrates ranging from 0.05 to 2 mL/min, with a mobile phase of 50 v/v% (Ultracore) or 49 v/v% (Excel) acetonitrile in water, to obtain the same retention factor for butyrophenone. A mixture of potassium iodide, acetophenone, propiophenone, and butyrophenone (Sigma-Aldrich), all dissolved to a concentration of 100 µg/mL in the mobile phase solvent, was used as the sample to study the column under retained (open-pore) conditions. In order to block the columns, they were flushed with IPA (Biosolve B.V.) at a flowrate of 0.2 mL/min for 60 min, subsequently filled with decane (Acros Organics) at a flowrate of 0.2 mL/min for approximately 100 column volumes, and finally flushed with a 10 mM ammonium acetate (Sigma-Aldrich) buffer (pH = 3) at a flowrate of 0.4 mL/min (10). Retention times under blocked pore conditions were measured by injecting 500 µg/mL of potassium iodide dissolved in the buffer every 5 min during this final flushing step. The same buffer and potassium iodide sample were used to measure the van Deemter curves on the blocked columns. All measurements were performed at 30 °C, at a wavelength of 254 nm and a frequency of 40 Hz, and the injection volume was set to 1 µL. Peak widths were measured at half height and 4.4% height (5σ).

Reduced van Deemter plots were calculated using the nominal value of the particle size as mentioned by the manufacturer (h = H/d_p). The calculated values of the true particle size were in very good agreement with the values provided by the vendor for the particle batches. The particle size estimated based on the experimentally measured permeability and porosity measured on the different columns varied at most 10% from their nominal size. The columns of the same type from the same vendor had a difference in particle size less than 3%, based on the experimentally measured permeability and porosity. Furthermore, since only a relative comparison of column performance between columns of the same vendors are discussed, the true value of the particle has no impact on the conclusions. For the diffusion coefficients, experimentally measured values reported in (17) were used, for the given component and employed mobile phase composition (ν_i = u_i·d_p/D_m), with D_m equal to 1.2 × 10^-9m²/s for acetophenone, and 2.1 × 10^-9m²/s for potassium iodide.

Results and Discussion

Unblocked Pore Dispersion

First, the columns were tested under retained component conditions (with normally accessible mesopores). The resulting reduced van Deemter curves for acetophenone are shown in Figure 2a. The curves display the typically observed difference between both particle types: Whereas the FPP column produces a minimal reduced plate height of h_min = 2.1 (indicative of a well-packed column [18]), the SPP column produces a significantly lower minimum (h_min = 1.2). These minima are obtained at an optimal velocity around α_opt = 9 for both the FPP and the SPP column. A similar difference (on the order of 0.8 to 1 reduced plate height units) is observed for the other, more strongly retained test analytes (data not shown). For the stronger retained compounds, the absolute h_min values are also shifted to a larger value because of the larger B-term contribution typically observed for components with a higher k. For the same reason, the optimal velocity is shifted to a somewhat larger value with increasing retention.

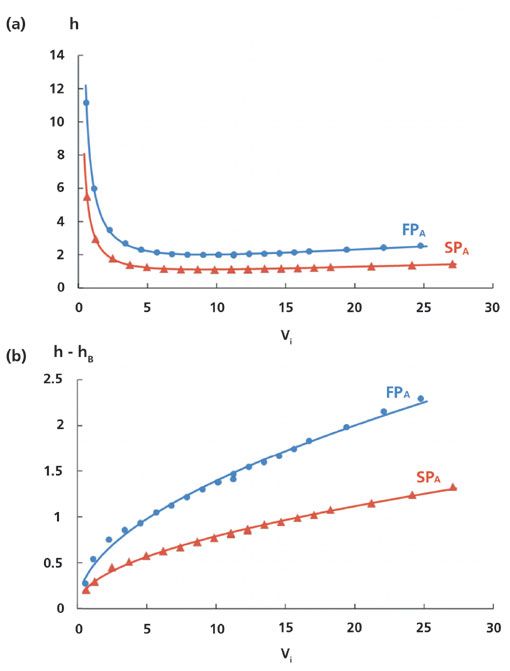
Figure 2: (a) Reduced plate height plot, and (b) B-term subtracted reduced plate height plot for acetophenone (k' = 1.6) on the FP_A and SP_A columns before blocking. Curve fits according to equation 1. FP_A: blue dots and SP_A: red triangles.

The data were fitted using the free-exponent Knox-model (19), producing a good fit (R² = 0.98–0.999):

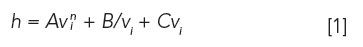
This model has been preferred over the more frequently used fixed-exponent Knox model (where n is fixed at n = 0.333). The original data set on which the classic n = 0.333 value was based was rather limited, while later work showed the exponent itself contains relevant information on the packing and can vary considerably (cf. range between n = 0.5 and n = 1 in [19]). The resulting fitting parameters are given in Table I. During the fitting, the C-term constant was fixed at the C_s constant value found from equation 2, discussed further on. In agreement with its larger overall h values, the A-, B-, and C-term constants of the FPP column are clearly higher than those found for the SPP column. The n-exponent (order of n = 0.45 to n = 0.5) is typical for the behavior of a retained analyte in a packed bed column (19).

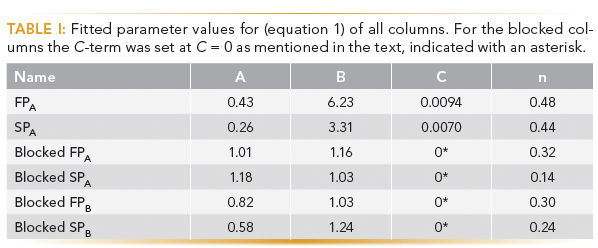
Table I: Fitted parameter values for (equation 1) of all columns. For the blocked columns the C-term was set at C = 0 as mentioned in the text, indicated with an asterisk.

Figure 2b shows what remains of the observed band broadening when subtracting the contribution of the longitudinal diffusion. Because of the subtraction, all curves now tend to zero when ν_i tends to zero. As can be noted, the difference between the SPP and the FPP column is about 0.57 reduced plate height units at the ν_i = 9 (h minimum of the two particle types in Figure 2a). This shows that roughly 35% of the difference between the FPP and the SPP column can be attributed to the difference in the B-term. As explained in (20–22), the significantly lower B-term band broadening of superficially porous particles is due to both the presence of the core as well as that the fabrication processes of the majority of the vendors leads to a mesoporous shell layer with a relatively low internal porosity.

Using the fitted value of the B-term to deduce the diffusion coefficient (D_pz) inside the mesoporous zone of the particles using the effective medium theory (20), we respectively obtained a value of D_pz= 7.8 × 10^-10 m²/s (fully porous) and D_pz= 4.2 × 10^-10m²/s (superficially porous). These values indicate the true transport rate inside the mesoporous material of the SPPs is significantly smaller (close to a factor of 2) than in the FPPs This has been observed in the past (20,23), and has been explained by differences in synthesis procedures and a concomitant difference in internal porosity. With these values, we can now calculate the expected contribution of the intraparticle mass transfer resistance (h_Cs) (so-called C_s-term band broadening):

with α, the shape factor, equal to 6 for packed bed spheres, k" the zone retention factor (k" = (1 + k') (ε_T/ε) - 1), εand ε_T the external and total porosity, respectively, and Sh_part given by equation (T-35) in Andrés and associates (24).

From equation 2, it can be calculated that the difference in h_Cs around the optimal velocities (ν_i = 9) is only on the order of about 0.025 reduced plate height units. (only about 3% of the difference in h_min observed in Figure 2a can be attributed to the presence of the core). Obviously, this effect is much smaller than the initial claims made by some manufacturers.

A similar exercise can be done to estimate the expected mobile zone mass transfer resistance (h_Cm) (so-called C_m-term band broadening):

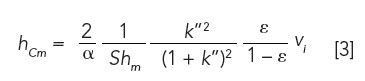
with Sh_m given by equation 11 in Deridder and associates (25). With the known k"-value(s), and using α = 6 for spherical particles and the ε-values determined below (see discussion of Figure 3), it can be calculated that the difference in h_Cm around ν_i = 9 is on the order of about 0.02 reduced plate height units, corresponding to some 2% of the difference observed in Figure 2a.

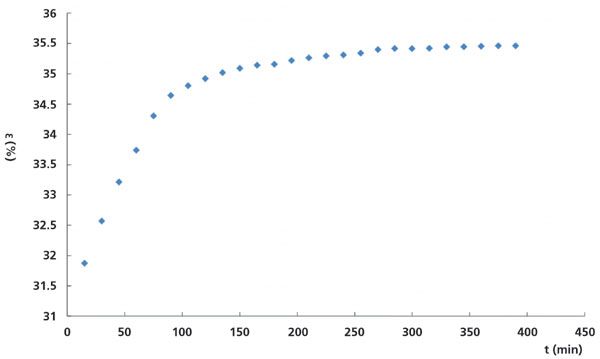
Figure 3: Plot of the external porosity versus time during the flushing step of the total pore blocking method of the SP_B column.

Blocked Pore Dispersion

The above analysis implies the remaining difference must be due to differences in eddy-dispersion (such as due to differences in packing quality and interstitial space heterogeneity). To investigate and measure this difference in the absence of any intraparticle contribution (to have the purest possible measure of the dispersion in the interstitial space), the columns were subsequently blocked following the procedure established in (10). During the flushing step needed to make the gradual transition between condition 1 and condition 2 in Figure 1, the relative retention volume of the KI marker (F·t_i/V_G = ε with V_G the geometrical volume of the open column) is continuously monitored until this value reaches its plateau value (see Figure 3, for example). Reaching the plateau is indicative of the state (Figure 1b) wherein the interstitial space is completely cleared of the decane originally occupying it (Figure 1a). This event is typically also marked by the fact that the detector trace becomes flat and stable again, after a period of strong disturbances caused by small amounts of decane passing the detector.

A final control to verify whether the entire interstitial space is cleared of decane is made by verifying that the pressure needed to send a given flow rate F through the column in the blocked state is equal to that required in the unblocked state. If this is the case, it can be guaranteed the interstitial space is in both cases the same (10). This can be understood by writing the well-known Kozeny-Carman equation in the following form (26), showing that, for a given F, the measured pressure drop only depends on the external porosity, and is independent of the intraparticle porosity:

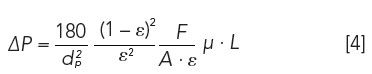
wherein d_p is the particle diameter, ε the external porosity, F the flow rate, A the cross-sectional area, µ the viscosity, and L the column length.

If part of the interstitial space were still occupied by a remaining fraction of decane, this would reduce the accessible interstitial space, and the mobile phase flow would experience a smaller external porosity ε. As can be noted from equation 4, showing a strong dependency between ΔP and ε (close to a ε^-5 dependency), even a small decrease in ε can already be expected to lead to a significant increase of the required inlet pressure. Figure 4 shows one of the pressure tests conducted in the plateau phase of Figure 3 to verify this. As can be noted, the measured pressures before and after blocking the column all lie close to the bisecting line, thus indicating the above criterion is satisfied, and indicating the interstitial space under blocked conditions is indeed identical to the actual interstitial space observed in the open-pore conditions.

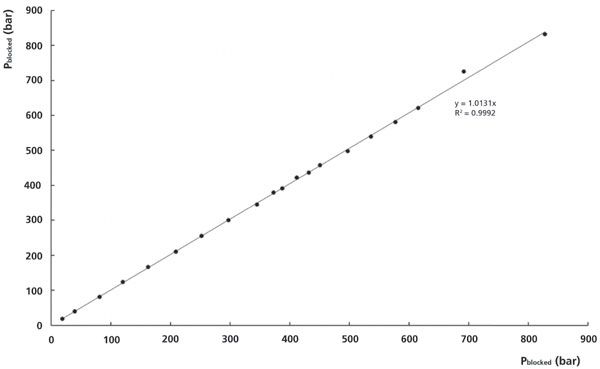
Figure 4: Plots of the measured pressures after blocking the column versus the measured pressures before blocking of the column for the SP_A column.

Figure 5 shows some of the potassium iodide tracer peaks eluting from the column in the plateau phase, such as under perfect blocking conditions. The peaks eluting from the FPP column are clearly broader than those eluting from the SPP column over the entire range of different flow rates, indicating the former suffers from a significantly larger packing heterogeneity than the latter. The difference also clearly increases with increasing flow rate. This is mainly due to the fact that, at the lower flow rates, a significant part of the band broadening is due to longitudinal diffusion, which is to a very large extent is independent of the degree of packing heterogeneity (see also small differences between B-term values in Table I).

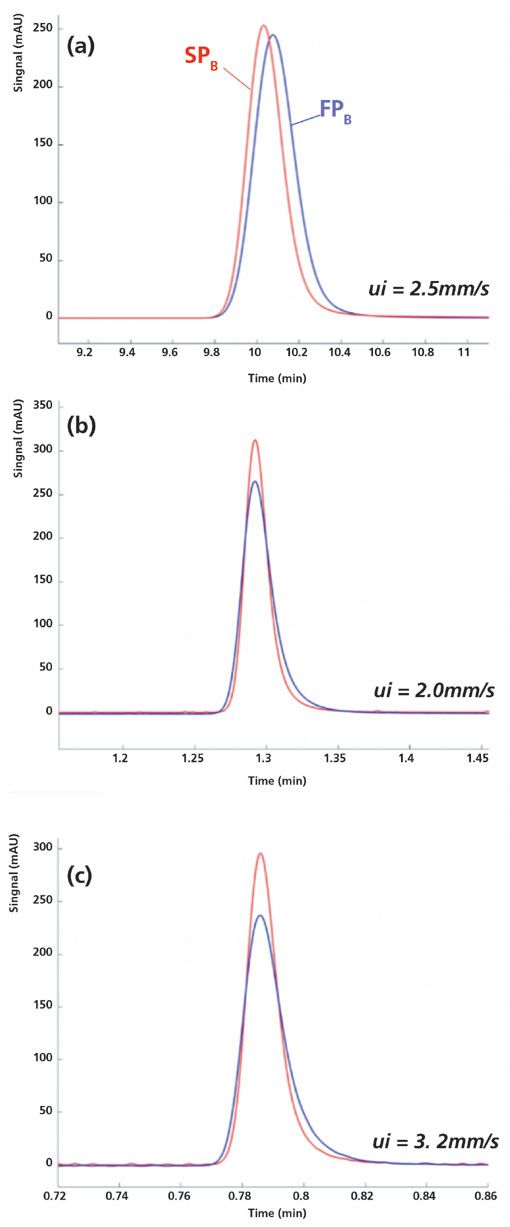
Figure 5: Overlay of the chromatogram peaks for KI of the blocked columns at three different interstitial velocities (a) u_i = 0.25 mm/s, (b) u_i = 2.0 mm/s, and (c) u_i = 3.2 mm/s. Blocked FP_B in blue and blocked SP_B in red.

The observations in Figure 5 are more quantitatively and comprehensively represented in the reduced van Deemter plots (h_TPB versus υ_i) in Figure 6. These show a clear difference (of around 0.2 to 0.3 reduced plate height units) between the FPP and the SPP column also shown in Figure 2. The contribution of the extra-column dispersion can be estimated to be on the order of 5 µL²; for example, about 5% at υ_i = υ_opt, and around 8% at the highest υ_i. The curves appears to cross for low υ_i, but this should not be emphasized too much, as it was recently shown that even small errors on the B-term constant result in large deviations in the estimated h-h_B values at low velocity (υ_i< 2) (27).

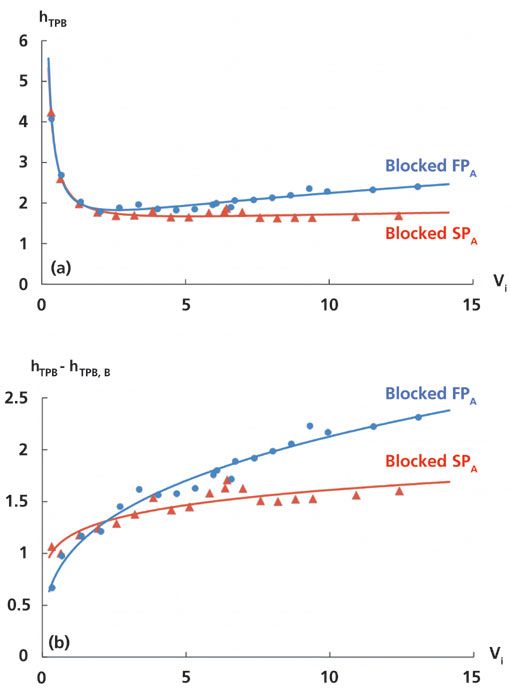
Figure 6: (a) Reduced plate height plot, and (b) B-term subtracted reduced plate height plot for Kovats index on the FP_A and SP_A columns after blocking. Curve fits according to equation 1. Blocked FP_A: blue dots and blocked SP_A: red triangles.

An important observation from Figure 6 is that the h_TPB-values are of the same order (and even larger in the SPP case) than the h-values observed in Figure 2a. This implies the dispersion measured using the total pore blocking technique cannot simply be considered as a measurement of the eddy-dispersion contribution prevailing under normal open-pore conditions; therefore, it cannot be seen as a measurement of the first term of equation 1. This lack of correspondence is due to the fact that the total pore blocking technique is measuring the dispersion under different boundary conditions at the particle outer surface than in the open-pore case (no diffusion towards and through the particles in blocked pore case, equal diffusion fluxes and chemical potentials in the open-pore case). However, this does not imply the measured differences under total pore blocking conditions are not relevant. On the contrary, it is precisely the absence of any interference with the intraparticle and transparticle diffusion that makes the h_TPB-values the purest possible measurement of the packing homogeneity of a column.

The same comparison was also conducted for another set of FPP and SPP particle columns from a different vendor. In this case, two columns were tested per particle type and the curves represented in Figure 7 show the average of the two columns.

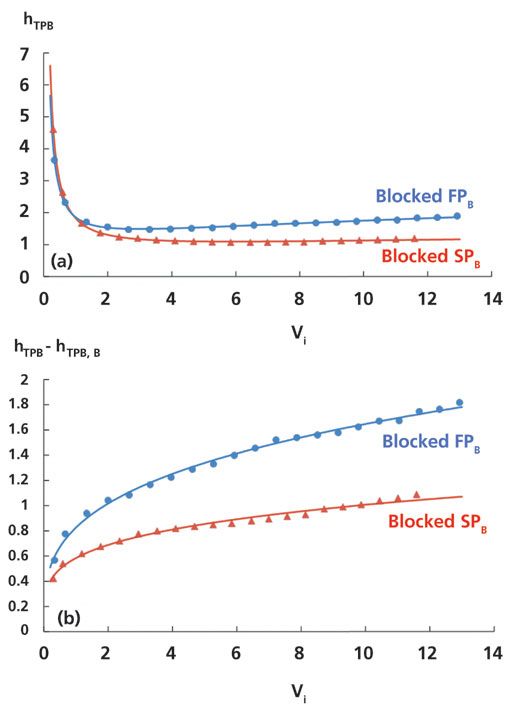
Figure 7: (a) Reduced plate height plot, and (b) B-term subtracted plate height plot for Kovats index on the FP_B and SP_B columns after blocking. Curve fits according to equation 1. Blocked FP_B: blue dots and blocked SP_B: red triangles.

The h_TPB-curves in Figures 7a and 7b have also been fitted with equation 1. This was done by keeping the C-term constant at C = 0 to represent the absence of any mass transfer to and in the particles. One interesting observation from the fitting parameters shown in Table I is that the difference between the B-term constants of the SPP and FPP particles has dropped below the significance level. This is again in agreement with the fact that longitudinal diffusion is known to be virtually independent of the packing heterogeneity. The actual B-values are somewhat smaller than the theoretically expected value around 1.5, but this can be attributed to the lack of a sufficient number of data points in the low υ_i-range. Another observation from Table I is that the n-values are significantly smaller in the blocked pore conditions than in the open pore conditions. A lower n indicates a larger contribution of velocity biases that are terminated by changes in the velocity field compared to those terminated by lateral diffusion. This makes perfect sense, given the blocked particles are now reducing the possibilities for transparticle diffusion equilibration.

Conclusions

The total pore blocking technique can be used to measure the interstitial space dispersion in the absence of any inter-, intra- or transparticle mass transfer contribution. Subsequently also removing the B-term contribution, the plots in Figures 6b and 7b clearly show that fully porous particle columns tend to have a higher interstitial space dispersion (for example, packing heterogeneity) than superficially porous particle columns. This was confirmed for two different vendors. The difference in dispersion, expressed in reduced plate height units, increases with increasing velocity. Around the minimum of the retained component van Deemter curve (υ_opt = 9), the difference is on the order of 0.6 to 0.8 reduced plate height units, depending on the column manufacturer.

Given the very small peak volumes eluted under pore blocking conditions, the measurements are limited to columns with a large volume packed with large particles. The latter is also needed to prevent high pressures that would make the blocking agent leak or contract.

Acknowledgments

The authors would like to thank Advanced Chromatography Technologies Ltd (ACE), Achrom and Phenomenex for the gift of the columns used in this study.

References

(1) S. Fekete, E. Oláh, and J. Fekete, J. Chromatogr. A 1228, 57–71 (2012).

(2) R. Hayes, A. Ahmed, T. Edge, and H. Zhang, J. Chromatogr. A 1357, 36–52 (2014).

(3) G. Guiochon, and F. Gritti, J. Chromatogr. A 1218, 1915–1938 (2011).

(4) Y. Vanderheyden, D. Cabooter, G. Desmet, and K. Broeckhoven, J. Chromatogr. A 1312, 80–86 (2013).

(5) D.V. McCalley, J. Chromatogr. A 1193, 85–91 (2008).

(6) J.J. Kirkland, T.J. Langlois and J.J. DeStefano, Am. Lab. 39, 18–21 (2007).

(7) D. Cabooter, A. Fanigliulo, G. Bellazzi, B. Allieri, A. Rottigni, and G. Desmet, J. Chromatogr. A 1217, 70–74 (2010).

(8) F. Gritti, D.S. Bell and G. Guiochon, J. Chromatogr. A 1355, 179–192 (2014).

(9) O.H.Ismail, M. Catani, L. Pasti, A. Cavazzini, A. Ciogli, C. Villani, D. Kotoni, F. Gasparrini, and D.S. Bell, J. Chromatogr. A 1454, 86–92 (2016).

(10) D. Cabooter, F. Lynen, P. Sandra, and G. Desmet, J. Chromatogr. A 1157, 131–141 (2007).

(11) A. Liekens, D. Cabooter, J. Denayer, and G. Desmet, J. Chromatogr. A 1217, 6754–6761 (2007).

(12) N. M. Devitt, R. E. Moran, J. M. Godinho, B. M. Wagner, and M. R. Schure, J. Chromatogr. A 1595, 117–126 (2019).

(13) C. Stassen, G. Desmet, K. Broeckhoven, L. Van Lokeren, and S. Eeltink, J. Chromatogr. A 1325, 115–120 (2014).

(14) F. Gritti and G. Guiochon, AIChE J. 56, 1495–1509 (2010).

(15) F. Gritti and G. Guiochon, AIChE J. 57, 333–345 (2011).

(16) N. Lambert and A. Felinger, J. Chromatogr. A 1565, 89–95 (2018).

(17) H. Song, Y. Vanderheyden, E. Adams, G. Desmet, and D. Cabooter, J. Chromatogr. A 1455, 102–112 (2016).

(18) J.H. Knox, J. Chromatogr. A 831, 3–15 (1999).

(19) J.H. Knox, J. Chromatogr. A 960, 7–18 (2002).

(20) A. Liekens, J. Denayer, and G. Desmet, J. Chromatogr. A 1218, 4406–4416 (2011).

(21) F. Gritti, A. Cavazzini, N. Marchetti, and G. Guiochon, J. Chromatogr. A 1157, 289–303 (2007).

(22) F. Gritti, I. Leonardis, J. Abia, and G. Guiochon, J. Chromatogr. A 1217, 3819–3843 (2010).

(23) F. Gritti and G. Guiochon, Chem. Eng. Sc. 66, 3773–3781 (2011).

(24) A. Andrés, K. Broeckhoven, and G. Desmet, Anal. Chim. Acta 894, 20–34 (2015).

(25) S. Deridder and G. Desmet, J. Chromatogr. A 1227, 194–202 (2012).

(26) D. Cabooter, J. Billen, H. Terryn, F. Lynen, P. Sandra, and G. Desmet, J. Chromatogr. A 1178, 108–117 (2008).

(27) H. Song, D. Sadriaj, G. Desmet and D. Cabooter, J. Chromatogr. A 1532, 124–135 (2018).

ABOUT THE AUTHORS

Kim Vanderlinden

Kim Vanderlinden is earning her PhD in Chemical Engineering at the Vrije Universiteit Brussel, Belgium.

Ken Broeckhoven

Ken Broeckhoven is an associate professor at the Department of Chemical Engineering at the Vrije Universiteit Brussel, Belgium. He has a Master's and a PhD in Chemical Engineering. His research focuses on the development of novel ultrahigh-pressure liquid chromatography instrumentation, fundamental aspects of supercritical fluid chromatography, investigation of the parameters affecting column performance, measurement and characterization of extra-column band broadening and the modeling of flow effects in chromatographic systems. He is the author of more than 60 peer-reviewed papers.

Gert Desmet

Gert Desmet heads the department of chemical engineering at the Vrije Universiteit Brussel, Belgium. His research mainly focuses on the miniaturization and automation of separation methods, as well as on the investigation and the modeling of flow effects in chromatographic systems. He is an Associate Editor for the journal "Analytical Chemistry" and holder of an ERC Advanced Grant.

ABOUT THE COLUMN EDITOR

David S. Bell

David S. Bell is a director of Research and Development at Restek. He also serves on the Editorial Advisory Board for LCGC and is the Editor for "Column Watch." Over the past 20 years, he has worked directly in the chromatography industry, focusing his efforts on the design, development, and application of chromatographic stationary phases to advance gas chromatography, liquid chromatography, and related hyphenated techniques. His main objectives have been to create and promote novel separation technologies and to conduct research on molecular interactions that contribute to retention and selectivity in an array of chromatographic processes. His research results have been presented in symposia worldwide, and have resulted in numerous peer-reviewed journal and trade magazine articles. Direct correspondence to: LCGCedit@ubm.com