Effects of Column Inner Diameter and Packed Bed Heterogeneities on Chromatographic Performance

Edward G. Franklin and Ty Kahler, Restek, Bellefonte, Pennsylvania, USA.

In recent years industry has been moving to columns with smaller and smaller inner diameters - moving from 4.6 mm and 3.0 mm i.d. columns to 2.1 mm, 1.0 mm, and even smaller. While small inner diameter columns have some clear advantages, they also bring challenges. Reduction of extracolumn volumes must be given greater consideration by both customers and manufacturers. This article focuses on the sources of band broadening within high performance liquid chromatography (HPLC) columns with an emphasis on eddy dispersion. The physical mechanisms of dispersion are discussed and a review of the current literature as it pertains to small inner diameter columns is presented.

The observed efficiency of an isocratic chromatographic separation is a function of the column itself (that is, its intrinsic efficiency) and the band broadening contributions of the instrument (for example, the injection process, the connecting tubing, the detector volume, and so forth) (1). The volume variances of these elements are additive, as shown in equation 1:

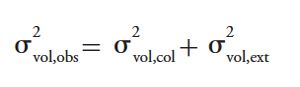
[1]

where σ2vol,obs is the volume variance of the observed peak, and σ2vol,col and σ2vol,ext are the variance contributions of the column and the extracolumn or instrument volumes, respectively.

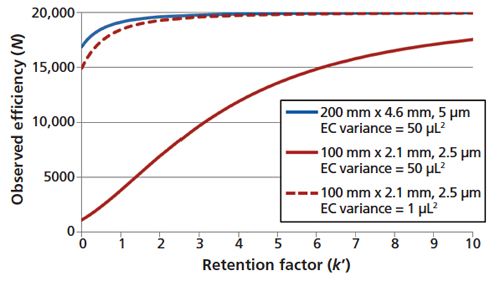
Before the introduction of ultrahigh‑pressure liquid chromatography (UHPLC) and columns packed with sub-2-μm particles, extracolumn volume contributions to the measured widths of chromatographic peaks were rarely seen as problematic (2). For example, a 200 mm × 4.6 mm column packed with 5-μm fully porous particles might have an intrinsic efficiency of 20,000 plates under typical isocratic elution conditions. The peak volume of a lightly retained analyte (k′ = 1) is approximately 132 μL. Using an instrument with even a relatively large variance contribution (50 μL2) results in an observed efficiency loss of less than 5%. A 100 mm × 2.1 mm column packed with 2.5-μm fully porous particles would exhibit a similar intrinsic efficiency (N = 20,000), but the peak volume would be much smaller (<14 μL), and the variance contribution of the instrument would be detrimental; approximately 3800 plates would be observed. Fortunately, a generation of UHPLC instruments has been designed that addresses extracolumn volumes and limits system volume variances to 1–10 μL2 (3). In a best case scenario, the previously described 100 mm × 2.1 mm column would exhibit approximately 92% of its intrinsic efficiency for a lightly retained analyte (k′ = 1) and 98% for an analyte with a retention factor of 3. Figure 1 shows the extracolumn volume effects on the observed efficiencies of columns capable of generating 20,000 theoretical plates.

Figure 1: Observed column efficiency (N) as a function of retention factor (k’) for instruments with volume variance contributions of 1 and 50 μL2.

Instrument design continues to be pushed by advancements in column technologies. Ever smaller particles are consistently attractive because plate height (H) scales with particle diameter (dp). However, the use of higher operating pressures, which are needed to realize those theoretical efficiency gains, results in frictional heating. Frictional heating can give rise to radial temperature gradients within the column that lead to decreased efficiency (4). Reducing the inner diameter of the column improves heat dissipation and also allows for the use of lower flow rates. This reduction of column diameter and flow rate is attractive from an economic perspective (that is, in the reduction of mobile phase solvent costs) as well as in clinical, forensic, environmental, and pharmaceutical settings where samples may be especially precious or limited. Reduced flow rates are also advantageous when attempting to improve the limits of detection (LOD) of methods that couple high performance liquid chromatography (HPLC) to electrospray ionization mass spectrometry (ESI-MS) (5).

Chromatographic Band Broadening: The Column

Given what has been said about observable column efficiencies as a function of instrument dispersion, it can be seen how highly efficient, narrow‑bore columns packed with small particles challenge the present state of instrument design. But now we turn our attention to the band broadening contributions of the column itself. The van Deemter equation is a classical expression of band broadening that groups the various contributions according to their dependencies on mobile-phase linear velocity, uavg. It is commonly expressed in reduced terms so that meaningful comparisons between columns can be made, regardless of particle size:

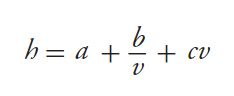
[2]

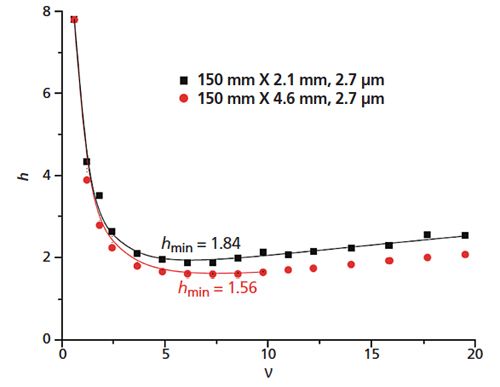
where h is the reduced plate height (H/dp), v is the reduced velocity (uavg dp/Dm), and Dm is the bulk molecular diffusion coefficient. The a , b, and c terms are coefficients that describe the band broadening contributions from eddy dispersion, longitudinal diffusion, and resistance to mass transfer, respectively. By a first approximation, we might expect to achieve the same intrinsic efficiency and reduced plate height (for example, h = 1.8) with a 50 mm × 1.0 mm column packed with 1.25 μm particles (H = 2.25 μm; N = 22,000) as we would with a 200 mm × 4.6 mm column packed with 5-μm particles (H = 9 μm; N = 22,000) in 1/16 the time and with a fraction of the flow rate. However, this would be true only if the structures of the packed beds were conserved independently of particle size and column diameter such that each of the terms in equation 2 remained constant (6). A comparison of experimental h–v data collected using columns packed with several types of core–shell particles reveals that these values are often affected by column inner diameter (7–10). Without exception, the minimum intrinsic plate heights obtained when using 4.6-mm i.d. columns are smaller than those obtained when using 2.1-mm i.d. columns packed with identical particles, as exemplified by the data presented in Figure 2. Likewise, the slopes of the h–v curves in the mass transfer (c term) dominated regions are slightly larger for the 2.1-mm i.d. columns than for the 4.6-mm i.d. columns. The mechanisms of mass transfer alone cannot explain these observations (6). Let us therefore turn our attention to a more rigorous model of band broadening capable of providing additional insight.

Figure 2: h–v curves of columns packed with 2.7-μm core–shell particles. The 4.6-mm i.d. column has a smaller minimum intrinsic plate height than the 2.1-mm i.d. column. (Adapted with permission from reference 10.)

The Giddings model describes plate height as a function of average mobile‑phase linear velocity, and it couples transverse (that is, radial) diffusion of the analytes and fluctuations in linear velocity, which occur in different regions of the packed bed (11):

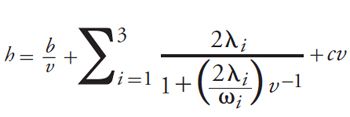
[3]

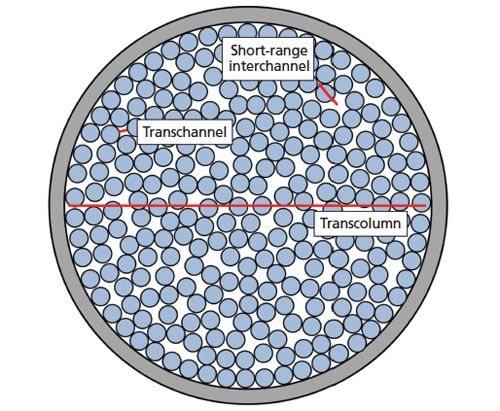
The first and last terms describe longitudinal diffusion and mass transfer, respectively. The remaining terms characterize eddy dispersion on time scales consistent with three categorical distances within the column, as shown in Figure 3. Unlike the van Deemter equation, eddy dispersion is presented here as velocity-dependent. λi and ωi represent structural parameters of the packed bed characteristic of each eddy dispersion term. As such, this coupling model provides physical correlatives to the parameters involved in describing eddy dispersion. The transchannel contribution (i = 1) accounts for mobile phase velocity differences within the small, interstitial channels between neighbouring particles. Although the channels in packed beds have very complicated geometries, a parabolic flow profile with the highest velocities occurring at the channel centres and the lowest velocities occurring at the particle interfaces can be envisioned. Short-range interchannel contributions (i = 2) describe the effects of velocity differences between channels separated by only a few particle diameters. These differences can arise because of small regions within the packed bed where particles group and pack more tightly than other particles in the immediate surrounding region. Finally, the transcolumn contribution (i = 3) characterizes the analyte band broadening that results from velocity differences existing between different regions of the column, such as between the centre of the column and the column walls. These velocity differences are the result of radial heterogeneities in the packed bed, which are largely attributable to the effects of the column walls (12).

Figure 3: Cross-section of a particle-packed column showing the three categorical distances over which mobile phase velocity fluctuations occur. Each distance is related to an eddy dispersion term in the Giddings model to describe plate height: transchannel (i = 1), short-range interchannel (i = 2), and transcolumn (i = 3).

The Column: Eddy Dispersion

The extent of analyte band broadening attributable to each of the eddy dispersion terms mentioned above (transchannel, short-range interchannel, and transcolumn) are characterized by their respective quantities λi and ωi. Looking at equation 3, we can identify reduced velocities at which the plate height contributions of each term reach half their limiting values, after which the individual heddy,i–v curves notably flatten. These are known as the transition velocities, v1/2 (12):

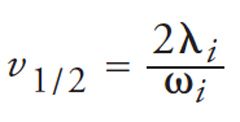
[4]

Before the transition velocity, the magnitude of each term increases with linear velocity such that the contribution appears indistinguishable from the mass transfer term (cv). The transchannel and transcolumn contributions are characterized by very high transition velocities. Only the values of λ2 and ω2 are such that the coupling characteristic of the short-range interchannel term remains relevant to the practical range of HPLC operation (1 ≤ v ≤ 20). This is why the van Deemter equation remains an accurate quantitative expression of plate height data even as it assigns incorrect relative plate height contributions to each of the a, b, and c terms. The physical and morphological characteristics of the packed bed that contribute to eddy dispersion are manifested in the c term (12,13).

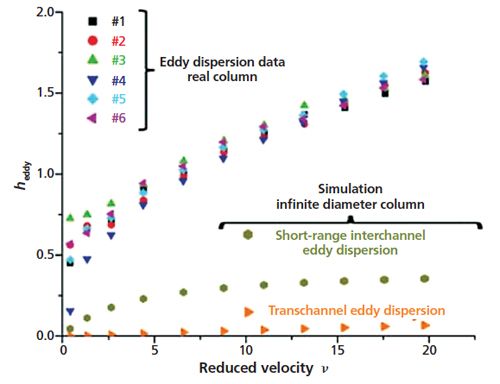
Simulations of band broadening in computer-generated sphere packings allow for comparison of the relative contributions of transchannel, short-range interchannel, and transcolumn eddy dispersion to total eddy dispersion (12). By simulating an “infinite bed”, total eddy dispersion is reduced to the sum of the transchannel and short-range interchannel terms. An experiment comparing real eddy dispersion data collected using 100 mm × 4.6 mm columns packed with 2.7-μm core–shell particles to simulated data generated from a corresponding “infinite bed” reveals the relative importance of the transcolumn contribution (13). As shown in Figure 4, the total eddy dispersion observed with the real columns is nearly three times higher than that of the simulated infinite bed, with the difference being mainly attributable to transcolumn dispersion. It is clear, then, that the efficiency of HPLC columns is largely affected by the confinement of the pack bed (that is, wall effects), which results in radial heterogeneity and a significantly large transcolumn dispersion term.

Figure 4: Real eddy dispersion data collected using 100 mm × 4.6 mm columns packed with 2.7-μm core–shell particles compared to dispersion data calculated for a simulated “infinite bed.” The differences between the total eddy dispersion of the real data and the sums of the short-range interchannel and transchannel eddy dispersion contributions from the simulated infinite bed data are largely attributable to transcolumn dispersion, induced by wall effects. (Adapted with permission from reference 13.)

The Column: Wall Effects and Radial Heterogeneity

There are two types of wall effects that result in the radial heterogeneity of packed beds (14). The first is a geometrical effect occurring at the column wall. Particles abut against the wall but cannot penetrate it. This leads to a highly ordered monolayer of particles along the wall. Subsequent layers are increasingly disordered to a distance of approximately 5 dp from the column wall, after which a random packing arrangement is achieved (12). These differences in packing arrangements and densities along the column radius mean that there are different interstitial porosities at different points in the column. This leads to higher-than-average mobile-phase velocities at the wall regions compared to the centre of the column. A chromatographic band broadens as analyte molecules sample these different velocity flow paths, and the extent of this broadening is characterized in the transcolumn eddy dispersion term. The second wall effect is manifested as a region of higher-than-average packing density occurring between 5 and 50 dp from the column wall. The extent of this effect is dependent upon the conditions used to pack the column and is due to the combination of radial and axial stresses present during bed consolidation (14,15).

Column Inner Diameter: 4.6 mm and 2.1 mm

Keeping in mind the contributions to total eddy dispersion, let us return our attention to the relative performances of 4.6-mm i.d. and 2.1‑mm i.d. columns packed with identical particles. As mentioned, 4.6‑mm i.d. columns consistently exhibit lower intrinsic minimum plate heights and better performance at high mobile‑phase linear velocities than their 2.1-mm i.d. counterparts (10). Gritti and Guiochon (13) attribute this to the respective heterogeneities of the packed beds (that is, differences between the respective transcolumn eddy dispersion terms). In other words, the empirically determined values λ3 and ω3 increase with decreasing column radius.

So far we have been concerned with the radial heterogeneity of packed beds and its effect on plate height. At this point, we should also mention a specific matter with regard to these column dimensions. When sample is introduced onto a column, it occupies a relatively narrow region at the centre of the packed bed, characterized by the average flow velocity. As the sample migrates axially down the column, it also disperses radially outward towards the column walls (16). For columns with inner diameters ranging from 2.1 to 4.6 mm and lengths between 5 and 25 cm, lightly retained analytes are often eluted from the column in less time than it takes for analyte molecules to reach the column walls where mobile-phase flow velocity can be much different than it is at the centre of the column. In such cases, there is an increasing total eddy dispersion contribution to plate height with decreasing column diameter. In addition to wall effects, border effects at the outlet of the column become increasingly important with decreasing inner diameter. With narrower inner diameter columns, analytes have a greater chance to reach the radial edge of the outlet frit where stagnant regions of mobile‑phase eluent may be found. These outer flow streams must then distort and converge upon the narrow outlet aperture to exit the column, thus leading to increased band broadening and peak tailing (13). Axial and transverse dispersion in relatively short, wide columns is illustrated in Figure 5(a).

Figure 5: Representations of axial and transverse dispersion of an analyte zone within a column. (a) In short and wide columns, lightly retained analytes are often eluted from the column in less time than it takes for analyte molecules to reach the column walls. As the column inner diameter decreases, wall and border effects become increasingly important. (b) In capillary columns, the retention times of analytes are much longer than the time it takes for analyte molecules to sample the column diameter many times. Radial heterogeneities induced by wall effects are a dominating factor in column efficiency (13).

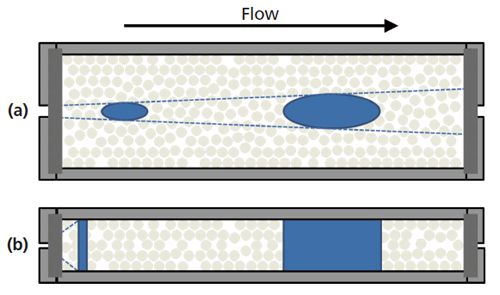
Parallel segmented flow chromatography (PSFC) has been suggested as a means to improve the performance of columns that are relatively wide and short (17–19). With this technology, a column is equipped with an outlet fitting that features a number of peripheral exit ports and a single central exit port. Mobile phase and analytes exiting through the central port are sent on for detection, while flow streams from along the walls of the column are collected and discarded through the peripheral ports. Improvements are realized if, for instance, the average mobile phase velocity at the centre of the column is higher than that at the wall regions (see the second wall effect described above). In such a case, elimination of the peripheral flow streams can increase the observed efficiencies and eliminate tailing. PSFC has been shown to dramatically improve the performance of 30 mm × 4.6 mm columns by reducing the combined influence of wall and border effects. In longer, narrower columns, analyte molecules have enough time before elution to reach the column wall and to statistically sample all of the available flow path velocities. There are no improvements to observed efficiencies when using PSFC in such cases.

Column Inner Diameter: Capillary Columns

As the diameter of the column narrows into the capillary range, the elution times of analytes becomes much longer relative to the times needed for analyte molecules to radially disperse to the column walls (13). The analyte molecules are thus able to sample the different velocity flow paths (induced by heterogeneities in the packed beds) many times. In such cases, wall effects are a dominating factor in column efficiency. This situation is illustrated in Figure 5(b). Bruns and colleagues (20) describe an experiment wherein 20 cm capillaries with inner diameters of 10, 20, 30, 50, and 75 μm were packed with 1.7-μm fully porous particles, and reduced plate heights as a function of linear velocity were observed. Representative segments of each packed bed were reconstructed through confocal scanning laser microscopy (CLSM), and radial interparticle porosity profiles were determined to quantify the extent of transcolumn heterogeneity. Capillary columns with larger inner diameters (that is, 30, 50, and 75 μm) were found to exhibit larger porosity fluctuations at the column walls before converging to the mean porosity values characterized by the random packing arrangements at the column centres. Analyte molecules experience more mobile-phase flow velocity differences across the diameter of the column, thus leading to high transcolumn dispersion and poorer chromatographic performance, as shown in Figure 6. Capillary columns with smaller inner diameters (that is, 10, 20, and 30 μm) were found to exhibit smaller radial porosity fluctuations, and thus performed better chromatographically. This latter result is partially explained through envisioning the lower aspect ratios of the column to particle diameters as substantially eliminating the bulk regions of the packings. In other words, packed‑bed morphologies in very narrow capillaries are dictated almost entirely by wall effects and are therefore
much more radially homogeneous (21,22).

Figure 6: h–v curves of different inner diameter capillary columns packed with 1.7‑μm fully porous particles. Confocal scanning laser microscopy (CLSM) data reveals the extent of radial heterogeneity for the poorer performing columns in Group 1 to be greater than the heterogeneities of columns in Group 2. (Adapted with permission from reference 23.)

At this point, it may be clear that reducing the radial heterogeneity of packed beds to reduce transcolumn dispersion is of special importance. Reising and colleagues (6) describe an experiment seeking to correlate column packing procedures, chromatographic performance, and the physical morphologies of packed beds as determined by CLSM. Nine 34 cm × 75 μm columns packed with the same batch of 1.3-μm fully porous particles using slurry concentrations ranging from 5 to 50 mg/mL were prepared. Examination of the physical morphologies revealed two competing effects of using increasingly concentrated particle slurries. Firstly, higher slurry concentrations effectively reduced the heterogeneities induced by the wall regions (6,23). Secondly, however, higher slurry concentrations resulted in a greater number of void regions within the packed bed. The effects of such voids can be manifold. Additional transchannel and short‑range interparticle contributions to eddy dispersion are likely, and additional transcolumn contributions are also possible if the voids are preferentially located in the wall regions. Chromatographic testing showed the column prepared with the 20 mg/mL slurry to perform best while those prepared with lower and higher slurry concentrations exhibited higher minimum plate heights and larger slopes in the h–v curves. There exists, then, an optimum intermediate slurry concentration where all other column packing conditions are more or less constant. This work suggests the possibility of improved chromatographic packings through the use of packing conditions that minimize wall effects and reduce radial heterogeneity while also avoiding the formation of larger
voids (24).

Summary

Intrinsic column performance as a function of column inner diameter has been observed and investigated by a number of practitioners and researchers, and the morphological structures of packed beds have received particular attention. The radial heterogeneity of a packed bed, specifically the differences between the packing structures at the column walls and the centre of the column, is a key contributor to chromatographic band broadening. The arrangement of particles at the column walls are often such that the interstitial porosities in those regions are higher than the porosities of the packing arrangements at the centre of the column. This leads to mobile‑phase flow velocity differences across the column diameter, which result in transcolumn eddy dispersion. It has been estimated that up to 70% of the total dispersion of a chromatographic peak is attributable to transcolumn eddy dispersion (13).

The effects of column radial heterogeneity (and border effects) become increasingly pronounced as column inner diameter decreases from 4.6 mm to 2.1 mm, as reflected in the diminished chromatographic performance of 2.1-mm i.d. columns (10,13). As column diameter narrows further into the capillary regime, wall‑induced radial heterogeneities become more and more important because analyte molecules have sufficient opportunity to sample the column diameter (and the different velocity flow paths) many times before elution. Very narrow microcapillary columns have displayed excellent intrinsic efficiencies because, as the aspect ratio of the column to particle diameter decreases, packed-bed morphologies are almost entirely dictated by the column walls and are therefore more radially homogeneous (20–22).

Given the attractiveness of reduced solvent consumption and increased sensitivity that is associated with the use of smaller inner diameter columns, instrument manufacturers, column manufacturers, and users continue striving to improve the observed performances of such columns. The reduction of extracolumn volumes and the design of less dispersive sample injection and detection schemes remain relevant along with methodological approaches involving sample trapping and gradient elution. Parallel segmented flow has been used to operate 4.6-mm i.d. columns as virtual 2.1-mm i.d. columns by tuning the ratio of peripheral flow stream mobile phase that is discarded before detection. This approach has been shown to significantly improve observed efficiencies (25). In capillaries, the correlation of column packing parameters and chromatographic performance to the morphological structures of packed beds suggests possible means to improve the intrinsic efficiencies of columns by improving radial homogeneity and reducing transcolumn eddy dispersion (6). Such approaches may be applicable across a range of column inner diameters.

References

1. A. Prüß, C. Kempter, J. Gysler, and T. Jira, J. Chromatogr. A1016, 129–141 (2003).
2. F. Gritti and G. Guiochon, J. Chromatogr. A1327, 49–56 (2014).
3. J. De Vos, K. Broeckhoven, and S. Eeltink, Anal. Chem.88, 262–278 (2016).
4. A. de Villiers, H. Lauer, R. Szucs, S. Goodall, and P. Sandra, J. Chromatogr. A1113, 84–91 (2006).
5. J.P. Chervet and M. Ursem, Anal. Chem. 68, 1507–1512 (1996).
6. A.E. Reising, J.M. Godinho, K. Hormann, J.W. Jorgenson, and U. Tallarek, J. Chromatogr. A1436, 118–132 (2016).
7. F. Gritti and G. Guiochon, J. Chromatogr. A 1252, 31–44 (2012).
8. F. Gritti and G. Guiochon, J. Chromatogr. A1252, 45–55 (2012).
9. F. Gritti and G. Guiochon, J. Chromatogr. A1252, 56–66 (2012).
10. F. Gritti and G. Guiochon, J. Chromatogr. A1218, 1592–1602 (2011).
11. J.C. Giddings, Dynamics of Chromatography, Part 1: Principles and Theory (Marcel Dekkar, New York, New York, USA, 1965).
12. S. Khirevich, A. Höltzel, A. Seidel‑Morgenstern, and U. Tallarek, Anal. Chem. 81, 7057–7066 (2009).
13. F. Gritti and G. Guiochon, Anal. Chem.85, 3017–3035 (2013).
14. R.A. Shalliker, B.S. Broyles, and G. Guiochon, J. Chromatogr. A888, 1–12 (2000).
15. B.G. Yew, J. Ureta, R.A. Shalliker, E.C. Drumm, and G. Guiochon, AlChE J. 49, 642–664 (2003).
16. R.A. Shalliker, B.S. Broyles, and G. Guiochon, J. Chromatogr. A994, 1–12 (2003).
17. F. Gritti and G. Guiochon, J. Chromatogr. A1297, 64–76 (2013).
18. F. Gritti and G. Guiochon, J. Chromatogr. A1314, 44–53 (2013).
19. F. Gritti, J. Pynt, A. Soliven, G.R. Dennis, R.A. Shalliker, and G. Guiochon, J. Chromatogr. A1333, 32–44 (2014).
20. S. Bruns, J.P. Grinias, L.E. Blue, J.W. Jorgenson, and U. Tallarek, Anal. Chem.84, 4496–4503 (2012).
21. R.T. Kennedy and J.W. Jorgenson, Anal. Chem.61, 1128–1135 (1989).
22. S. Hsieh and J.W. Jorgenson, Anal. Chem.68, 1212–1217 (1996).
23. S. Bruns, E.G. Franklin, J.P. Grinias, J.M. Godinho, J.W. Jorgenson, and U. Tallarek, J. Chromatogr. A1318, 189–197 (2013).
24. M.R. Shure and R.S. Maier, J. Chromatogr. A1126, 58–69 (2006).
25. R. Shalliker, M. Camenzulli, L. Pereira, and H.J. Richie, J. Chromatogr. A1262, 64–69 (2012).

Edward G. Franklin is a product development chemist at Restek. He earned a Bachelor’s degree from Duquesne University in Pittsburgh and a PhD in analytical chemistry from The University of North Carolina at Chapel Hill. Ed joined Restek in 2012 and his main focus is the development of new chromatographic technologies.

Ty Kahler joined Restek as a Senior Innovations Chemist in 2008 and has since become the Manager of the HPLC R&D and Applications group. In addition to overseeing the HPLC lab, his responsibilities include LC methods development, quality investigation and implementation, and phase design and research. Before joining Restek, Ty worked as a manager, study director, and principal investigator for a contract research laboratory conducting methods development, validation, and routine analysis of pharmaceuticals and agrochemicals. He has been in the field of liquid chromatography for more than 16 years, including time as a quality chemist and instrumentation metrologist.

David S. Bell is a manager in pharmaceutical and bioanalytical research at MilliporeSigma (formerly Sigma-Aldrich/Supelco). With a B.S. degree from SUNY Plattsburgh and a PhD in analytical chemistry from The Pennsylvania State University, Dave spent the first decade of his career within the pharmaceutical industry performing analytical method development using various forms of chromatography and electrophoresis. During the past 15 years, working directly in the chromatography industry, Dave has focused his efforts on the design, development, and application of stationary phases for use in HPLC and hyphenated techniques. In his current role at MilliporeSigma,
Dr. Bell’s main focus has been to research, publish, and present on the topic of molecular interactions that contribute to retention and selectivity in an array of chromatographic processes. Direct correspondence to: LCGCedit@ubm.com