The LCGC Blog: So Just How Well Set-Up is Your UV Detector?

Most of us who have used UV detection for HPLC analyses consider them very straightforward and find they produce fit for purpose data whenever we need them to.

However, there are instances when we need our detectors to perform "above the norm," or we may have simply learned to live with the level of performance we get from these instruments. Could we be doing better? Could we be getting better baselines, better sensitivity, or better reproducibility from our UV detectors without too much effort? The answer is almost invariably yes – and here's how.

Principles

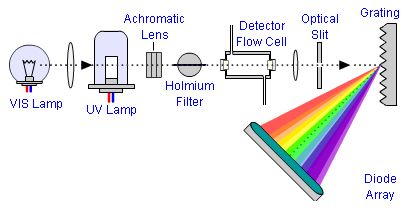
Figure 1: Schematic of the Reversed Optics of a typical Diode Array UV detector used in HPLC systems.

In this typical Diode Array detector (so called "Reversed Optics"), light from a Tungsten and or Deuterium lamp is focussed onto the detector flow cell, where light of a certain wavelength will be absorbed according to the electronic configuration of the analytes flowing through the cell. The slit is used to control how the light falls onto the grating, and ultimately controls the balance between spectral resolution and sensitivity (signal to noise). The grating is used to split the emergent light into its constituent wavelengths which then fall onto a diode which measures the light intensity across the selected wavelength range.

Choosing your flow cell

The choice of flow cell volume and type will directly affect the sensitivity of your method and the efficiency of the peaks within your chromatogram.

Larger volume flow cells have a larger path length and therefore, in keeping with the Beer-Lambert Law (Eqn. 1), will give rise to more intense sample signals, improved signal to noise ratio and better analytical sensitivity.

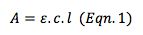
A = dimensionless quantity measured in absorbance units
ε = molar extinction co-efficient (mol^-1dm³cm^-1)
l = path length of flow cell (cm)
c = concentration (mol dm^-3)

However, as HPLC column dimensions reduce, as they are tending to do these days, the volume of eluent containing the total analyte band becomes smaller (i.e. the peak volume is small). If this small volume peak elutes into a large volume flow cell, a great deal of dispersion will occur and the analyte band will become broadened and the signal to noise ratio significantly reduced.

The ideal solution is to have a high path length flow cell with a low volume – which is why many manufacturers now offer the option of a "light-pipe" design, which satisfies both these conditions, while having the added benefit of increased absorbance through total internal reflection of the light beam which results in higher response and improved signal to noise ratios.

One good rule of thumb is to restrict the internal volume of the flow cell to no more than 10% of the peak volume and typically one would choose an early eluting, narrow peak, to make the assessment.

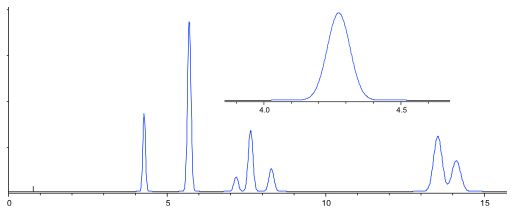
At 2.0 mL/min. eluent flow, this peak represents an elution volume of 0.35 min x 2.0 = 700 µL. Therefore, maximum flow cell volume would be 70 µl – easily achievable.

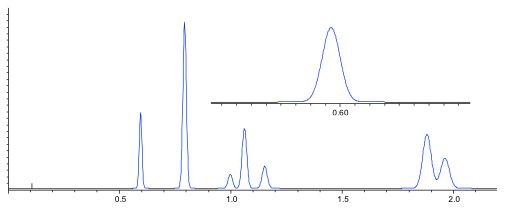
At 1.0 mL/min. eluent flow, this peak represents an elution volume of 0.04 min x 1.0 = 40 µL. Therefore maximum flow cell volume would be 4 µl – more challenging.

Figure 2: Assessing flow cell volume requirements to avoid excessive extra column band broadening from the chromatographic peak width.

* - ultimate efficiency depends upon the total system extra column volume and column dimensions

Table 1: Effect of flow cell volume on chromatographic performance.

Setting the Slit Width

Some instruments include an electro-mechanical slit which is programmable. A narrow slit width provides improved spectral resolution for analytes which give UV spectra with enough fine detail to be useful for qualitative analysis. Improved spectral resolution will increase the confidence of library matching search results when attempting to identify unknown peaks within a chromatogram. A wide slit width allows more of the light passing through the flow cell to reach the photodiode array; hence, the signal intensity and detector sensitivity will increase. Baseline noise will also be reduced leading to an increase in signal to noise ratio. However, with a wider slit width the optical resolution of the spectrophotometer (its ability to distinguish between different wavelengths) diminishes. The wavelength of light falling on each diode becomes less specific as the light becomes more diffuse. Any photodiode receives light within a range of wavelengths determined by the slit width, and so spectral resolution decreases.

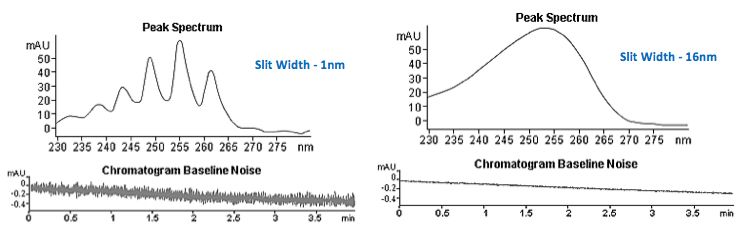
Figure 3: Effects of slit width on sensitivity and resolution of the UV response.

We probably all know how important it is to select the correct sampling rate in order to achieve the optimum resolution and peak modelling – especially as this parameter has become more important with the advent of UHPLC and the very narrow peaks generated, which drives the faster sampling rates of modern UV detectors.

Although debate rages, I've always found that 20-25 samples (measurements) across the peak has given rise to good quantitative reproducibility and peak shape modelling (sensitivity). However, we can also be OTT with respect to data sampling and high sampling rates and fast response times (Time Constants) can give rise to some very noisy baselines – which can become problematic when measuring smaller peaks in the presence of larger ones (trace analysis). While high data sampling rates are to be encouraged, we should be mindful that we may need to alter the time constant in order to electrically filter out some of the associated noise. These principles are outline in Figure 4.

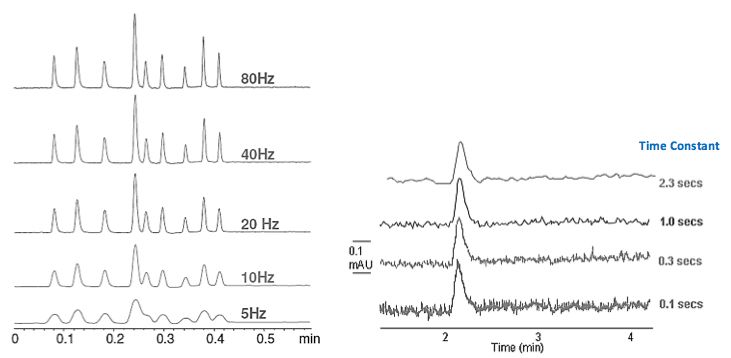
Figure 4: Left – effect of data sampling rate on peak shape, resolution and sensitivity. Right – use of time constant to adjust noise, which is especially important in high frequency measurements.

Statistically the noise of a system is defined by 1/√n, where n is the number of data points – therefore, doubling the data sampling rate will result in an increase of around 5% in peak height but also an increase of baseline noise by a factor of 1.4 – which is a balance that needs to be carefully considered!

Bandwidths and References

There are almost as many opinions on the setting of acquisition and reference wavelengths and bandwidths as there are authors on this subject – and here is another! I like to use real life examples wherever possible – so here is one we made earlier.

I always chose a "maxima" within the analyte UV spectrum, to give a good compromise between sensitivity, whilst keeping the acquisition wavelength well above 200 nm, to avoid absorbance effects from the organic solvents and additives we use in UV detection (yes – it's not just the organic solvent which absorbs!!). In the example shown below using the maleic anhydride veratrylamine derivative (MAVA), I chose 228 nm. There are arguments about choosing the peak maximum and robustness – however I figure if you have a reasonable bandwidth setting and your instrument is half decent, there shouldn't be any issues with wavelength "drift" causing irreproducible absorbance between analyses or even over a long campaign of samples.

The bandwidth parameter in diode array detection is related to the number of diode responses which are averaged in order to obtain a signal at a particular wavelength. A wide bandwidth has the advantage of reducing noise by averaging over a greater diode range. Noise is random; therefore, averaging the response over a large range of diodes will reduce noise. As the bandwidth is increased, the signal intensity (detector sensitivity) increases as some diodes will result in a lower absorbance compared to a reading using only the single most intense wavelength (λmax). A wide bandwidth results in a larger range of wavelengths being averaged when producing a spectral data point, which results in a loss of spectral resolution.

To choose a reasonable bandwidth setting, I imagine the spectral feature I'm working with as a discrete peak (red lines in Figure 5) and set the bandwidth to the peak width at half height for this imaginary feature within the spectrum - a value of 40 nm was chosen in the example (208 to 248 nm).

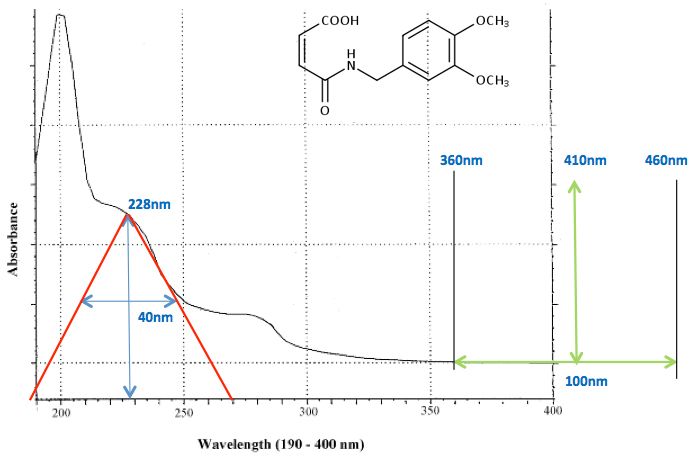
Figure 5: Using the analyte UV spectrum to determine the correct sample and reference wavelength and bandwidth settings.

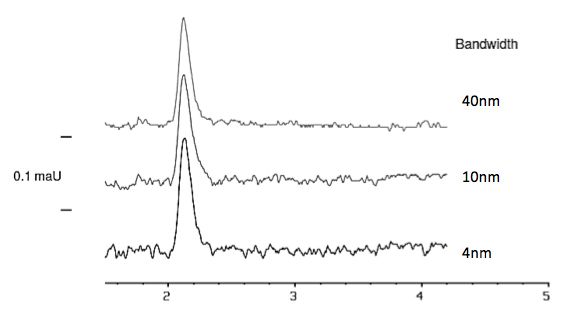
Figure 6: Effect of altering the bandwidth on signal to noise ratio in UV determinations.

Some manufacturers offer the opportunity to set a reference wavelength. The reference wavelength compensates for fluctuations in lamp intensity as well as changes in the absorbance/refractive index of the background (i.e. mobile phase) during gradient elution. During gradient elution the composition of the eluent will change, as will its refractive index, which will affect absorbance as the Beer Lambert Law in only linear when constant refractive index is assumed. To compensate for the change in refractive index properties a reference wavelength can be set to avoid baseline drift (Figure 7). Noise will also be reduced as the reference wavelength is moved closer to the sample signal. Without any reference measurement all noise and variability in lamp intensity is recorded within the signal. When using a reference signal all lamp intensity and background (mobile phase) variability is subtracted out of the signal being measured. The closer the reference wavelength is to the sample wavelength the more effectively these background deviations are catered for and the better the detector sensitivity. However, the reference wavelength should not be selected too close to the analyte wavelength or the signal intensity may be seriously reduced.

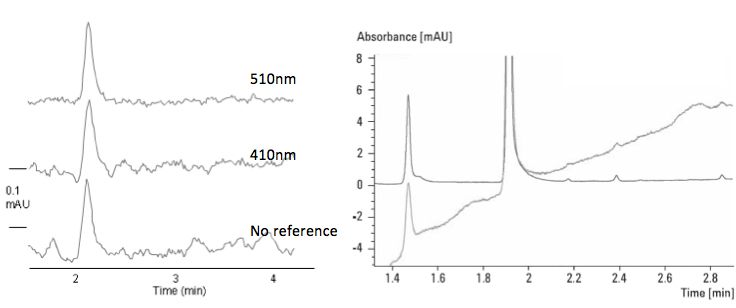
Figure 7: Effects of choosing an appropriate reference wavelength on (Left) – baseline noise and (Right) baseline drift during gradient elution.

My usual approach to setting reference wavelength (and reference bandwidth if it's available), is also shown in Figure 5. The reference is chosen at the point at which the analyte spectrum falls below 0.1 mAu (i.e. negligible absorbance form the analyte) PLUS 50 nm – around 400 nm in the example shown. If a reference 'window' can be chosen, the window begins at 10 nm above the point at which the analyte stops absorbing (360 nm in the example) and the window itself is 100 nm wide, giving a reference wavelength of the window start wavelength PLUS 50 nm (410 nm in the example).

For more information – contact either
Bev ([email protected]) or Colin ([email protected]).

For more tutorials on LC, GC, or MS, or to try a free LC or GC troubleshooting tool, please visit www.chromacademy.com