Making the Most of a Gradient Scouting Run

The use of a gradient scouting run can be a powerful tool to jump-start method development. But what conditions should be chosen, and what do we do with the results?

Those of us involved in the development of liquid chromatography (LC) methods are under constant pressure from our clients to spend less time in the development process — they'd like the method yesterday! One of the challenges when we face such requirements is how to make the most of each LC run. The use of a gradient scouting run can be a very efficient way to get to the fine-tuning stage of development faster. We'll see that even if our goal is an isocratic run, starting with a gradient can be a faster way to that goal than starting with an isocratic separation. Another factor that often confuses the process today is the large choice of column and particle sizes available. Are we using a conventional 150 or 250 mm × 4.6 mm, 5-µm particle column, or an ultrahigh-pressure LC (UHPLC) system with a 50 mm × 2.1 mm, ≤2-µm column? How do we start with any of these and still get acceptable results? For the present discussion, we'll assume that a reversed-phase column will be used This is a good assumption, because somewhere around 70–80% of all methods are developed on a C8 or C18 reversed-phase column. These, and some related topics, will be covered in this month's "LC Troubleshooting."

The Isocratic Way

Isocratic methods, where the mobile-phase concentration is constant throughout the run, are the technique of choice for most chromatographers. This is because such methods tend to be more intuitive to develop and adjust, they don't require waiting for the column to equilibrate between runs, and artifact peaks from the mobile phase are less of a problem than in gradient elution. The age-old technique to develop isocratic methods is quite simple. Just start at a high percentage of the B, or organic, solvent (usually acetonitrile or methanol), step back in 10% increments until you obtain a promising chromatogram, and then fine-tune it. So, 90% B, 80%, 70%, and so forth. This tried-and-true technique has been in use as long as modern LC has, but even under the best circumstances, it may take half a day or more before you have a glimpse of potential isocratic conditions, and you may be thoroughly disappointed when the polarity range of your samples is so large that a single isocratic run may not be possible.

The Gradient Scouting Run

With isocratic runs, it is desirable to have the peaks contained in a retention factor, k, window of 1 = k = 20, or better yet, 2 = k = 10. When peaks are eluted within this range, the retention time of the first peak isn't so short that it runs into the garbage at the solvent front, and the last peak is not retained so strongly that it seems to take forever to come off the column, and is broad and hard to distinguish from the baseline.

When gradient elution is used, isocratic k values cannot be used. Instead a gradient retention factor, k*, describes retention, and often is described as an average retention factor. The following relationship can be used to approximate k*:

k* ≈ (t_G × F)/(Δ%B × V_m × 5) [1]

where t_G is the gradient time in minutes, F is the flow rate in milliliters per minute, Δ%B is the gradient range as a decimal (for example, 5–95% B gives Δ%B = 0.90), and V_m is the column volume in milliliters. The factor 5 is used for small-molecule samples (for example, molecular weights <1000 Da). Notice that a single value of k* is assigned for the gradient, rather than a range of k values, as in isocratic. Again, this is an approximation — although k* values differ somewhat for different analytes, a single value of k* is sufficiently accurate for the present purposes.

We can rearrange equation 1 to make it more useful for our current purpose of designing scouting gradients:

t_G ≈ (5 × k* × V_m × Δ%B)/F [2]

Equation 2 allows us to find scouting gradient conditions for an unknown sample and a specific experimental setup. All we need to do is fill in the unknown quantities on the right side of equation 2 and we will know the gradient time to use. As mentioned above, the gradient k* value will be approximately the same for all peaks in a separation. The same general rules apply for k* as for k — that is, we'd like k* in the 2 ≤ k* ≤ 10 range, just like isocratic k. A value in the middle of the range is a good place to start, so we'll use k* ≤ 5.

Next, we need an estimate of the column volume. This is the volume of mobile phase within the column and usually is 60–70% of the volume of the empty column. A good estimate (±10%) can be made as follows:

V_m ≤ (0.5 × L × d_c² )/1000 [3]

where L is the column length and d_c is the column internal diameter, both in millimeters. If we use the most popular column size, 150 mm × 4.6 mm, we get a value of V_m = 1.6 from equation 3. In Table I, I've shown the volumes of some other popular column sizes.

Because we generally don't know the polarity range of an unknown sample, it is a good idea to use a wide gradient range. Also, to avoid column dewetting, it is best to start the gradient at ≥5% B. We can end the gradient at 90–100% B. For the present example, we'll run 5–95% B, so Δ%B = 0.9. With a 150 mm × 4.6 mm column packed with 5-µm diameter particles, we can use flow rates of 1–2 mL/min without excessive pressure. I'm impatient, so let's use 2 mL/min.

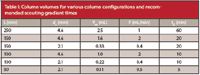
Table I: Column volumes for various column configurations and recommended scouting gradient times

The conditions chosen above give t_G = 17.9 ≈ 20 min. So a full-range, 5–95% B gradient of 20 min on a 150 mm × 4.6 mm, 5-µm column operated at 2 mL/min should give k*≈ 5. This isn't excessively long to wait to get "good" chromatography on the first injection of an unknown sample. From this we'll get an idea of how complex the sample is, and, as we'll see below, we can use this information to take the next step in method development.

If other sizes of columns are used, and at other flow rates, the recommended gradient times will be different. I have shown estimates of the gradient times for several typical run conditions in Table I (as usual in such presentations, the values have been rounded for display purposes, so your calculations will vary slightly from those in Table I). Just a warning here — the estimation of k* in equation 1 is just that, an estimation. Some assumptions and simplifications have been made to make it easy to use, yet still give reasonable results. As a consequence, don't get tied up in too many decimal places — a rounded, whole-number value for the gradient time is recommended. If you want more details and more exact values, consult reference 1.

Gradient or Isocratic?

Where to from here? The first use of the results of our scouting run can be to help make a decision about whether or not an isocratic run can be used. Let's look at a couple of possible chromatograms from our scouting runs. One technique to help make the isocratic or gradient choice is what I call the 25/40% rule. This states that if the peaks of a full-range gradient scouting run occupy <25% of the total run time, an isocratic method can be generated. Or if the peaks occupy >40% of the run time, a gradient will be necessary. Between 25% and 40%, either type of run may be appropriate, with the decision influenced by the complexity of the sample. The 25/40% rule is not hard-and-fast, but it gives us a simple guideline that is useful for evaluating scouting gradients. Let's look at a couple of examples of this in the simulated chromatograms of Figure 1.

Figure 1: Simulated chromatograms for gradient scouting runs using a 150 mm ՠ4.6 mm column run at 2 mL/min with a 5–95% B gradient over 20 min.

In Figure 1, both chromatograms are based on the 150 mm × 4.6 mm column discussed above, operated with a gradient of 5–95% B over 20 min at a flow rate of 2 mL/min. To determine the percent-range of the peaks, note the retention times of the first and last peaks and divide by the run time. For Figure 1a, the first peak has a retention time of 13 min and the last peak comes off at 17 min. So (17–13)/20 = 20%. This is less than the 25% cutoff for our 25/40% rule, so an isocratic method should be possible. We'll discuss how to move to isocratic conditions a little later. For the chromatogram of Figure 1b, the first peak comes off at 7 min and the last at 16 min. So (16–7)/20 = 45%. This is more than the 40% cutoff, so a gradient method would be the best choice here.

What Next?

Next, let's see how to convert the gradient scouting run into a more useful chromatogram. First, let's consider the isocratic case of Figure 1a. One popular technique is to estimate the mobile-phase composition at the midpoint of the eluted peaks and use it for the next isocratic step. In the example of Figure 1a, the midpoint between the first and last peaks is 15 min. If we assume a 0–100% gradient over 20 min, this is a 5%/min gradient. This means that a 15-min peak would be eluted at 15 × 5 = 75% B. You could fine-tune this estimate if you wanted by using the actual gradient (90%/20 min), and correcting for the fact that the gradient starts at 5% B. Also, the peak is eluted at the mobile-phase composition at the end of the column, not that at the inlet, so this is another adjustment to make. You can read about such adjustments in reference 1 if you want more information.

Rather than go to all the work of refining the estimates of the appropriate isocratic conditions, I prefer to take advantage of a free calculator that you can access from the LC Resources website (2). I've filled in the blanks for the present example in Figure 2. The column dimensions (in centimeters, not millimeters), flow rate, gradient range, gradient time, and retention times of the first and last peaks are obvious. The dwell volume, sometimes called the gradient delay or mixing volume, is a characteristic of each LC system and should be measured at some point for best results.

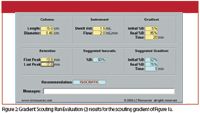
Figure 2: Gradient Scouting Run Evaluation (2) results for the scouting gradient of Figure 1a.

But for the present discussion, we can estimate the dwell volume as ≈ 1.5 mL for a conventional high-pressure mixing system, 2.5 mL for a conventional low-pressure mixing system, 0.4 mL for a high-pressure mixing UHPLC system or one tuned for use with a mass spectrometric detector (LC–MS), or 1.0 mL for a low-pressure mixing UHPLC or LC–MS system. I've used 1.5 mL in the current example, assuming a conventional high-pressure mixing system was used for the initial scouting gradient.

The results of calculations based on the input values (yellow) are shown in blue. You can see that an isocratic run is recommended, so our choice was correct. However, it recommends 60% as the target %B for the first isocratic run. This is quite a bit less than the 75% we guessed at above. This is because the calculator takes dwell volume, the gradient lag, and column volume into account. I suggest rounding the %B recommendation to the nearest 5% and trying it. Because the calculator bases its predictions on an "average" compound, it is not likely that it will be perfect on the first try. However, it is easy to inspect the actual 60% B run and make adjustments. Usually, you will be within 5% B or so of the necessary mobile phase to give retention in the 1 < k < 20 region. If peaks come out a bit early, add 5% more of the aqueous phase; if retention is a bit late, add 5% more organic. You can see that just two runs — one gradient and one isocratic — will get you to the fine-tuning stage of isocratic method development. This is likely to be much faster than the 90%, 80%, 70% . . . technique.

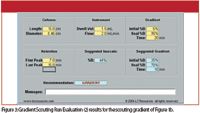
Figure 3: Gradient Scouting Run Evaluation (2) results for the scouting gradient of Figure 1b.

What about the example of Figure 1b? The results from the calculator are shown in Figure 3. As expected, a gradient is recommended, but notice that the suggested gradient conditions for the next run are different than the originals. The original run was 5–95% B in 20 min, but the calculator suggests 25–70% B in 10 min. This is because it has automatically trimmed off some of the wasted time in the scouting run. It recognized that nothing was happening until the first peak came out at 7 min and that there wasn't much point in continuing the gradient after the last peak was eluted. Therefore, it suggested to increase the starting conditions from 5% to 25% B and to stop the gradient at 70% rather than 95%. But that is all that should be changed at this point — we would like the chromatogram to look the same in all other ways. To have this happen, the k* values for the new gradient should match those for the original gradient. This is simple to do based on equation 1. We changed Δ%B from 0.95–0.05 = 0.9 to 0.70–0.25 = 0.45. To keep k* constant, some other factor must be adjusted by the same 0.45/0.9 = 0.5 ratio. The gradient range is in the denominator, so we could change either the flow rate or the gradient time in the numerator of equation 1 to compensate. You can see that changing from a 20-min gradient to a 10-min gradient will do this, and k* will remain constant. The new gradient should have the same peak spacing as the original, because k* is constant, but should not have as much wasted time at the beginning or end. Make the next run under the recommended conditions, then you can make further adjustments in the conditions to continue method development.

Summary

We have seen that whether you desire a final isocratic method or a gradient, starting with a simple scouting gradient can be the fastest way to get to your goal. The scouting run will give you an idea of the complexity of the sample, it will take a predetermined time, and the results will help you decide if an isocratic run is possible or if a gradient will be required. Finally, you can use the results of the scouting gradient to help determine the isocratic or gradient conditions for the next method development run. This can be based on manual calculations, or more easily on a simple calculator that can be downloaded for free (2).

References

L.R. Snyder and J.W. Dolan, High-Performance Gradient Elution (Wiley Interscience, New York, New York, 2007).

Gradient Scouting Run Evaluation calculator, scouting_gradients.xls found at http://www.lcresources.com/resources/resxchange.html.

John W. Dolan "LC Troubleshooting" Editor John Dolan has been writing "LC Troubleshooting" for LCGC for more than 25 years. One of the industry's most respected professionals, John is currently the Vice President of and a principal instructor for LC Resources, Walnut Creek, California. He is also a member of LCGC's editorial advisory board. Direct correspondence about this column via e-mail to John.Dolan@LCResources.com.