Current and Future Chromatographic Columns: Is One Column Enough to Rule Them All?

The vast majority of separations in liquid chromatography (LC) still use the typical packed particle bed format, most commonly with fully or superficially porous particles in particle sizes as low as 1.3 µm. As an alternative, monolithic columns have been the topic of many studies, but they are currently used only in some niche applications. Research into perfectly ordered microfabricated columns has shown tremendous possibility for these high performance columns for use in nano-LC, but their development is still ongoing. The possibilities that emerging three-dimensional (3D) printing technology offers make it theoretically possible to develop any imaginable structure with high precision, but the technology is currently limited. This article provides a critical review of all these technologies and demonstrates how further development of chromatographic columns will be of paramount importance in the future.

The improvements in instrument and column performance in liquid chromatography (LC) over the past 15–20 years have resulted in an almost 10-fold reduction in analysis time and threefold increase in separation efficiency. Nevertheless, the complexity of the samples emerging in life sciences (proteomics, metabolomics, lipidomics, and so forth), containing 10,000 or more analytes in a wide range of concentrations and physicochemical properties (including size, polarity, and ionization state) is so vast that it is impossible to even dream of ever achieving full resolution using one-dimensional chromatography with the present state-of-the-art instrumentation and columns. The rise of two-dimensional (2D) separation techniques, in combination with modern tandem mass spectrometry (MS/MS) systems, vastly increases the overall resolving power that can be achieved (1–4). Nevertheless, even 2D separations are ultimately limited by the efficiency of the chromatographic column in the individual dimensions. In addition to the need for enhanced resolution, a further increase in separation speed for the second-dimension column is in high demand as well because this would allow the sampling rate in the first dimension to increase, better preserving its resolution.

(IMAGE CREDIT: JOE ZUGCIC, ZUGCIC PHOTOGRAPHERS.)

The further development of faster and more efficient LC chromatographic columns thus remains of paramount importance in the years and decades to come. In addition, the chromatographic instrumentation will need to follow these improvements and changes in the performance and format of chromatographic columns (5).

Overall, the main factors that determine the separation power and speed of any system are given by the Knox and Saleem equation (6,7), determining the time, t, needed to achieve a given number of theoretical plates, N, under fully optimized kinetic conditions and for a given maximum pressure, ΔP_max (which can be either column or instrument limited), and mobile phase viscosity, η:

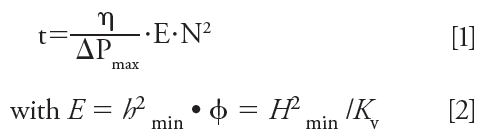
with H_min and h_min the minimum absolute and reduced plate height, respectively, and K_v and ϕ the hydraulic permeability and the flow resistance of the bed, respectively. The importance of the instrumentation (pressure limit, extracolumn dispersion) and the effect of mobile phase viscosity (LC, gas chromatography [GC], and supercritical fluid chromatography [SFC]) in this equation were discussed earlier (5,8). The focus of the present contribution is on the factors grouped in the so-called separation impedance E (6,7), determining the column quality. E is a dimensionless number and hence is independent of the size of the support. It only depends on its shape. In fact, the E number represents the ability of a given chromatographic support shape to transport the mobile phase through the column with a minimum of dispersion and pressure losses. The smaller the value of E, the smaller these losses, and hence the shorter the time needed to achieve a given N. By far the most important geometrical factor determining the value of E is the external porosity ε, essentially because of its effect on the flow resistance. Computational fluid dynamics simulations on idealized monolithic support structures with varying ε have revealed that, while the domain-size based h does not vary much when ε increases, ϕ can easily decrease with an order of magnitude when the structure becomes more open and, for example, increases from ε = 40% (equal to the porosity of the packed bed of spheres) to say ε = 85% (9). As a consequence, also E and the associated separation time (equation 1) can be expected to drop by an order of magnitude. For an increase in ε = 40% to ε = 60%, ϕ is about three times smaller, offering threefold faster separations than with a packed bed. However, to benefit from the shorter analysis times via the reduction of E with increasing porosity, this increase should be accompanied by a significant decrease of the support size. If not, the optimal N value for which equation 1 holds increases as well, leading to longer analysis times. Finding ways to simultaneously increase ε and decrease the size of the support elements, while maintaining a good structural homogeneity, mechanical strength, and sufficient retention surface, is the key to realizing a paradigm shift in the speed and performance of LC columns.

Packed-Bed Columns

The vast majority of chromatographic columns sold nowadays are filled with fully or superficially porous particles (10). These columns show excellent reproducibility in both performance and selectivity and are available from capillary up to (semi-)preparative scale in a wide range of lengths, packed with different particle sizes and stationary phase chemistries. Whereas h_min = 2 was long considered the practically achievable lower limit for column efficiency for analytical columns (2.1–4.6 mm i.d.), the new generation of superficially porous particles (SPPs) allows us to achieve h_min values as low as 1.4. Spurred by these developments, attempts have been made to produce fully porous particle batches with a reduced particle size distribution, such that nowadays h_min values as low as 1.7 can be achieved with fully porous particles (11–14). It should be noted that in capillary formats, h_min values down to h_min = 1 have been demonstrated in research laboratories (15).

The fact that particles in a packed bed need to be in contact with each other to obtain a stable and pressure resistant bed means that the external porosity ε of a randomly packed bed is always around 36–40%. As a consequence, the flow resistance ϕ₀ of packed beds is difficult to alter or optimize and usually lies between 600 and 800 (16,17). In a first approximation, ϕ₀ can be calculated according to Kozeny-Karman's law (based on the u₀ velocity of an unretained t₀-marker):

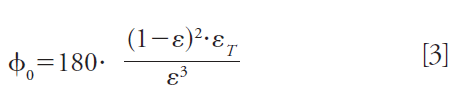
Since ϕ₀ not only depends on ε but also on the total porosity ε_T, a clear reduction of the flow resistance is obtained when switching from fully porous to superficially or nonporous particles (lower total porosity ε_T than fully porous particles). However, the effect is rather small and difficult to exploit because a reduction of the porous zone fraction of the particles reduces the sample loadability, causing efficiency loss when a large sample mass is injected (18).

The only way to further improve the kinetic performance of packed-bed columns would thus be a further reduction in h_min. The latter can be highly effective, given the quadratic variation of E with h_min. As a result of this quadratic dependency, the seemingly modest reduction of h_min by some 20–30% that is typically observed when moving from fully porous to superficially porous corresponds to a very significant twofold reduction in analysis time. Using typical values for h_min and ϕ₀ for superficially porous (h_min = 1.5, ϕ₀ = 600) and fully porous (h_min = 2, ϕ₀ = 800) particle columns, E values are around 1350 and 3200, respectively. These values are in good agreement with experimental results (17,19).

Further improvements in packing heterogeneity, reducing the so-called eddy dispersion contribution (A term), are thus of high interest. As recently discussed by Gritti and colleagues, perfectly ordered packed beds (A term = 0) are expected to yield h_min values equal to 0.9, 0.7, or 0.5 for fully, superficially, and nonporous particle columns, respectively, because it is impossible to eliminate longitudinal diffusion and mass transfer resistance contributions (13). Finding ways to further suppress the eddy dispersion while sticking to the traditional slurry packing methods seems to be rather difficult (Figure 1a), if not impossible, given the many efforts already devoted to the problem in the past decades (13). It seems that radically novel packing methods are needed. One approach, currently under investigation in our group, would be the use of additive layer manufacturing, where ordered layers of monodisperse silica particles are assembled layer by layer to form a three-dimensionally (3D) printed particle column (Figure 1b). However, this concept is still far from reality. Besides the cost efficiency, one critical aspect is the pressure stability of these beds under the very high operating pressures (up to 1500 bar) nowadays available in commercial ultrahigh-pressure liquid chromatography (UHPLC) equipment.

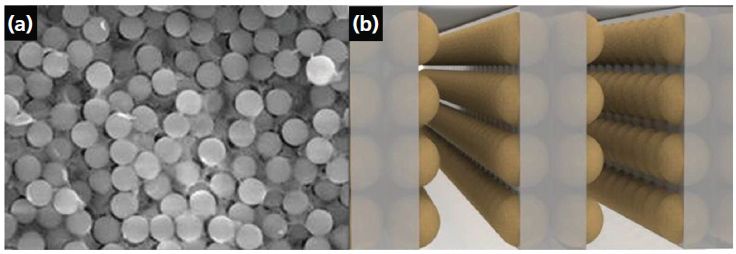
Figure 1: (a) Scanning electron microscope (SEM) image of monodisperse particles in a random sphere packing showing the inherent packing heterogeneities. (b) Artist's impression of a packed bed column made using additive layer manufacturing, with monodisperse particles assembled layer by layer to form a 3D printed particle column.

An approach to lower the h_min of packed bed columns that appears closer to reality (given the existence of an experimental proof delivered by Wei and colleagues [20]) is the production of core–shell particles wherein the mesopores are oriented purely radially instead of forming a randomly connected network. Although this difference seems only a small change, it has such a strong effect on the B term that it can be expected to lead to a further reduction of 0.5 reduced h units compared to the conventional core–shell particle performance (20,21).

Monolithic Columns

Instead of stacks of individual particles as in packed bed columns, monolithic columns consist of a continuous porous skeleton with large through-pores (Figure 2). In the early 1950s, the potential of this column format was discussed by Nobel Prize Laureates Martin and Synge (22). The in situ synthesis of monolithic materials has several advantages, including the absence of frits to retain particles in the column and a facilitated development of miniaturized column formats, such as capillaries (Figures 2a and 2c) and microfluidic chips (Figure 2e). Also the use of thin monolithic layers to obtain a retentive porous layer for use in open-tubular (Figure 2d) or pillar-array devices (Figure 2b) has been demonstrated (23–25). In principle, monolithic stationary phases have the potential to outperform packed columns. Whereas the efficiency of packed columns is related to the particle size while the total porosity and thus flow resistance is fixed, the use of porogenic solvents in the preparation of monolithic materials facilitates the optimization of the globule size or skeleton (almost) independently of ε. The advantage that ε can be made very large (values up to ε = 86% have been reported for use in LC [26]), makes them intrinsically much better suited to obtain small E values and a correspondingly improved kinetic performance. Silica-based capillary monolithic columns, for example, have been shown to produce E values as low as 300 (27). This quantum leap in E is entirely because of the lower flow resistance of monolithic columns (in turn a direct consequence of their higher external porosity ε), because monolithic columns can at best (that is, when they are produced with similar degrees of eddy dispersion) be expected to produce about the same (domain size-based) h_min value as packed bed columns (see the small effect of ε on h_min in Figure 10 of reference 9).

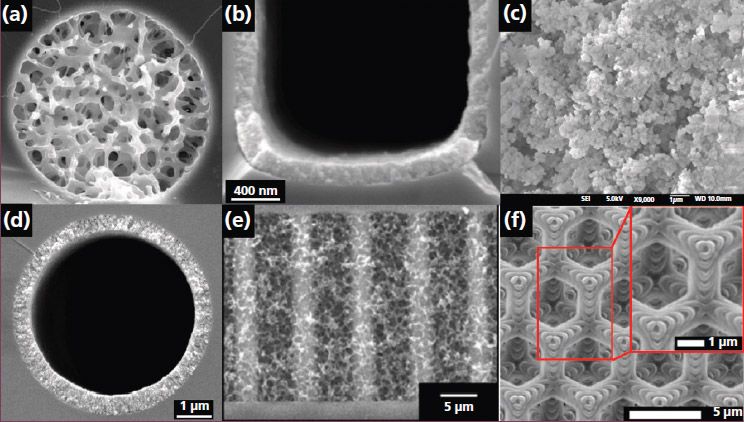
Figure 2: SEM images of a (a) silica monolithic column, (b) silica monolithic layer deposited on REP column (24), (c) polymer monolithic column, (d) silica monolithic layer deposited on capillary column for use in open-tubular LC (23), (e) silica monolithic column synthesized in pillar array column (25), and (f) 3D printed monolithic column. Figures adapted from references 23–25 with permission.

However, an advantageous shape and a concomitantly low E number is not everything. The absolute size of the support also matters. Here the rule is very simple: instead of creating a large external porosity by increasing the size of the through-pores, the latter should be kept constant (or even made smaller) to keep the same mobile to stationary zone diffusion distances. The only way to achieve the required high external porosity then consists of shrinking the size of the structural elements. However, this approach brings about a number of problems that seem so difficult to solve that they currently impede the success of monolithic columns. By far, the most tenacious problem in this respect is the so-called small domain size limit (28,29). This problem originates from the fact that each monolith synthesis process inevitably displays a local variability on the size and position of the produced solid zone elements, which are at best absolute in size. This variability implies that the general heterogeneity of the structure will increase when smaller feature sizes are being pursued, putting a fundamental limit on the possible feature size reduction of monolithic columns.

Polymer Monolithic Columns

Whereas early forms of (gel-like) polymer monolithic materials collapsed when pressure was applied, rigid polymer-based monolithic materials (Figure 2c) that are compatible with high-pressure operation have been available since the 1990s (30,31). The two most prominent classes of materials are the poly(styrene-co-divinylbenzene)-based materials and monolithic entities based on methacrylate ester–based precursors. Polymerization mixtures are typically prepared from mono- and oligovinylic monomers and an initiator in the presence of an inert diluent, called porogen. The porogen, typically a binary solvent mixture, is selected based on its ability to dissolve the monomers, yielding a homogenous solution. During the course of the polymerization reaction microgel particles are formed, following interparticle reactions via pendant vinyl groups leading to the formation of microgel clusters (32). Ultimately, a microscopic porous network is formed, and a phase separation occurs. Details of how the reaction conditions affect the size of the microglobules and resulting macropore structure can be found in the literature (33,34). To advance the kinetic performance of monoliths, Vaast and colleagues described the development of nanostructured high-porosity monolithic supports allowing for sub-minute peptide separations (35). Furthermore, Vaast linked the effects of macropore and microglobule size, and structure homogeneity, to the separation performance measured in gradient elution, both in terms of peak capacity and gradient plate height (35).

Polymer-monolithic stationary phases have emerged as an attractive alternative for packed columns in the field of biomolecule separations, and their potential has been demonstrated for a wide range of biomolecules (36,37). In reversed-phase gradient mode, ultrafast separations (<1-min gradients) of intact proteins have been realized in both large internal diameter columns and using capillary column formats (35). Using a 250-mm-long capillary monolithic column and applying a 2-h gradient, intact proteins, including protein isoforms arising from various amino-acid modifications, were resolved yielding a maximum peak capacity of 650 (38). Figure 3 shows the separation of an E. coli digest using a 1-m monolithic column yielding a peak capacity in excess of 1000 (39). To further extend the kinetic performance and applicability of monolithic columns, different innovative approaches are currently being explored, such as composite cryopolymers (40) or the incorporation of nanoparticles to extend monoliths with only reversed-phase functionalities to ion exchange (41). These nanoparticles might also act as structure directing agents to improve kinetic performance.

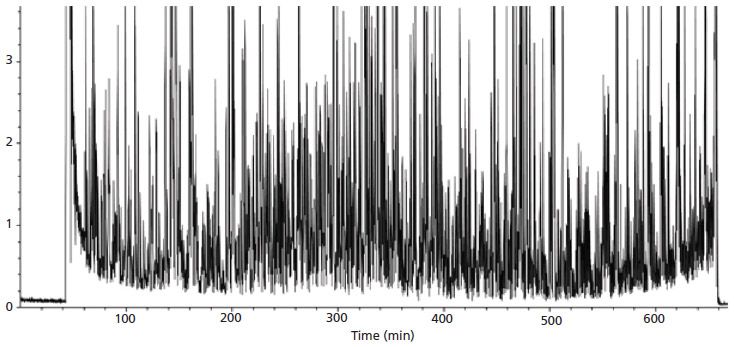
Figure 3: High-resolution LC–MS/MS analysis of a tryptic digest of E. coli obtained on a 1-m long monolithic column. Adapted with permission from reference 39.

Although excellent results can be obtained for the separation of larger biomolecules, the plate numbers achieved for small molecules on polymer-monolithic columns are typically one order of magnitude lower than those obtained on classical packed columns. Whereas the C18 layer on modified silica particles is extremely thin and hence the diffusion distance is short, it has been speculated that small molecules can penetrate into the polymer globules of monolithic materials, and excessive dispersion is a result of "surface diffusion" (42).

Silica Monolithic Columns

Silica monoliths (Figure 2a) are produced via a sol-gel process wherein alkoxysilanes are hydrolyzed and then polycondensed in the presence of a water-soluble porogen (43,44). Siloxane oligomers formed during successive condensation reactions link together to form a gel network. Spinodal decomposition occurs and phase separation takes place between the silica-rich and solvent-rich phase, forming the future silica skeletons and through-pores, respectively. Similar to polymer monoliths, the phase separation and the pore size of the gel are controlled by varying the concentration of the porogen. The stiffness and strength of the gel are increased by aging in a siloxane solution, and mesopores are formed by adding ammonium to the aging solution. Finally, the gel is dried and clad with polyether ether ketone (PEEK) to obtain a silica monolith suitable for chromatographic purposes. This column housing, however, limits the maximum operating pressure in analytical scale columns to high performance liquid chromatography (HPLC)-like operating pressures (generally below 400 bar), while it has recently been shown that the silica monolithic skeleton itself can withstand pressures up to at least 800 bar (45).

For analytical scale monoliths (2.1–4.6 mm i.d.), through-pore sizes are typically d_tp = 1–2 µm and high external porosities (ε > 60%) are obtained. Because of their intrinsic high permeability, silica monoliths can be operated at high linear velocities, or in long (coupled) columns, resulting in extremely high efficiencies (46). The small size of the silica skeletons (typically d_skel = 1–2 µm) results in efficiencies comparable to those obtained in columns packed with 5-µm particles, especially when operated at high flow rates (47). However, because of the poor radial homogeneity-which can be related to their fabrication process, concomitant high eddy dispersion, and their limited pressure resistance-silica monoliths (48) have not been able to compete with the particle-packed columns (sub-2 µm or sub-3 µm core–shell) that were developed around the same time (49–51). To improve their performance, efforts have been made to improve the radial homogeneity while at the same time reducing their feature sizes by adjusting the preparation process (for example, concentration and porogen type). This improvement has resulted in the introduction of the so-called second generation of silica monoliths (27,52,53). Because of their improved radial homogeneity and reduced skeleton (d_skel < 1 µm) and through-pore sizes (d_tp = 1.1–1.2 µm), H_min values are much lower compared to the first generation, and comparable to what can be obtained in 3–3.5 µm particle packed columns (54). The downside of these reduced feature sizes is that the permeability of the monolithic column decreases accordingly, from K_v0 = 4.7 × 10^-14 m² and K_v0 = 4.0 × 10^-13 m² for the first generation (26,27,55) to significantly smaller values K_v0 = 2.0 × 10^-14 m² for the second generation (55). According to Deridder and colleagues, the permeability of a silica monolith is directly related to the square of its skeleton size, while a more complex relation between permeability and external porosity exists, depending on the geometry of the monolith (56). Considering that external porosity values measured for first- and second-generation monoliths are largely the same, the decreased permeability of the second-generation monoliths must therefore mainly be attributed to the reduced skeleton and through-pore sizes (55). Nevertheless, the lower permeability of the second-generation monoliths is still well above those measured for sub-3-µm particle columns.

Comparing silica monoliths with packed-bed columns, similar E values (at the lower end of the range) as for fully porous particles columns are found, with E = 2200–4600 and 2200–3400 for the first and second generation, respectively (55). To compare the separation power for a given separation problem, the kinetic plot method is a useful alternative to the impedance because it represents the maximum plate count obtainable in a certain analysis time. Figure 4 compares the kinetic performance for first and second generation monoliths with ΔP_max = 200 bar. It is clear that second-generation monoliths perform better (1.5–2.5x faster for a certain N) than the first generation monoliths for N < 50.000. For more challenging separations (N > 50.000), the first-generation monoliths perform better because their large permeability allows them to be used in longer columns without compromising the separation speed (55). Comparing their performance with a 2.7-µm superficially porous particle packed column, operated at its own ΔP_max = 600 bar, shows that the SPP-based column outperforms both generations of monoliths over the entire range of practically relevant plate counts. A similar conclusion can be drawn when comparing silica monoliths with sub-2-µm particle columns with ΔP_max = 1000–1500 bar (57). Further improvements in the structural size and homogeneity of the silica monoliths by improving their production process, together with the development of higher pressure–resistant material to clad the monolithic columns, are required before the monolithic columns can become competitive with the current state of the art in particle-packed column technology.

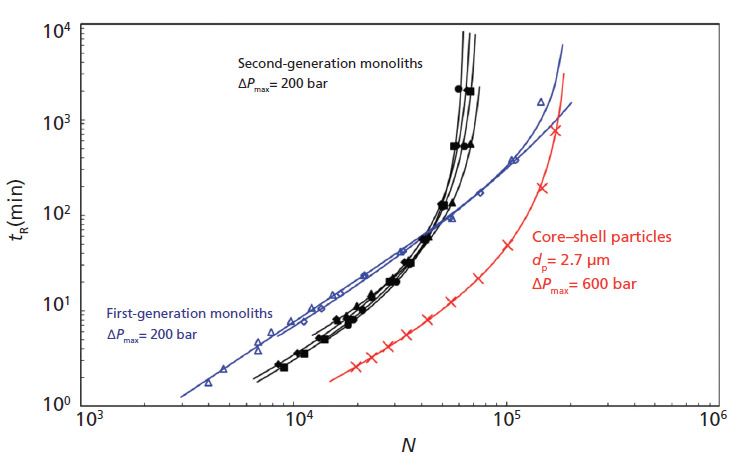
Figure 4: Kinetic plots of analysis time (t_R) versus plate count (N) for benzophenone and for first-generation monoliths (⋄ and Δ) and second-generation monoliths (◼ ♦ • ▴) evaluated at 200 bar. Open, blue symbols refer to first generation monoliths, closed, black symbols refer to second generation monoliths. The red curves (×) are obtained for a core-shell column (100 mm × 2.0 mm, d_p = 2.7 µm) operated at a maximum pressure of 600 bar. The mobile phase was adapted on all columns to obtain k = 8.7 for benzophenone. Adapted with permission from reference 55.

In capillary formats, the radial variation in external porosity (and hence in flow resistance) caused by the inevitable post-synthesis shrinking process is much smaller than in analytical bore columns. Producing silica monoliths with a domain size of about 2 µm in 100-µm columns, H_min values as low as 4.1–4.4 µm have been demonstrated (58). However, these values are still around two times larger than the lowest H_min ever reported for packed bed columns (H_min = 2 µm when using 1.3-µm core–shell particles) (59). This discrepancy shows that a further significant decrease of the domain size of silica monolithic columns is still needed.

Microfabricated Columns

Pillar-array columns were introduced in 1998 by Fred Regnier and coworkers as an ordered alternative to disordered chromatographic packings (60,61). Because the packing was originally intended for capillary electrochromatography separations requiring nonconducting substrates, the first experiments were carried out using columns produced in fused silica and polydimethylsiloxane (PDMS). Because these substrates do not easily allow fabrication of pillars with sidewall slopes close to 90°, the substrates had to be replaced with silicon before the predicted absence of eddy dispersion in the perfectly ordered structures were indeed reflected in the measured van Deemter curves. The first reversed-phase separations on silicon micropillar arrays were reported in 2007 (62), showing plate heights as low as 4 µm for retained components in a nonporous pillar bed. These initial results were obtained by measuring the band broadening in the center of the beds-that is, by excluding the sidewall region where the flow resistance of the bed was different from that in the rest of the bed. Using computational fluid dynamics simulations this problem could be solved and appropriate designs for the sidewall region were proposed (63). One particularly useful solution were radially elongated pillars (REP, Figure 5g) having a lateral-to-axial aspect ratio larger than 10 (64–66). The use of (at least a number of rows of) such REP structures in the flow distributors (Figure 5f) at the inlet and outlet sections of the bed also proved to be essential to interface the columns with the outer world (67). These distributors were also key to producing sufficiently long columns on the (relatively limited) surface of a silicon wafer, connecting different channel tracks using low dispersion turns (Figure 5b). An interesting alternative approach was implemented by Isokawa and colleagues (68), who designed a dedicated curve and pillar bed with varying density to minimize dispersion, while at the same time increasing permeability in the turn zone.

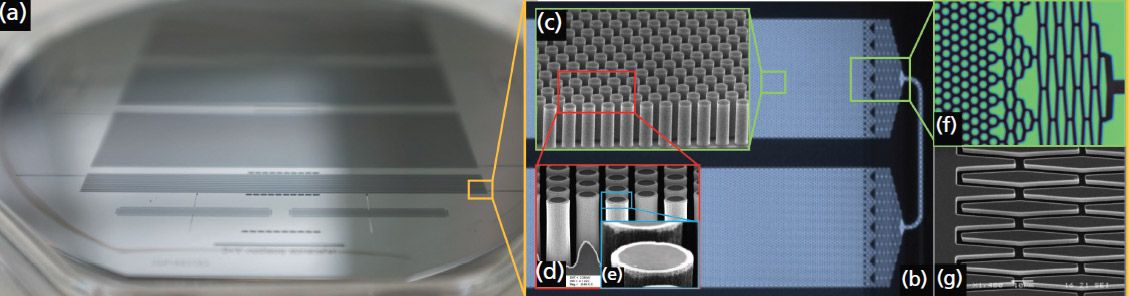
Figure 5: (a) Silicon wafer featuring several pillar-array columns, with enlarged views of (b) turn structures at the end of each channel to increase total column length, (c) cylindrical pillars making up the chromatographic bed, (d) anodized pillars to increase retentive surface (67), (e) detail of porous shell (67), (f) channel inlet flow distributor, (g) alternative bed structure with radially elongated pillars (65). Figures 5d–5e were adapted from reference 67 with permission from the Royal Society of Chemistry and Figure 5g was adapted from reference 65, with permission.

In 2017, the first generation of pillar-array columns was introduced, consisting of a 2-m-long pillar bed with 5-µm-diameter pillars, spaced 2.5 µm from each other (width 315 µm, depth 18 µm, porous layer of 200 nm) (Figures 5a–5c). This column has the permeability of a 10-µm packed bed (that is, 2.7 × 10^-13 m²), while it produces plate heights comparable with 3–4 µm porous spherical beads (that is, 5–7 µm) (67). The corresponding E number is on the order of 50–100-that is, more than an order of magnitude less than in packed bed columns. The pressure tolerance of more than 400 bar would even allow one to construct columns with lengths of more than 10 m, producing more than 1.5 million plates under retained conditions (small molecules like phenones) in about 12 h. A lot of effort was put in the conformal integration of porous layers into the chips to increase the specific surface of the nonporous pillars. Two methods that additionally allow tuning of the pore geometry have been developed to this end and have been applied for 2.5-µm pillar spacings (24,69). With electrochemical anodization (silicon) pores are grown inside the silicon pillar (Figures 5d and 5e), leaving the contour of the pillar unaltered (69), whereas with the sol-gel deposition technique a porous glass layer is grown on the pillar (Figure 2b), thereby increasing the size of the pillar (24). For some applications, such as ion-pair reversed-phase chromatography of nucleotides (70) or hydrodynamic chromatography (71), it is actually preferred that the pillars are nonporous (Figure 5b) (72). In this case, the plate count roughly doubles (73). These columns have an equivalent cylindrical diameter of around 80 µm, which is a typical targeted diameter to achieve optimal flow rates for electrospray ionization (ESI)-MS detection. These column features make the first generation of commercial pillar-array columns extremely suited to achieve peak capacities of >1000 in the nano-LC flow rate range.

Since this first generation still uses relatively large pillars (5 µm), great improvements in both speed and efficiency can be expected when new generations will be produced with pillar diameter and spacing of the same order as the current sub-2-µm particles used in packed bed columns. Technologically this approach is feasible, given that the Bosch etching technology has the potential to achieve even submicrometer resolution (63). Sidewall effects might appear again, but they could be countered by using REP structures that are insensitive to this effect (65).

3D-Printed Columns

In the last decade, almost every field in scientific research has started to use 3D printing or additive manufacturing. Compared to traditional manufacturing technologies, 3D printing offers the possibility to use the full three-dimensional fabrication potential to freely tune and fabricate any favorable geometry. For applications in chromatography, 3D printing especially holds the promise of providing a way to produce perfectly ordered structures, thus allowing one to eliminate any eddy-dispersion contributions. Similar to microfabricated columns, the external porosity and thus the flow resistance can freely be tuned within the range where mechanically stable structures can be printed. Combining 3D printing with computational fluid dynamics simulations can lead to the design of "fully optimized" stationary phases having limited diffusion and a low resistance to mass transfer. Another asset of 3D printing is the fast manufacturing of prototypes, making rapid iterative device development possible. The use of additive manufacturing should not be limited to stationary phases, because complete chromatographic columns (containing stationary phase, column wall, flow inlet, and frits) can be fabricated simultaneously. Combining 3D-printed columns with printed valves, micropumps, connectors, and other microfluidic parts (74), one can even dream of producing a complete 3D-printed chromatographic system on a chip.

The emerging possibilities of 3D printing have already reached the field of HPLC as could be seen at the latest HPLC conferences. In 2016, Brett Paul and coworkers showed the possibilities to fabricate 3D metal printed chromatographic columns, which were functionalized in situ with a thermally polymerized monolith (75). Instead of using 3D printing to obtain a scaffold on which a stationary phase is deposited, the whole column, including walls, can be printed in one step, as shown by Fee and colleagues (76). They exploited the full 3D potential of additive manufacturing, printing both octahedral beads in a simple cubic configuration (apothems of 113.6 ± 1.9 µm), and monolith hexagonal channels, both in parallel and herringbone arrangements (apothems of 148.2 ± 2.0 µm). In 2017, Fee's group printed a broad range of particle shapes including tetrahedral, octahedral, stellar octangular, triangular bipyramid, and truncated icosahedra particles, in different geometric arrangements as simple cubic, body-centered cubic, and face-centered cubic (77). However, for 3D printing to become the new standard of column manufacturing, some significant disadvantages need to be overcome. The most important hurdle is the resolution or minimal printed feature size, which is about 25–100 µm for the most widespread technologies, such as extrusion-based printing (fused deposition modeling), stereolithography (stereolithographic apparatus, digital light processing), and powder-based printing (selective laser melting, selective laser sintering, inkjet-based printers) (74). State-of-the art packed bed column technologies with, for example, sub-2-µm particles, have feature sizes (flow-through pores) on the order of 500 nm and below. Currently, only one additive manufacturing technology exists that offers competitive resolutions, namely two-photon polymerization printing (2PP). Nevertheless, other 3D-print technologies with lower resolution can be applied for the manufacturing of preparative columns, not being so demanding toward micrometer-scale feature sizes. A 2PP printer operates by emitting near-infrared (NIR) femtosecond pulses of photons into a photopolymerizable resin. Two photons need to be simultaneously absorbed to initiate radical polymerization, a process so rare that it only takes place in the focal spot size of the laser, leading to extremely small voxel (volume-pixel) sizes. With minimal feature sizes smaller than 50 nm (78), stationary phases with characteristic distances of 1 µm can thus be manufactured with high accuracy (see Figures 2f and 6). A drawback of 2PP, however, is the trade-off between high resolution and printing time or volumes. Whereas new structures can be quickly prototyped in manifold with all other 3D-print technologies, the speed of production with 2PP still has a long way to go. The manufacturing of a structure as shown in Figure 2f (ε = 80%, edges of 1 µm, through-pores of 1.5 µm) for a 1-cm-long column with a width of 100 µm and depth of 10 µm (equivalent to a 40-µm i.d. capillary) takes almost 1 day to print. It needs to be mentioned that printing time is highly influenced by the spatial dimensions, structure, material choice, laser objective, writing direction, and many other parameters. In addition, 2PP is much more expensive than the more widespread technologies.

Figure 6: Example of a 3D-printed tetrahadron skeleton model type bed (zoom: see Figure 2f).

Another important hurdle is the material choice, as the final obtained structure needs to be temperature and solvent resistant (such as no swelling). In addition, these materials will have to be functionalized to obtain an appropriate retentive surface chemistry (for example, C8, C18, phenyl, amide), to such an extent that no undesired interactions are possible with the starting material. Because typical 2PP printed materials have no inherent porosity, either the printed structure will need to be modified to create mesopores or a porous layer will need to be deposited on the surface of the printed structure to obtain a sufficiently high retention surface.

Conclusions

Despite the many research efforts on novel column technologies, packed columns are still the first choice in liquid chromatography. Monolithic columns are used in rather niche applications or for their flexible applicability and possible in situ generation in complex geometries. Further improvements in the production process of the silica-gel monoliths should aim at reducing the domain size further while maintaining or further improving the homogeneity before they can ever become competitive with the current state-of-the-art particle-packed columns. The development of a suitable ultrahigh-pressure column housing is another issue. Polymeric monolithic columns show great potential for the separation of large molecules, including biomolecules, but have inherent disadvantages for small-molecule separations. The first generation of microfabricated pillar arrays shows promising results for separations that require high resolving power, but reduced feature sizes are required to further enhance separation speed and efficiency. Three-dimensional printing shows a tremendous intrinsic potential for the fabrication of chromatographic columns, but the current printing hardware either does not allow one to obtain the desired submicrometer resolution or the printing time is too long, limiting the technology at this moment to either preparative scale separations or the development of chip-scale devices. These novel developments clearly show that we have not yet reached the end of column development and that further improvements can be expected in upcoming decades.

References

(1) D.R. Stoll and T.D. Maloney, LCGC North Am. 35, 680–687 (2017).

(2) D.R. Stoll, K. Shoykhet, P. Petersson, and S. Buckenmaier, Anal. Chem. 89, 9260–9267 (2017).

(3) M. Pursch and S. Buckenmaier, Anal. Chem. 87, 5310–5317 (2015).

(4) K. Sandra, M. Steenbeke, I. Vandenheede, G. Vanhoenacker, and P. Sandra, J. Chromatogr. A 1523, 283–292 (2017).

(5) K. Broeckhoven, J. De Vos, and G. Desmet, LCGC Eur. 30, 618–625 (2017).

(6) M. Golay, in Gas Chromatography D.H. Desty, Ed. (Butterworths, London, 1959), p. 36.

(7) J.H. Knox and M. Saleem, J. Chromatogr. Sci. 7, 614 (1969).

(8) S. Jespers, K. Broeckhoven, and G. Desmet, LCGC Eur. 30, 284–291 (2017).

(9) J. Billen and G. Desmet, J. Chromatogr. A 1168, 73–99 (2007).

(10) R.E. Majors, LCGC North Am. 30, 20–34 (2012).

(11) O.H. Ismail, M. Catani, L. Pasti, A. Cavazzini, A. Ciogli, C. Villani, D. Kotoni, F. Gasparrini, and D.S. Bell, J. Chromatogr. A 1454, 86–92 (2016).

(12) M. Catani, O.H. Ismail, A. Cavazzini, A. Ciogli, C. Villani, L. Pasti, C. Bergantin, D. Cabooter, G. Desmet, F. Gasparrini, and D.S. Bell, J. Chromatogr. A 1454, 78–85 (2016).

(13) F. Gritti and M.F. Wahab, LCGC North Am. 36, 82–98 (2018).

(14) R. Henry, LCGC North Am. 32, 12–19 (2014).

(15) J.M. Godinho, A.E. Reising, U. Tallarek, and J.W. Jorgenson, J. Chromatogr. A 1462, 165–169 (2016).

(16) D. Cabooter, J. Billen, H. Terryn, F. Lynen, P. Sandra, and G. Desmet, J. Chromatogr. A 1178, 108–117 (2008).

(17) D. Cabooter, A. Fanigliulo, G. Bellazzi, B. Allieri, A. Rottigni, and G. Desmet, J. Chromatogr. A 1217, 7074–7081 (2010).

(18) D.V. McCalley, J. Chromatogr. A 1218, 2887–2897 (2011).

(19) Y. Vanderheyden, D. Cabooter, G. Desmet, and K. Broeckhoven, J. Chromatogr. A 1312, 80–86 (2013).

(20) T.-C. Wei, A. Mack, W. Chen, J. Liu, M. Dittmann, X. Wang, and W.E. Barber, J. Chromatogr. A 1440, 55–65 (2016).

(21) S. Deridder, M. Catani, A. Cavazzini, and G. Desmet, J. Chromatogr. A 1456, 137–144 (2016).

(22) A. Tiselius, Disc. Faraday Soc. 7, 7–11 (1949).

(23) T. Hara, S. Futagami, S. Eeltink, W. De Malsche, G. V. Baron, and G. Desmet, Anal. Chem. 88, 10158–10166 (2016).

(24) S. Futagami, T. Hara., H. Ottevaere, G.V. Baron, and W. De Malsche, J. Chromatogr. A 1523, 234–241 (2017).

(25) F. Detobel, H. Eghbali, S. De Bruyne, H. Terryn, H. Gardeniers, and G. Desmet, J. Chromatogr. A 1216, 7360–7367 (2009).

(26) N. Tanaka, H. Kobayashi, K. Nakanishi, H. Minakuchi, and N. Ishizuka, Anal. Chem. 73, 420A–429A (2001).

(27) H. Minakuchi, K. Nkanishi, N. Soga, N. Ishizuka, and N. Tanaka, J. Chromatogr. A 797, 121–131 (1998).

(28) J. Billen, P. Gzil, G.V. Baron, and G. Desmet, J. Chromatogr. A 1077, 28–36 (2005).

(29) J. Billen, P. Gzil, and G. Desmet, Anal. Chem. 78, 6191–6201 (2006).

(30) F. Svec and J.M.J. Fréchet, Anal. Chem. 64, 820–822 (1992).

(31) Q.C. Wang, F. Svec, and J.M.J Fréchet, Anal. Chem. 65, 2243–2248 (1993).

(32) S. Wouters, T. Hauffman, M.C. Mittelmeijer-Hazeleger, G. Rothenberg, G. Desmet, G.V. Baron, and S. Eeltink, J. Sep. Sci. 39, 4492–4501 (2016).

(33) C. Viklund, F. Svec, J.M.J. Fréchet, and K. Irgum, Chem. Mater. 8, 744–750 (1996).

(34) E.C. Peters, F. Svec, J.M.J. Fréchet, C. Viklund, and K. Irgum, Macromolecules 32, 6377–6379 (1999).

(35) A. Vaast, H. Terryn, F. Svec, and S. Eeltink, J. Chromatogr. A 1374, 171–179 (2014).

(36) S. Eeltink, S. Wouters, J.L. Dores-Sousa, and F. Svec, J. Chromatogr. A 1498, 8–21 (2017).

(37) A. Premstaller, H. Oberacher, and C.G. Huber, Anal. Chem. 72, 4386–4393 (2000).

(38) S. Eeltink, B. Wouters, G. Desmet, M. Ursem, D. Blinco, G.D. Kemp, and A. Treumann, J. Chromatogr. A 1218, 5504–5511 (2011).

(39) S. Eeltink, S. Dolman, F. Detobel, R. Swart, M. Ursem, and P.J. Schoenmakers, J. Chromatogr. A 1217, 6610–6615 (2010).

(40) R.D. Arrua and E.F. Hilder, RSC Adv. 5, 71131–71138 (2015).

(41) R.D. Arrua, A. Nordborg, P.R. Haddad, and E.F. Hilder, J. Chromatogr. A 1273, 26–33 (2 013).

(42) I. Nischang, I. Teasdale, and O. Bruggeman, J. Chromatogr. A 1217, 7514–7522 (2010).

(43) K. Nakanishi and N. Soga, J. Non-Cryst. Sol. 139, 1–13 (1992).

(44) K. Nakanishi and N. Soga, J. Non-Cryst. Sol. 139, 14–24 (1992).

(45) T. Hara, S. Eeltink, and G. Desmet, J. Chromatogr. A 1446, 164–169 (2016).

(46) K. Miyamoto, T. Hara, H. Kobayashi, H. Morisaka, D. Tokuda, K. Horie, K. Koduki, S. Makino, O. Núñez, C. Yang, T. Kawabe, T. Ikegami, H. Takubo, Y. Ishihama, and N. Tanaka, Anal. Chem. 80, 8741 (2008).

(47) D.V. McCalley, J. Chromatogr. A 965, 51–64 (2002).

(48) F. Gritti and G. Guiochon, J. Chromatogr. A 1218, 5216–5227 (2011).

(49) D.T.-T. Nguyen, D. Guillarme, S. Heinisch, M.-P. Barrioulet, J.-L. Rocca, S. Rudaz, and J.-L. Veuthey, J. Chromatogr. A 1167, 76 (2007).

(50) R.E. Majors, LCGC North Am. 23, 1248 (2005).

(51) J.E. MacNair, K.C. Lewis,and J.W. Jorgenson, Anal. Chem. 69, 983 (1997).

(52) K. Morisato, S. Miyazaki, M. Ohira, M. Furuno, M. Nyudo, H. Terashima, and K. Nakanishi, J. Chromatogr. A 1216, 7384–7387 (2009).

(53) S. Altmaier and K. Cabrera, J. Sep. Sci. 31, 2551–2559 (2008).

(54) F. Gritti and G. Guiochon, J. Chromatogr A 1225, 79–90 (2012).

(55) D. Cabooter, K. Broeckhoven, R. Sterken, A. Vanmessen, I. Vandendael, K. Nakanishi, S. Deridder, and G. Desmet, J. Chromatogr. A 1325, 72–82 (2014).

(56) S. Deridder, S. Eeltink, and G. Desmet, J. Sep. Sci. 34, 2038–2046 (2011).

(57) R.E. Majors, LCGC North Am. 33, 886–887 (2015).

(58) T. Hara, G. Desmet, G.V. Baron, H. Minakuchi, and S. Eeltink, J. Chromatogr. A 1442, 42–52 (2016).

(59) S. Fekete and D. Guillarme, J. Chromatogr. A 1308, 104–113 (2013).

(60) B. He, N. Tait, and F.E. Regnier, Anal. Chem. 70, 3790–3797 (1998).

(61) F.E. Regnier, J. High Resolut. Chromatogr. 23, 19–26 (2000).

(62) W. De Malsche, H. Eghbali, D. Clicq, J. Vangelooven, H. Gardeniers, and G. Desmet, Anal. Chem. 79, 5915–5926 (2007).

(63) J. Op De Beeck, W. De Malsche, S.T. Tezcan, P. De Moor, C. Van Hoof, and G. Desmet, J. Chromatogr. A 1239, 35–48 (2012).

(64) J. Op De Beeck, M. Callewaert, H. Ottevaere, H. Gardeniers, G. Desmet, and W. De Malsche, Anal. Chem. 85, 5207–5212 (2013).

(65) J. Op De Beeck, M. Callewaert, H. Ottevaere, H. Gardeniers, G. Desmet, and W. De Malsche, J. Chromatogr. A 1367, 118–122 (2014).

(66) G. Desmet, M. Callewaert, H. Ottevaere, and W. De Malsche, Anal. Chem. 87, 7382–7388 (2015).

(67) M. Callewaert,J. Op De Beeck, K. Maeno, S. Sukas, H. Thienpont, H. Ottevaere, H. Gardeniers, G. Desmet, and W. De Malsche, Analyst 139, 618–625 (2014).

(68) M. Isokawa, K. Takatsuki, Y.T. Song, K. Shih, K. Nakanishi, Z.M. Xie, D.H. Yoon, T. Sekiguchi, T. Funatsu, S. Shoji, and M. Tsunoda, Anal. Chem. 88, 6485–6491 (2016).

(69) W. De Malsche, D. Clicq, V. Verdoold, P. Gzil, G. Desmet, and H. Gardeniers, Lab Chip 7, 1705–1711 (2007).

(70) W. De Malsche, L. Zhang, J. Op De Beeck, J. Vangelooven, B. Majeed, and G. Desmet, J. Sep. Sci. 33, 3613–3618 (2010).

(71) J. Op De Beeck, W. De Malsche, P. De Moor, and G. Desmet, J. Sep. Sci. 35, 1877–1883 (2012).

(72) W. De Malsche, S. De Bruyne, J. Op De Beeck, P. Sandra, H. Gardeniers, G. Desmet, and F. Lynen, J. Chromatogr. A 1230, 41–47 (2012).

(73) W. De Malsche, J. Op De Beeck, S. De Bruyne, H. Gardeniers, and G. Desmet, Anal. Chem. 84, 1214–1219 (2012).

(74) S. Waheed, J.M. Cabot, N.P. Macdonald, T. Lewis, R.M. Guijt, B. Paull, and M.C. Breadmore, Lab Chip 16, 1993–2013 (2016).

(75) S. Sandron, B. Heery, V. Gupta, D.A. Collins, E.P. Nesterenko, P.N. Nesterenko, M. Talebi, S. Beirne, F. Thompson, G.G. Wallace, D. Brabazon, F. Regan, and B. Paull, Analyst 139, 6343–6347 (2014).

(76) C. Fee, S. Nawada, S.D. Dimartino, J. Chromatogr. A. 1333, 18–24 (2014).

(77) S. Nawada, S. Dimartino, and C. Fee, Chem. Eng. Sci. 164, 90–98 (2017).

(78) M. Emons, K. Obata, T. Binhammer, A. Ovsianikov, B.N. Chichkov, and U. Morgner, Opt. Mater. Express 2, 942 (2012).

K. Broeckhoven, S. Eeltink, W. De Malsche, F. Matheuse, and G. Desmet are with the Department of Chemical Engineering at the Vrije Universiteit Brussel, in Brussels, Belgium. D. Cabooter is with the Department of Pharmaceutical and Pharmacological Sciences at the University of Leuven (KU Leuven), in Leuven, Belgium. Direct correspondence to: ken.broeckhoven@vub.be